A boat floats in a swimming pool. In the boat is a block of ice. If the block is dumped into the water and melts, does the water level rise or fall?
Puzzles
The Broken Deck
When eight certain cards are removed from a standard poker deck, it becomes impossible to deal a straight flush. What are the cards? (Assume the deck contains no jokers.)
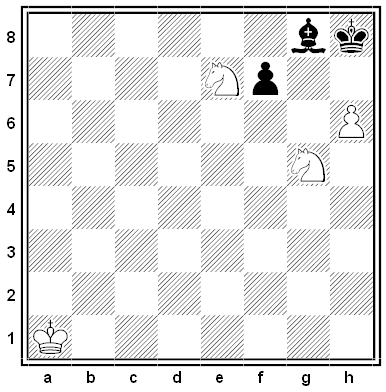
Black and White
Bootstrap Percolation

On a 12 × 12 grid, some squares are infected and some are healthy. On each turn, a healthy square becomes infected if it has two or more infected orthogonal neighbors. (In the example above, the black squares are infected, the white squares are healthy, and the gray squares will be infected next turn.) What’s the smallest number of initially infected squares that can spread an infection over the whole board?
Counterfeit Redux
A more challenging version of the Counterfeit Coin puzzle from 2011:
You have 12 coins, one of which has been replaced with a counterfeit. The false coin differs in weight from the true ones, but you don’t know whether it’s heavier or lighter. How can find it using three weighings in a pan balance?
“A Financial Puzzle”
This, now, is straightforward and business-like: A. applied to B. for a loan of $100. B. replied, ‘My dear A., nothing would please me more than to oblige you, and I’ll do it. I haven’t $100 by me, but make a note and I’ll indorse it, and you can get the money from the bank.’ A. proceeded to write the note. ‘Stay,’ said B., ‘make it $200. I want $100 myself.’ A. did so, B. indorsed the paper, the bank discounted it, and the money was divided. When the note became due, B. was in California, and A. had to meet the payment. What he is unable to cipher out is whether he borrowed $100 of B., or B. borrowed $100 of him.
— Henry C. Percy, Our Cashier’s Scrap-Book, 1879
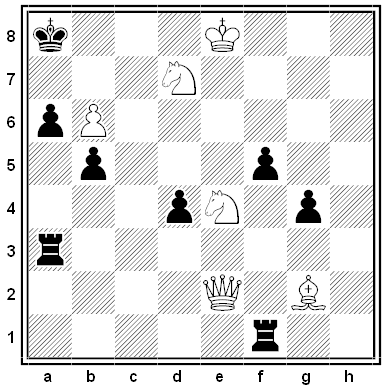
Black and White
Caller ID
In his 1936 collection Brush Up Your Wits, British puzzle maven Hubert Phillips relates that his brother-in-law felt himself cursed with an unintelligent maid. “I have just overheard her taking a ‘phone call,” he told Phillips, “and this is what I heard:
“‘Is Mr. Smith at home?’
“‘I will ask him, sir. What name shall I give him?’
“‘Quoit.’
“‘What’s that, sir?’
“‘Quoit.’
“‘Would you mind spelling it?’
“‘Q for quagga, U for umbrella, O for omnibus, I for idiot –‘
“‘I for what, sir?’
“‘I for idiot, T for telephone. Q, U, O, I, T, Quoit.’
“‘Thank you, sir.'”
Why did he accuse her of unintelligence?
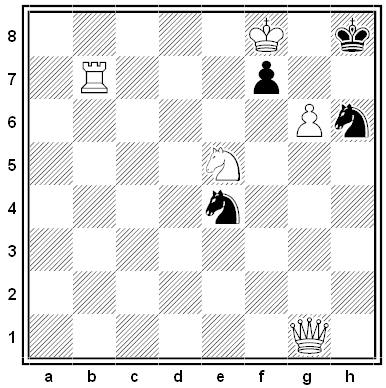
Brood War
A newlywed couple are planning their family. They’d like to have four children, a mix of girls and boys. Which is more likely: (1) two girls and two boys or (2) three children of one sex and one of the other? (Assume that each birth has an equal chance of being a boy or a girl.)