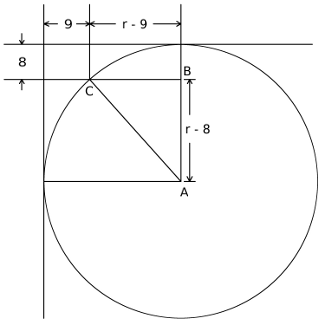
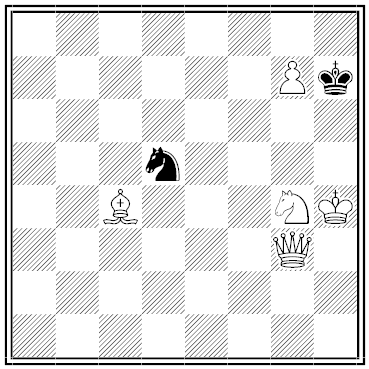
A circular table stands in a corner, touching both walls. A certain point on the table’s edge is 9 inches from one wall and 8 inches from the other. What’s the diameter of the table?
Puzzles
Going Down
In antiquity Aristotle had taught that a heavy weight falls faster than a light one. In 1638, without any experimentation, Galileo saw that this could not be true. What had he realized?
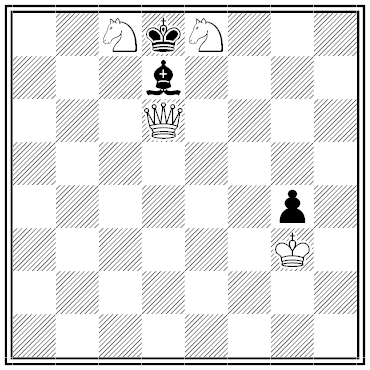
Black and White
Fish Story
A logic exercise by Lewis Carroll: What conclusion can be drawn from these premises?
- No shark ever doubts that it is well fitted out.
- A fish that cannot dance a minuet is contemptible.
- No fish is quite certain that it is well fitted out unless it has three rows of teeth.
- All fishes except sharks are kind to children.
- No heavy fish can dance a minuet.
- A fish with three rows of teeth is not to be despised.
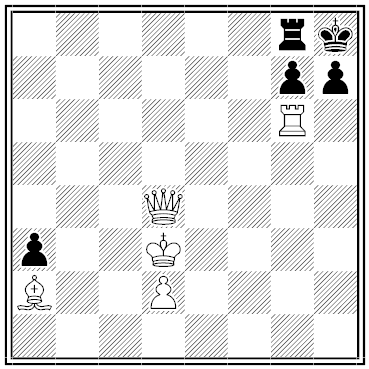
Black and White
Two for One
Longfellow thought that Dante Gabriel Rossetti, the Victorian poet and painter, was two different people. On leaving Rossetti’s house he said, “I have been very glad to meet you, Mr. Rossetti, and should like to have met your brother also. Pray tell him how much I admire his beautiful poem, ‘The Blessed Damozel.'”
In Philosophical Troubles, Saul A. Kripke offers a related puzzle. Peter believes that politicians never have musical talent. He knows of Paderewski, the great pianist and composer, and he has heard of Paderewski the Polish statesman, but he does not know that they are the same person. Does Peter believe that Paderewski had musical talent?
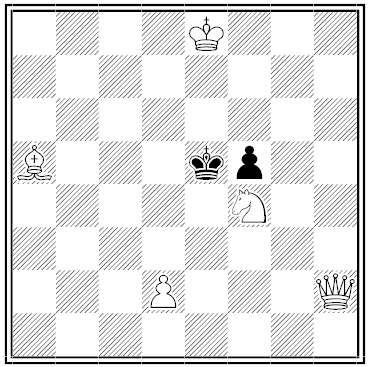
Black and White
Black and White
What Am I?
A riddle by Horatio Walpole:
Before my birth I had a name,
But soon as born I chang’d the same;
And when I’m laid within the tomb,
I shall my father’s name assume.
I change my name three days together
Yet live but one in any weather.
Dog Tired
Another puzzle by Boris Kordemsky: Jack London tells of racing from Skagway, Alaska, to a camp where a friend lay dying. London drove a sled pulled by five huskies, which pulled the sled at full speed for 24 hours. But then two dogs ran off with a pack of wolves. Left with three dogs and slowed down proportionally, London reached the camp 48 hours later than he had planned. If the two lost huskies had remained in harness for 50 more miles, he would have been only 24 hours late. How far is the camp from Skagway?