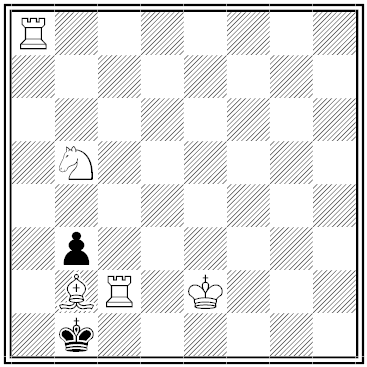
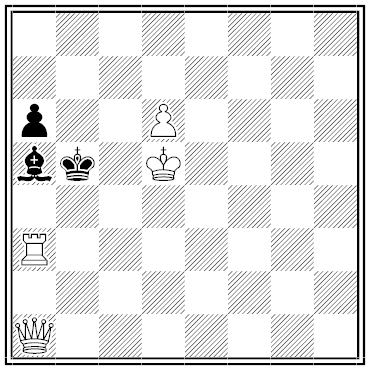
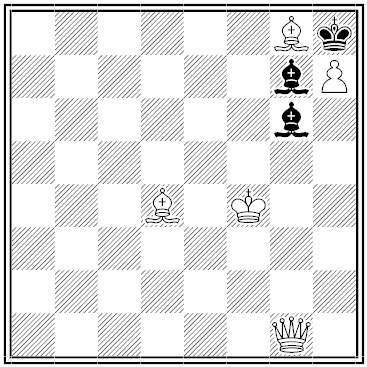
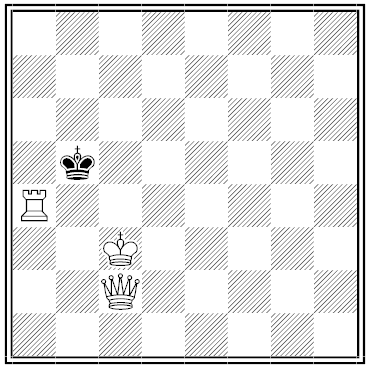
Puzzles
Going Up
A problem from the 2003 Moscow Mathematical Olympiad:
A store has three floors, which are connected only by an elevator. At night the store is empty, and during the workday:
(1) Of the customers who enter the elevator on the second floor, half go to the first floor and half to the third floor.
(2) The number of customers who get out the elevator on the third floor is less than 1/3 the total number of customers who get out of the elevator.
Which is greater, the number of customers who go from the first floor to the second on a given workday, or the number who go from the first floor to the third?
Too Late?
Paul R. McClenon of Washington D.C. contributed this problem to the January-February 1964 issue of Recreational Mathematics Magazine:
The poor patient read the prescription which would save his life. ‘Mix carefully a one-pint drink, made of scotch whisky and water, mixed one to five (1/6 scotch, 5/6 water). Drink it quickly and go to bed.’
However, the patient finds only the following items at hand:
A one-quart bottle, about half full of scotch whisky.
An eight-ounce glass.
An unlimited supply of water from his faucet.
A sink with a drain.
No other containers or measuring devices.He can pour from either container to the other, without spilling a drop, and can fill either to the brim without loss. How should he mix the prescription? Will he figure it out in time? Will he be saved? Did a doctor or bartender write this prescription?
The magazine went out of business before it could publish the solution. I’ll leave it to you.
05/17/2013 UPDATE: There seem to be a number of ways to accomplish this. Here’s one:
We need a 16-ounce dose that’s 1/6 whiskey, so the final mixture must contain 2.666 ounces of whiskey.
- Fill the 8-ounce glass with whiskey, then empty the jug.
- Return the glassful of whiskey to the empty jug and add two glassfuls of water.
- Fill the glass with this 2-to-1 mixture of water and whiskey. The 8-ounce glass now contains 2.666 ounces of whiskey, our target.
- Empty the bottle, pour the glass’ contents into it, and add one 8-ounce glassful of water.
That leaves us with 16 ounces in the jug, 1/6 of which is whiskey and the rest water, as directed.
Thanks to everyone who wrote in.
Black and White
“Illustrated Rebus”

From The Youth’s Companion, Sept. 25, 1879:
Why is this man likely to succeed in life?
Why do we know he has reached middle life?
How does the picture indicate his occupation?
Black and White
Quick!
If you choose an answer to this question at random, what’s the chance that you’ll be correct?
(a) 25% (b) 50% (c) 0% (d) 25%
Black and White
Name Sense
A 1936 poser by British puzzle maven Hubert Phillips:
A man I met in Fleet Street yesterday told me the following anecdote:
‘I met yesterday (he said) a friend of mine whom I had not seen since I was at Oxford. That was some years ago and we had not, during all that time, had any communication with one another. Nor had we at Oxford any friends or acquaintances in common.
‘I was delighted to see my friend, nevertheless. “I suppose,” I said, “that lots of things have happened to you.”
‘”Why, yes,” was the answer. “I am married now and this is my little girl.”
‘I looked at the child — a pretty little thing of about six. “And what is your name, my dear,” I asked her. “Margaret,” was the reply. “Aha,” said I, “the same name as your mother’s.”‘
How did the speaker know?