Puzzles
Well Done

Using a 7-quart and a 3-quart jug, how can you obtain exactly 5 quarts of water from a well?
That’s a water-fetching puzzle, a familiar task in puzzle books. Most such problems can be solved fairly easily using intuition or trial and error, but in Scripta Mathematica, March 1948, H.D. Grossman describes an ingenious way to generate a solution geometrically.
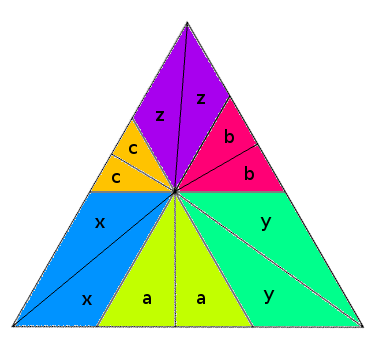
Let a and b be the sizes of the jugs, in quarts, and c be the number of quarts that we’re seeking. Here, a = 7, b = 3, and c = 5. (a and b must be positive integers, relatively prime, where a is greater than b and their sum is greater than c; otherwise the problem is unsolvable, trivial, or can be reduced to smaller integers.)
Using a field of lattice points (or an actual pegboard), let O be the point (0, 0) and P be the point (b, a) (here, 3, 7). Connect these with OP. Then draw a zigzag line Z to the right of OP, connecting lattice points and staying as close as possible to OP. Now “It may be proved that the horizontal distances from OP to the lattice-points on Z (except O and P) are in some order without repetition 1, 2, 3, …, a + b – 1, if we count each horizontal lattice-unit as the distance a.” In this example, if we take the distance between any two neighboring lattice points as 7, then each of the points on the zigzag line Z will be some unique integer distance horizontally from the diagonal line OP. Find the one whose distance is c (here, 5), the number of quarts that we want to retrieve.
Now we have a map showing how to conduct our pourings. Starting from O and following the zigzag line to C:
- Each horizontal unit means “Pour the contents of the a-quart jug, if any, into the b-quart jug; then fill the a-quart jug from the well.”
- Each vertical unit means “Fill the b-quart jug from the a-quart jug; then empty the b-quart jug.”
So, in our example, the map instructs us to:
- Fill the 7-quart jug.
- Fill the 3-quart jug twice from the 7-quart jug, each time emptying its contents into the well. This leaves 1 quart in the 7-quart jug.
- Pour this 1 quart into the 3-quart jug and fill the 7-quart jug again from the well.
- Fill the remainder of the 3-quart jug (2 quarts) from the 7-quart jug and empty the 3-quart jug. This leaves 5 quarts in the 7-quart jug, which was our goal.
You can find an alternate solution by drawing a second zigzag line to the left of OP. In reading this solution, we swap the roles of a and b given above, so the map tells us to fill the 3-quart jug three times successively and empty it each time into the 7-quart jug (leaving 2 quarts in the 3-quart jug the final time), then empty the 7-quart jug, transfer the remaining 2 quarts to it, and add a final 3 quarts. “There are always exactly two solutions which are in a sense complementary to each other.”
Grossman gives a rigorous algebraic solution in “A Generalization of the Water-Fetching Puzzle,” American Mathematical Monthly 47:6 (June-July 1940), 374-375.
Clatter Plot
A puzzle by Lewis Carroll:
Two travelers, starting at the same time, went opposite ways round a circular railway. Trains start each way every 15 minutes, the easterly ones going round in 3 hours, the westerly in 2. How many trains did each meet on the way, not counting trains met at the terminus itself?
Pandigital Primes
The digits 123456789 can be arranged to form 362,880 distinct 9-digit numbers.
How many of these are prime?
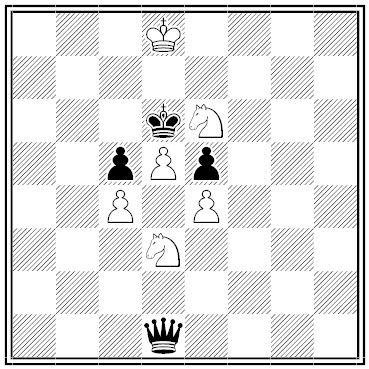
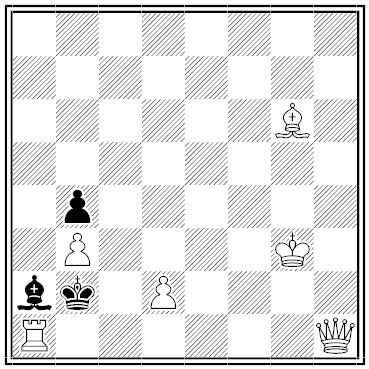
Black and White
Black and White
Round Numbers

Alan and Bob are playing a game of marbles. Alan has two marbles, Bob has one, and each rolls to try to come nearest to a fixed point. If the two have equal skill, what is the chance that Alan will win?
There seem to be two contradictory arguments. On the one hand, each of the three marbles has an equal chance of winning, and two of them belong to Alan, so it seems that there’s a 2/3 chance that Alan will win.
On the other hand, there are four possible outcomes: (a) both of Alan’s rolls are better than Bob’s, (b) Alan’s first roll is better than Bob’s, but his second is worse, (c) Alan’s first roll is worse than Bob’s, but his second is better, and (d) both of Alan’s rolls are worse than Bob’s. In 3 of the 4 cases, Alan wins, so it appears that his overall chance of winning is 3/4.
Which argument is correct?
More Is Less
A riddle attributed to British prime minister George Canning, among many others:
A word there is of plural number,
Foe to ease and tranquil slumber.
Any other word you take
And add an S will plural make;
But if you add an S to this,
So strange the metamorphosis,
Plural is plural now no more
And sweet what bitter was before.
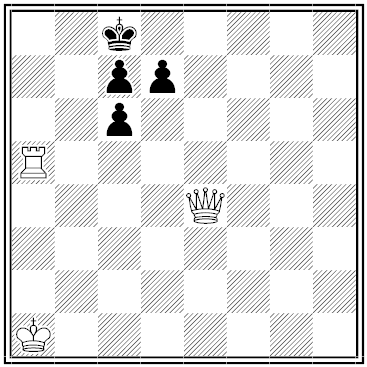
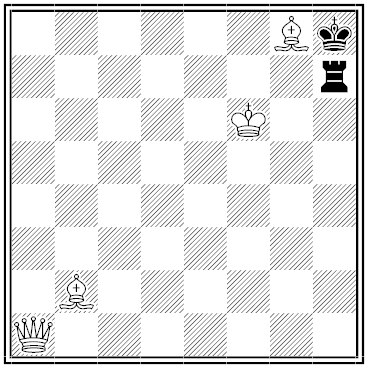
Black and White
Half and Half
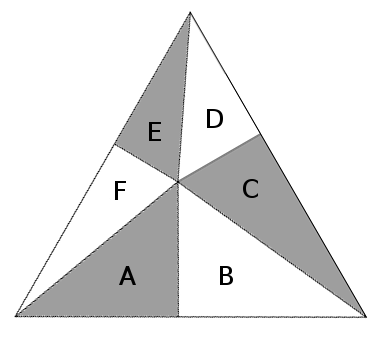
Choose an arbitrary point inside an equilateral triangle and draw segments to the vertices and perpendiculars to the sides. This divides the triangle into six smaller triangles, A, B, C, D, E, and F. Prove that the areas A + C + E and B + D + F are equal.