A puzzle by Henry Dudeney:
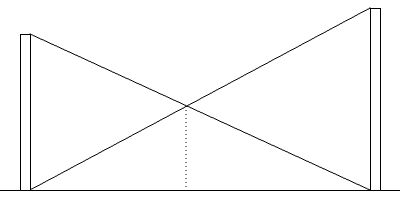
A man planted two poles upright in level ground. One pole was six and a half feet and the other seven feet seven inches above ground. From the top of each pole he tied a string to the bottom of the other — just where it entered the ground. Now, what height above the ground was the point where the two strings crossed one another? The hasty reader will perhaps say, “You have forgotten to tell us how far the poles were apart.” But that point is of no consequence whatever, as it does not affect the answer!