English meteorologist Lewis Fry Richardson (1881-1953) spent the last 25 years of his life trying to establish a mathematical theory of the causes of war. In the first of two books on this subject, Arms and Insecurity, he works out a model of arms races using differential equations and reaches the conclusion that
where:
U and V are the annual defense budgets of two parties to a conflict
k is a positive constant representing the response to threat
α is a positive constant representing the fatigue and expense of keeping up defenses
U0 and V0 represent cooperations between the parties, tentatively assumed to remain constant
and g and h represent the “grievances and ambitions, provisionally regarded as constant,” on each side.
The term in brackets is a constant, so Richardson predicted that plotting d(U + V)/dt against (U + V) would produce a straight line. He tried this out using the defense budgets of the Franco-Russian and Austro-German alliances for 1909-14 and got this:
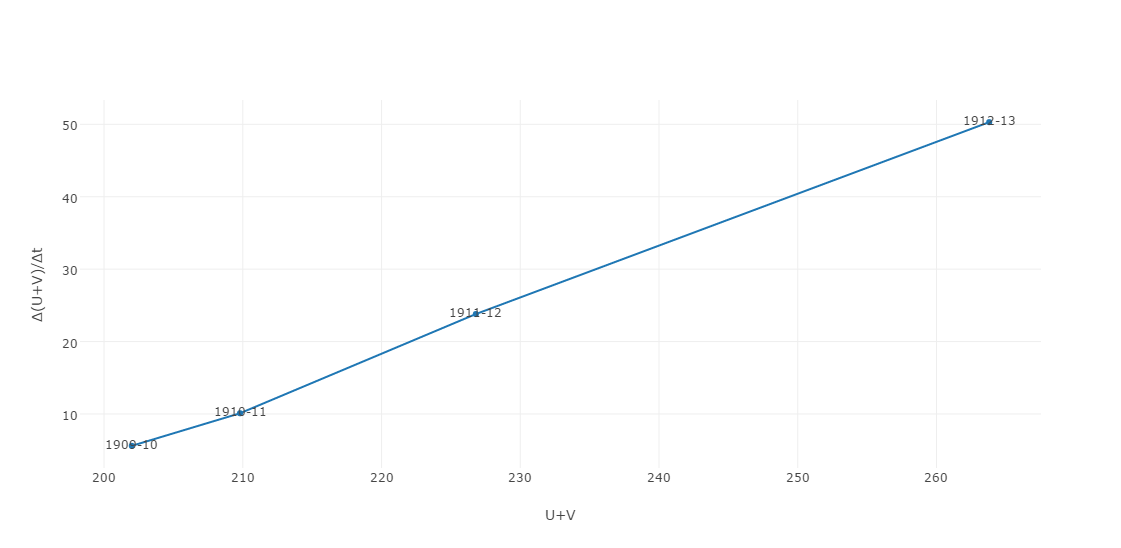
“The four points lie close to a straight line, closer, indeed, than one might expect,” he writes. “Since I first drew this diagram, which was shown at the British Association in Cambridge in 1938, and printed in Nature of 29 October of that year, I have been incredulous about the marvelously good fit. Yet there is no simple mistake. … The mere regularity of these phenomena shows that foreign politics had then a rather machine-like quality, intermediate between the predictability of the moon and the freedom of an unmarried young man.”
The extrapolated straight line hits the x axis at U + V = £194 pounds sterling. “As love covereth a multitude of sins, so the good will between the opposing alliances would just have covered £194 million of defense expenditures on the part of the four nations concerned. Their actual expenditure in 1909 was £199 millions; and so began an arms race which led to World War I.”
(Lewis F. Richardson, Arms and Insecurity, 1949.)