Nepal’s constitution contains complete instructions for drawing its flag:
(A) Method of Making the Shape Inside the Border
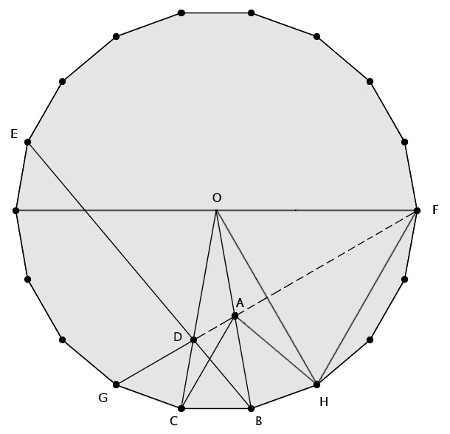
(1) On the lower portion of a crimson cloth draw a line AB of the required length from left to right.
(2) From A draw a line AC perpendicular to AB making AC equal to AB plus one third AB. From AC mark off D making line AD equal to line AB. Join BD.
(3) From BD mark off E making BE equal to AB.
(4) Touching E draw a line FG, starting from the point F on line AC, parallel to AB to the right hand-side. Mark off FG equal to AB.
(5) Join CG.(B) Method of Making the Moon
(6) From AB mark off AH making AH equal to one-fourth of line AB and starting from H draw a line HI parallel to line AC touching line CG at point I.
(7) Bisect CF at J and draw a line JK parallel to AB touching CG at point K.
(8) Let L be the point where lines JK and HI cut one another.
(9) Join JG.
(10) Let M be the point where line JG and HI cut one another.
(11) With centre M and with a distance shortest from M to BD mark off N on the lower portion of line HI.
(12) Touching M and starting from O, a point on AC, draw a line from left to right parallel to AB.
(13) With centre L and radius LN draw a semi-circle on the lower portion and let P and Q be the points where it touches the line OM respectively.
(14) With centre M and radius MQ draw a semi-circle on the lower portion touching P and Q.
(15) With centre N and radius NM draw an arc touching PNQ [sic] at R and S. Join RS. Let T be the point where RS and HI cut one another.
(16) With Centre T and radius TS draw a semi-circle on the upper portion of PNQ touching it at two points.
(17) With centre T and radius TM draw an arc on the upper portion of PNQ touching at two points.
(18) Eight equal and similar triangles of the moon are to be made in the space lying inside the semi-circle of No. (16) and outside the arc of No. (17) of this Schedule.(C) Method of Making the Sun
(19) Bisect line AF at U and draw a line UV parallel to line AB touching line BE at V.
(20) With centre W, the point where HI and UV cut one another and radius MN draw a circle.
(21) With centre W and radius LN draw a circle
(22) Twelve equal and similar triangles of the sun are to be made in the space enclosed by the circles of No. (20) and of No. (21) with the two apexes of two triangles touching line HI.(D) Method of Making the Border
(23) The width of the border will be equal to the width TN. This will be of deep blue colour and will be provided on all the sides of the flag. However, on the five angles of the flag the external angles will be equal to the internal angles.
(24) The above mentioned border will be provided if the flag is to be used with a rope. On the other hand, if it is to be hoisted on a pole, the hole on the border on the side AC can be extended according to requirements.Explanation: The lines HI, RS, FE, ED, JG, OQ, JK and UV are imaginary. Similarly, the external and internal circles of the sun and the other arcs except the crescent moon are also imaginary. These are not shown on the flag.
That’s a good thing — it’s the only national flag that’s not a quadrilateral. The two pennants represent different branches of a ruling dynasty in the 19th century. The nation signaled its pride in the new design last February by setting a world record for the largest human flag — 35,000 Nepalese gathered in Kathmandu to break Pakistan’s record and to demonstrate their own national unity. I wonder how they worked out the geometry: