“A science is any discipline in which the fool of this generation can go beyond the point reached by the genius of the last generation.” — Max Gluckman
Science & Math
Two in One

Look at this image closely and you’ll see the features of Albert Einstein.
But look at it from across a room and you’ll see Marilyn Monroe.
It’s a “hybrid image,” created using a technique developed by Aude Oliva of MIT and Philippe Schyns of the University of Glasgow. The image combines the low spatial frequencies of one picture with the high spatial frequencies of another, so that it’s processed differently at different viewing distances.
See their paper for the details, and this gallery for more examples.
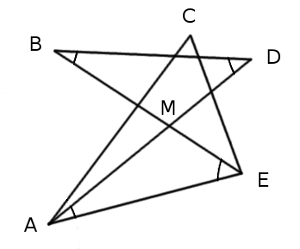
Star Power
A puzzle by A. Korshkov, from the Russian science magazine Kvant:
It’s easy to show that the five acute angles in the points of a regular star, like the one at left, total 180°.
Can you show that the sum of these angles in an irregular star, like the one at right, is also 180°?
Misc

- The clock face on the Marienkirche in Bergen auf Rügen, Germany, has 61 minutes. Does this mean time moves more slowly there — or more quickly?
- To ensure quiet, poet Amy Lowell hired five rooms at every hotel — her own and those on either side, above, and below.
- A perplexing sentence from a letter by Dorothy Osborne, describing shepherdesses in Bedfordshire, May 1653: “They want nothing to make them the happiest people in the world but the knowledge that they are so.”
- OVEREFFUSIVE is a palindrome in Scrabble — its letter values are 141114411141. (Discovered by Susan Thorpe.)
- The sum of the digits of every multiple of 2739726 up to the 72nd is 36. (E.M. Langley, Mathematical Gazette, 1896)
- I’ll bet I have more money in my pocket than you do. (Of course I do — you have no money in my pocket!)
- In 1996 a model airplane enthusiast was operating a remote-controlled plane in Phoenix Park in Dublin when the receiver died and the plane flew off on its own. It flew five miles to the northeast, ran out of fuel, and glided to a landing … on the taxi-way to Runway 28 at Dublin Airport.
(Thanks, Brian and Breffni.)
Say Red
Cornell mathematician Robert Connelly devised this intuition-defying card game. I shuffle a standard deck of 52 cards and deal them out in a row before you, one at a time. At some point before the last card is dealt, you must say the word “red.” If the next card I deal is red, you win $1; if it’s black you lose $1. If you play blind, your chance of winning is 1/2. Can you improve on this by devising a strategy that considers the dealt cards?
Surprisingly, the answer is no. Imagine a deck with two red cards and two black. Now there are six equally likely deals:
RRBB
RBBR
BBRR
RBRB
BRBR
BRRB
By counting, we can see that the chance of success remains 1/2 regardless of whether you call red before the first, second, third, or fourth card.
Trying to outsmart the cards doesn’t help. You might resolve to wait and see the first card: If it’s black you’ll call red immediately, and if it’s red you’ll wait until the fourth card. It’s true that this strategy gives you a 2/3 chance of winning if the first card is black — but if it’s red then it has a 2/3 chance of losing.
Similarly, it would seem that if the first two cards are black then you have a sure thing — the next card must be red. This is true, but it will happen only once in six deals; on the other five deals, calling red at the third card wins only 2/5 of the time — so this strategy has an overall success rate of (1/6 × 1) + (5/6 × 2/5) = 1/2, just like the others. The cards conspire to erase every seeming advantage.
The same principle holds for a 52-card deck, or indeed for any deck. In general, if a deck has r red cards and b black ones, then your chance of winning, by any strategy whatsoever, is r/(b + r). Seeing the cards that have already been dealt, surprisingly, is no advantage.
(Robert Connelly, “Say Red,” Pallbearers Review 9 [1974], 702.)
Six by Six
The sestina is an unusual form of poetry: Each of its six stanzas uses the same six line-ending words, rotated according to a set pattern:

This intriguingly insistent form has appealed to verse writers since the 12th century. “In a good sestina the poet has six words, six images, six ideas so urgently in his mind that he cannot get away from them,” wrote John Frederick Nims. “He wants to test them in all possible combinations and come to a conclusion about their relationship.”
But the pattern of permutation also intrigues mathematicians. “It is a mathematical property of any permutation of 1, 2, 3, 4, 5, 6 that when it is repeatedly combined with itself, all of the numbers will return to their original positions after six or fewer iterations,” writes Robert Tubbs in Mathematics in Twentieth-Century Literature and Art. “The question is, are there other permutations of 1, 2, 3, 4, 5, 6 that have the property that after six iterations, and not before, all of the numbers will be back in their original positions? The answer is that there are many — there are 120 such permutations. We will probably never know the aesthetic reason poets settled on the above permutation to structure the classical sestina.”
In 1986 the members of the French experimental writers’ workshop Oulipo began to apply group theory to plumb the possibilities of the form, and in 2007 Pacific University mathematician Caleb Emmons offered the ultimate hat trick: A mathematical proof about sestinas written as a sestina:

Bonus: When not doing math and poetry, Emmons runs the Journal of Universal Rejection, which promises to reject every paper it receives: “Reprobatio certa, hora incerta.”
(Caleb Emmons, “S|{e,s,t,i,n,a}|“, The Mathematical Intelligencer, December 2007.) (Thanks, Robert and Kat.)
Overheard

A puzzle by Princeton mathematician John Horton Conway:
Last night I sat behind two wizards on a bus, and overheard the following:
A: I have a positive integral number of children, whose ages are positive integers, the sum of which is the number of this bus, while the product is my own age.
B: How interesting! Perhaps if you told me your age and the number of your children, I could work out their individual ages?
A: No.
B: Aha! AT LAST I know how old you are!
“This is an incredible puzzle,” writes MIT research affiliate Tanya Khovanova. “This is also an underappreciated puzzle. It is more interesting than it might seem. When someone announces the answer, it is not clear whether they have solved it completely.”
We can start by auditioning various bus numbers. For example, the number of the bus cannot have been 5, because in each possible case the wizard’s age and the number of his children would then uniquely determine their ages — if the wizard is 3 years old and has 3 children, then their ages must be 1, 1, and 3 and he cannot have said “No.” So the bus number cannot be 5.
As we work our way into higher bus numbers this uniqueness disappears, but it’s replaced by another problem — the second wizard must be able to deduce the first wizard’s age despite the ambiguity. For example, if the bus number is 21 and the first wizard tells us that he’s 96 years old and has three children, then it’s true that we can’t work out the children’s ages: They might be 1, 8, and 12 or 2, 3, and 16. But when the wizard informs us of this, we can’t declare triumphantly that at last we know how old he is, because we don’t — he might be 96, but he might also be 240, with children aged 4, 5, and 12 or 3, 8, and 10. So the dialogue above cannot have taken place.
But notice that if we increase the bus number by 1, to 22, then all the math above will still work if we give the wizard an extra 1-year-old child: He might now be 96 years old with four children ages 1, 1, 8, and 12 or 1, 2, 3, and 16; or he might be 240 with four children ages 1, 4, 5, and 12 or 1, 3, 8, and 10. The number of children increases by 1, the sum of their ages increases by 1, and the product remains the same. So if bus number b produces two possible ages for Wizard A, then so will bus number b + 1 — which means that we don’t have to check any bus numbers larger than 21.
This limits the problem to a manageable size, and it turns out that the bus number is 12 and Wizard A is 48 — that’s the only age for which the bus number and the number of children do not uniquely determine the children’s ages (they might be 2, 2, 2, and 6 or 1, 3, 4, and 4).
(Tanya Khovanova, “Conway’s Wizards,” The Mathematical Intelligencer, December 2013.)
Two similar puzzles: A Curious Conversation and A Curious Exchange.
Sweet Science
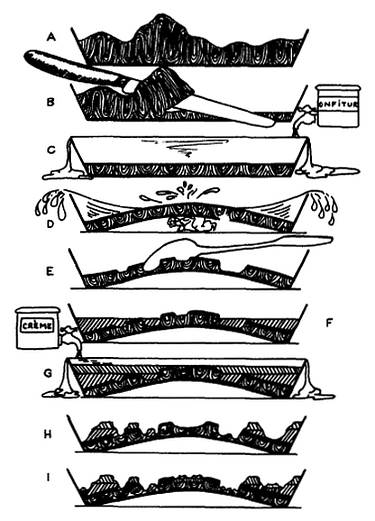
Sahara geology presented as a flan recipe, from French naturalist Théodore Monod’s Méharées: Explorations au vrai Sahara, 1937:
Take a flan-tray, which represents the basement (our Mauretanian and Tuareg granites).
- Place some pastry in the flan-tray in irregular masses (A) — these are the Precambrian mountain chains, the Saharides.
- Level this off with a knife (B) so that the folds, as in erosional peneplanation of the Sahara, are seen only in the ravines which cross the plain; the mountains are now vigorously planed down.
- First event: a tap (from which, fortunately, jam flows) floods the garnished mould (C). Similarly the sea at the beginning of the Palaeozoic invaded the Saharan basement, which it then partly occupied, until the middle Carboniferous — what an enormous amount of jam! All this time the Sahara is under water, and sandstones, limestones, conglomerates and shales were deposited — all the sediments of the Tuareg and Mauritanian plateaux.
- A new event (the djinns must have been at work here) — the bottom of the flan-tray experiences an uplift; the dish, pastry and jam emerge (D). This is the time of the coal measures; the sea retreats, and the Sahara is left high and dry, basking in the sun.
- But whoever says dry land, implies erosion; the sediments rise up, are corroded, and the spoon cuts so deeply that it exposes the jam, pastry, and sometimes even the metal of the flan-tray (E).
- And while this continues for millions of years, erosion is unable to evaporate its own debris and the eroded sediments are not washed away to the sea — they just accumulate, and what is lost in some districts is gained by others, whilst gradual infilling continues (F).
- Then one fine day, while iguanodons are blundering around in Picardy, and swarms of ammonites are scudding around in the Parisian sea, a second tap is turned on again and adds another layer, this time of cream (for convenience of explanation) (G). The sea re-invades a good part of the Sahara and deposits the usual sediments — Cretaceous and Eocene.
- A new retreat of the sea and a new continental phase occur, with customary erosion and deposition (H).
- Gradually, the country comes to be like it is today; sprinkle with granular sugar (fresh-water Quaternary deposits), and icing sugar dunes (I).
- And there we are! Serve hot or chilled.
“Very well — that will teach me to invent foolish nonsense for my neophyte when it is so easy to explain the influence of Saharidian tectonics on the orientation of Hercynian virgations, the suggestion of angular discordnce separating the basal congomerate of the continental beds from the post-Visean argillites, or more simply the origin of the bowlingite included in the pigeonite andesite with diabase facies of Telig. But I doubt that he would understand it any better …”
The Paradox of Goals

Suppose that two teams of equal ability are playing football. If goals are scored at regular intervals, it seems natural to expect that each team will be in the lead for half the playing time. Surprisingly, this isn’t so: If a total of n = 20 goals are scored, then the probability that Team A leads after the first 10 goals and Team B leads after the second 10 goals is only 6 percent, while the probability that one team leads throughout the entire game is about 35 percent. (When the scores are equal, the leading team is considered to be the one that was leading before the last goal.) And the chance that one team leads throughout the second half is 50 percent, no matter how large n is.
Such questions began with a study of ballot problems: In 1887 Joseph Bertrand found that if in an election Candidate P scores p votes and Candidate Q scores q votes, where p > q, then the probability that P leads throughout the voting is (p – q)/(p + q).
But pursuing them has led to “conclusions that play havoc with our intuition,” writes Princeton mathematician William Feller. If Peter and Paul toss a coin 20,000 times, we tend to think that each will lead about half the time. But in fact it is 88 times more probable that Peter leads in all 20,000 trials than that each player leads in 10,000 trials. No matter how long the series of coin tosses runs, the most probable number of changes of lead is zero.
“In short, if a modern educator or psychologist were to describe the long-run case histories of individual coin-tossing games, he would classify the majority of coins as maladjusted,” Feller writes. “If many coins are tossed n times each, a surprisingly large proportion of them will leave one player in the lead almost all the time; and in very few cases will the lead change sides and fluctuate in the manner that is generally expected of a well-behaved coin.”
(Gábor J. Székely, Paradoxes in Probability Theory and Mathematical Statistics, 2001; William Feller, An Introduction to Probability Theory and Its Applications, 1957.)
Curves of Constant Width
Trap a circle inside a square and it can turn happily in its prison — a circle has the same breadth in any orientation.
Perhaps surprisingly, circles are not the only shapes with this property. The Reuleaux triangle has the same width in any orientation, so it can perform the same trick:
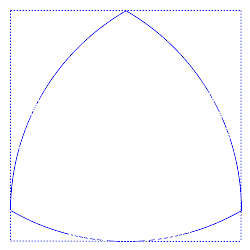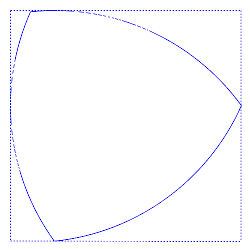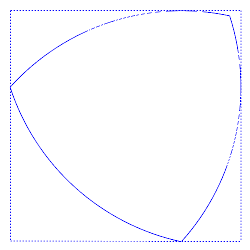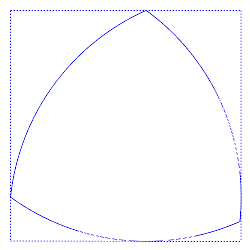
In fact any square can accommodate a whole range of “curves of constant width,” all of which have the same perimeter (πd, like the circle). Some of these are surprisingly familiar: The heptagonal British 20p and 50p coins and the 11-sided Canadian dollar coin have constant widths so that vending machines can recognize them. What other applications are possible? In the June 2014 issue of the Mathematical Intelligencer, Monash University mathematician Burkard Polster notes that a curve of constant width can produce a bit that drills square holes:
… and a unicycle with bewitching wheels:
The self-accommodating nature of such shapes permits them to take part in fascinating “dances,” such as this one among seven triangles:
This inspired Kenichi Miura to propose a water wheel whose buckets are Reuleaux triangles. As the wheel turns, each pair of adjacent buckets touch at a single point, so that no water is lost:
Here’s an immediately practical application: Retired Chinese military officer Guan Baihua has designed a bicycle with non-circular wheels of constant width — the rider’s weight rests on top of the wheels and the suspension accommodates the shifting axles:
(Burkard Polster, “Kenichi Miura’s Water Wheel, or the Dance of the Shapes of Constant Width,” Mathematical Intelligencer, June 2014.)