Theorem 1. A crocodile is longer than it is wide.
Proof. A crocodile is long on the top and bottom, but it is green only on the top; therefore a crocodile is longer than it is green. A crocodile is green along both its length and width, but it is wide only along its width; hence a crocodile is greener than it is wide. Therefore a crocodile is longer than it is wide.
Theorem 2. Napoleon was a poor general.
Proof. Most men have an even number of arms. Napoleon was warned that Wellington would meet him at Waterloo. To be forewarned is to be forearmed. But four arms is a very odd number of arms for a man. The only number that is both even and odd is infinity. Therefore, Napoleon had an infinite number of arms in his battle against Wellington. A general who loses a battle despite having an infinite number of arms is very poor general.
Theorem 3. If 1/0 = ∞, then 1/∞ = 0.
Proof. Given:
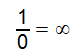
Rotate both sides 90° counterclockwise:
![]()
Subtract 8 from both sides:
![]()
Now reverse the rotation:
![]()