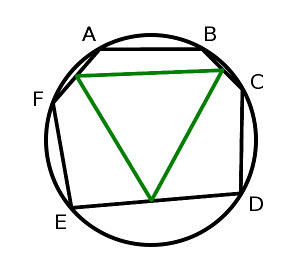
Inscribe a hexagon in a unit circle such that AB = CD = EF = 1.
Now the midpoints of BC, DE, and FA form an equilateral triangle.
See A Better Nature.

Inscribe a hexagon in a unit circle such that AB = CD = EF = 1.
Now the midpoints of BC, DE, and FA form an equilateral triangle.
See A Better Nature.

Arthur W.J.G. Ord-Hume calls this “the most graceful and simple perpetual motion machine of all time.” It was offered by American inventor F.G. Woodward in the 19th century. A heavy wheel is mounted between two rollers so that the wheel’s weight causes it to roll continuously in the direction of the arrow.
Or so Woodward hoped. Ord-Hume notes that the principle required the left half of the wheel always to be heavier than the right half. “Sadly, it wasn’t.”
How do I know that I’m not just a fictional character in some imagined story? What could I learn about myself that would prove that I’m real? “I am human, male, brunette, etc., but none of that helps,” writes UCLA philosopher Terence Parsons. “I see people, talk to them, etc., but so did Sherlock Holmes.”
Descartes would say that the very fact that I’m thinking about this shows that I exist: cogito ergo sum. But a fictional character could make the same argument. “Hamlet did think a great many things,” writes Jaakko Hintikka. “Does it follow that he existed?” Robert Nozick adds, “Could not any proof be written into a work of fiction and be presented by one of the characters, perhaps one named ‘Descartes’?”
Tweedledee tells Alice that she’s only a figment of the Red King’s dream. “If that there King was to wake,” adds Tweedledum, “you’d go out — bang! — just like a candle!”
Alice says, “Hush! You’ll be waking him, I’m afraid, if you make so much noise.”
“Well, it’s no use YOUR talking about waking him,” replies Tweedledum, “when you’re only one of the things in his dream. You know very well you’re not real.”
“It seems to me that this is a philosophical problem that deserves to be treated seriously on a par with issues like the reality of the external world and the existence of other minds,” Parsons writes. “I don’t know how to solve it.”
(Terence Parsons, Nonexistent Objects, 1980; Charles Crittenden, Unreality, 1991; Robert Nozick, “Fiction,” Ploughshares 6:3 [1980], 74-78; Jaakko Hintikka, “Cogito, Ergo Sum: Inference or Performance?”, Philosophical Review 71:1 [January 1962], 3-32.)

If a flock of birds disperses gradually, at what point does it cease to be a flock?
“There is at the moment a pipe on my desk,” wrote MIT philosopher Richard Cartwright in 1987. “Its stem has been removed, but it remains a pipe for all that; otherwise no pipe could survive a thorough cleaning.”
But he also owned a two-volume set of John McTaggart’s The Nature of Existence, one volume of which was in Cambridge and the other in Boston. Do those two volumes still make one thing? If so, is there a “thing” composed of the Eiffel Tower and the Old North Church? Why not?
(From Cartwright’s Philosophical Essays.)
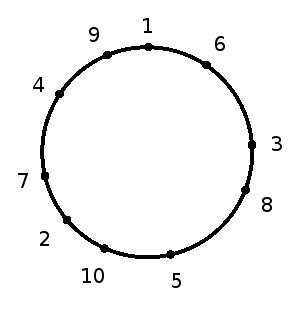
Draw a circle whose circumference is the golden mean. Choose a point and label it 1, then move clockwise around the circle in steps of arc length 1, labeling the points 2, 3, and so on. At each step, the difference between each pair of adjacent numbers on the circle is a Fibonacci number.
When Montenegro declared independence from Yugoslavia, its top-level domain changed from .yu to .me.
If I buy two toothbrushes in a “buy one, get one free” offer … which one did I buy, and which was free?
(From philosopher Roy Sorensen.)
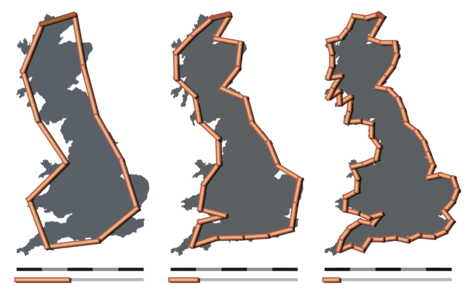
How long is a coastline? If we measure with a long yardstick, we get one answer, but as we shorten the scale the total length goes up. For certain mathematical shapes, indeed, it goes up without limit.
English mathematician Lewis Fry Richardson discovered this perplexing result in the early 20th century while examining the relationship between the lengths of national boundaries and the likelihood of war. If the Spanish claim that the length of their border with Portugal is 987 km, and the Portuguese say it’s 1,214 km, who’s right? The ambiguity arises because a wiggly boundary occupies a fractional dimension — it’s something between a line and a surface.
“At one extreme, D = 1.00 for a frontier that looks straight on the map,” Richardson wrote. “For the other extreme, the west coast of Britain was selected because it looks like one of the most irregular in the world; it was found to give D = 1.25.”
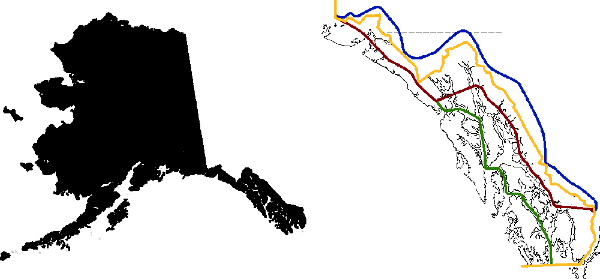
This is a mathematical notion, but it’s also a practical problem. On the fjord-addled panhandle of Alaska, the boundary with British Columbia was originally defined as “formed by a line parallel to the winding of the coast.” Who gets to define that? On the map below, the United States claimed the blue border, Canada wanted the red one, and British Columbia claimed the green. The yellow border was arbitrated in 1903.
Write out the positive powers of 10 in both base 2 and base 5:
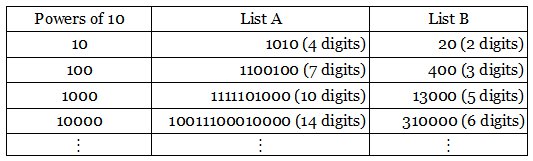
Now for any integer n > 1, we’ll find exactly one number of length n somewhere on the two lists. They contain one 3-digit number, one 4-digit number, and so on forever — if n = 100 we find a 100-digit number in the 30th position on the base 2 list.
(This result first appeared in the 1994 Asian Pacific Mathematics Olympiad. I found it in Ravi Vakil’s A Mathematical Mosaic.)
Two further curious lists: If we write out the triangular numbers, those in positions 3, 33, etc. show a pattern:
T(3) = 6
T(33) = 561
T(333) = 55611
T(3,333) = 5556111
T(33,333) = 555561111
T(333,333) = 55555611111
Similarly:
T(6) = 21
T(66) = 2211
T(666) = 222111
T(6,666) = 22221111
T(66,666) = 2222211111
T(666,666) = 222222111111
(Thanks, Larry.)
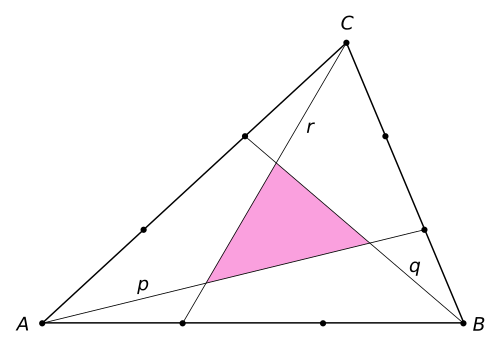
Draw any triangle and divide each leg into three equal segments. Connect each vertex to one of the trisection points on the opposite leg, as shown, and the triangle formed in the center will have 1/7 the area of the original triangle.
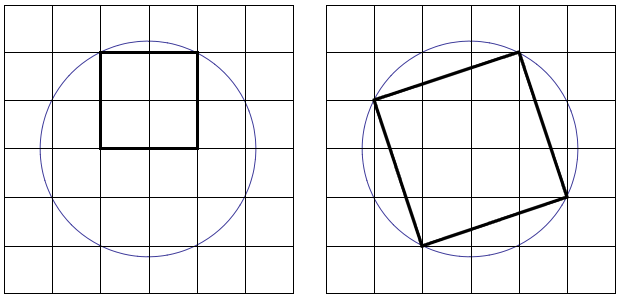
A square inscribed in a semicircle has 2/5 the area of a square inscribed in a circle of the same radius.
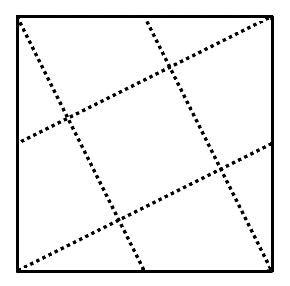
Draw a square and connect each vertex to the midpoint of an opposite side, as shown. The square formed in the center will have 1/5 the area of the original square.
A “proof without words”:
Trisect each side of a triangle and join each vertex to the opposite trisection points. Then write a hexagram in the hexagon in the center. The area of the hexagram is 7/100 the area of the original triangle.