Steven Bartlett and Peter Suber’s Self-Reference: Reflections on Reflexivity contains a bibliography of works on reflexivity.
It includes an entry for Steven Bartlett and Peter Suber’s Self-Reference: Reflections on Reflexivity.
Steven Bartlett and Peter Suber’s Self-Reference: Reflections on Reflexivity contains a bibliography of works on reflexivity.
It includes an entry for Steven Bartlett and Peter Suber’s Self-Reference: Reflections on Reflexivity.

Until 1911, the U.S. House of Representatives grew along with the country. Accordingly, when the 1880 census showed an increase in population, C.W. Seaton, chief clerk of the census office, worked out apportionments for all House sizes between 275 and 350, in order to see which states would get the new seats.
He was in for a surprise. The method was straightforward: Take the total U.S. population and divide it by the proposed number of seats in the House, rounding all fractions down. This would dispose of most of the seats; any leftover seats would be awarded to the states whose fractional remainders had been highest. But Seaton discovered an oddity:

If the House had 299 seats, Alabama would get 8 representatives (because its remainder, .646, was higher than that of Texas or Illinois). But if the House had 300 seats it would get only 7 (the extra representative would now go to Illinois, whose remainder had surpassed Alabama’s). The problem is that the “fair share” of a large state increases more quickly than that of a small state.
Seaton called this the Alabama paradox. A related problem is the population paradox: If the method above had been used in 1901 to reallocate 386 seats in the House, Virginia would have lost a seat to Maine even though the ratio of their populations had increased from 2.67 to 2.68:

Here, even though the size of the House has not changed, a fast-growing state receives fewer representatives than a slow-growing one.
In 1982 mathematicians Michel Balinski and Peyton Young showed that if each party gets one of the two numbers closest to its fair share of seats, then any system of apportionment will run into one of these paradoxes. The solution, it seems clear, is to start cutting legislators into pieces.
(These data are from Hannu Nurmi’s Voting Paradoxes and How to Deal With Them, 1999. Balinski and Young’s book is Fair Representation: Meeting the Ideal of One Man, One Vote.)
In 1981, when science journalist Marcus Chown was an undergraduate physics student, his mother watched a profile of Richard Feynman on the BBC series Horizon. She had never shown an interest in science before, and he wanted to encourage her, so when he advanced to Caltech to study astrophysics, he told Feynman of his mother’s interest and asked him to send her a birthday note. She received this:
Happy Birthday Mrs. Chown!
Tell your son to stop trying to fill your head with science — for to fill your heart with love is enough!
Richard P. Feynman (the man you watched on BBC “Horizons”)
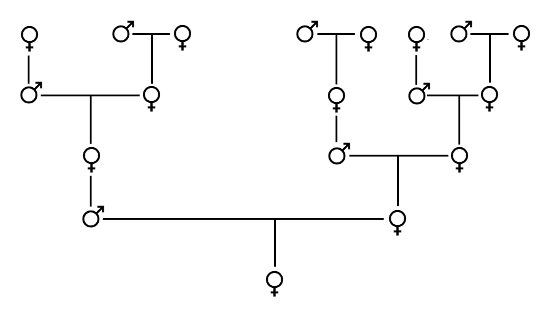
Male bees come from unfertilized eggs, so they have mothers but no fathers. Females come from fertilized eggs, so they have parents of both sexes. This produces an interesting pattern: The number of males in a given generation equals the number of females in the succeeding generation. And the number of females in a given generation equals the number of females in the succeeding two generations:

So the total number of bees, male and female, in generation n is the Fibonacci number Fn.
W. Hope-Jones discovered the relationship in 1921; this example is from Thomas Koshy’s Fibonacci and Lucas Numbers With Applications, 2001.
The first 10 digits of the golden ratio φ can be rearranged to give the first 10 digits of 1/π:
φ = 1.618033988 …
1/π = .3183098861 …
And the first nine digits of 1/φ can be rearranged to give the first 9 digits of 1/π:
1/φ = .618033988 …
1/π = .318309886 …

In 1983 amateur mathematician George Odom discovered that if points A and B are the midpoints of sides EF and DE of an equilateral triangle, and line AB meets the circumscribing circle at C, then AB/BC = AC/AB = φ. Odom used this fact to construct a pentagon, which H.S.M. Coxeter published in the American Mathematical Monthly with the single word “Behold!”

In 2011 Australian architect Horst Kiechle created an entire human torso from paper, as a geometric sculpture, for the science lab at the International School Nadi in Fiji.
He’s made the templates available for free — you can fold your own paper man, complete with removable organs.
Completed in 1997, German artist Jo Niemeyer’s 20 Steps Around the Globe installed 20 high-grade steel columns on a great circle around the earth, establishing the distances between them using the golden ratio φ, 1.61803398875.
The first poles, shown here, were erected in Finnish Lapland, north of the polar circle. The first two were placed 0.458 meters apart; the third was placed 0.458 × φ = 0.741 meters beyond the second; and so on, marching off in a beeline toward the horizon. The first 12 poles are in Finland; the 13th and 14th in Norway; the 15th, 16th, and 17th in Russia; the 18th in China; and the 19th in Australia. The 20th coincides with the first back in Finland.
In this way the project models the golden section and the Fibonacci sequence, tailoring them to our planet. Niemeyer calls it “an interdisciplinary expedition into the secrets of the power of limits.”
In 1680 Robert Hooke sprinkled a plate with flour, drew a violin bow across its edge, and saw the flour spring into surprising geometric shapes. The plate was resonating, driving the flour into invisible nodal lines on its surface that were not vibrating.
German physicist Ernst Chladni pursued these experiments in the 18th century and published his results in Discoveries in the Theory of Sound in 1787. Today they’re known as Chladni figures.
“The universe is full of magical things,” wrote Eden Phillpotts, “patiently waiting for our wits to grow sharper.”

In 1972 Canadian scientists R.W. Sheldon and S.R. Kerr set out to reason out the number of monsters that occupy Loch Ness. Because the creatures are reportedly large and rarely seen, it follows that their numbers must be small. (“It has been suggested from time to time that as the monsters are never caught it must therefore follow that they do not exist. This is both irresponsible and illogical.”)
By estimating the fish stock available in the loch, they determined that the total mass of monsters is between 3,135 and 15,675 kg. Taking the minimum monster size as 100 kg (“anything smaller is not suitably monstrous”), they estimate that the loch contains between 1 and 156 monsters. The high end of this range seems unlikely; and since monsters have been reported for centuries they’re probably breeding, which would require a population of at least 10.
Given the available quantity of fish and assuming a stable population, monsters weighing 100 kg would have to die at a rate of at least 3 per year. Larger animals would die less frequently, and this seems likely since dead monsters are never found (and since the juveniles that must replace them are never seen). So it seems the lake probably contains a small number of large monsters, perhaps 10-20 monsters weighing up to 1,500 kg each and measuring about 8 meters, “a size that agrees well with observational data.”
“We would like to thank Kate Kranck for drawing our attention to this problem, because until she mentioned it we were unaware that monsters were a problem.”
(“The Population Density of Monsters in Loch Ness,” Limnology and Oceanography 17:5, 796–798)
Label the faces of a fair set of dice with these numbers:
Die A: 3, 3, 3, 3, 3, 6
Die B: 2, 2, 2, 5, 5, 5
Die C: 1, 4, 4, 4, 4, 4
This gives them a curious property. In the long run Die A will tend to beat Die B, Die B will tend to beat Die C, and Die C will tend to beat Die A. The three dice form a ring in which each die beats its successor. No matter which die our opponent chooses, we can select another that is likely to beat it.
Business magnate Warren Buffet once challenged Bill Gates to such a game using four nontransitive dice. “Buffett suggested that each of them choose one of the dice, then discard the other two,” wrote Janet Lowe in her 1998 book Bill Gates Speaks. “They would bet on who would roll the highest number most often. Buffett offered to let Gates pick his die first. This suggestion instantly aroused Gates’s curiosity. He asked to examine the dice.”
“It wasn’t immediately evident that because of the clever selection of numbers for the dice, they were nontransitive,” Gates said. “Assuming the dice were rerolled, each of the four dice could be beaten by one of the others.” He invited Buffett to choose first.