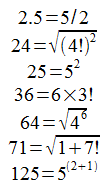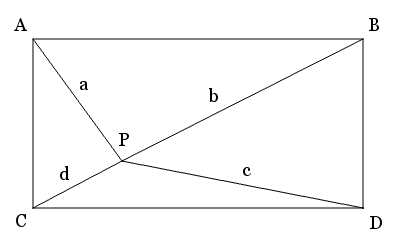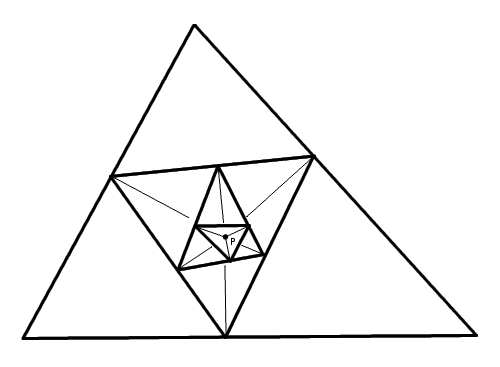
From a point P, drop perpendiculars to the sides of a surrounding triangle. This defines three points; connect those to make a new triangle and drop perpendiculars to its sides. If you continue in this way, the fourth triangle will be similar to the original one.
In 1947, Mary Pedoe memorialized this fact with a poem:
Begin with any point called P
(That all-too-common name for points),
Whence, on three-sided ABC
We drop, to make right-angled joints,
Three several plumb-lines, whence ’tis clear
A new triangle should appear.
A ghostly Phoenix on its nest
Brooding a chick among the ashes,
ABC bears within its breast
A younger ABC (with dashes):
A figure destined, not to burn,
But to be dropped on in its turn.
By going through these motions thrice
We fashion two triangles more,
And call them ABC (dashed twice)
And thrice bedashed, but now we score
A chick indeed! Cry gully, gully!
(One moment! I’ll explain more fully.)
The fourth triangle ABC,
Though decadently small in size,
Presents a form that perfectly
Resembles, e’en to casual eyes
Its first progenitor. They are
In strict proportion similar.
The property generalizes: Not only is the third “pedal triangle” of a triangle similar to the original triangle, but the nth “pedal n-gon” of an n-gon is similar to the original n-gon.