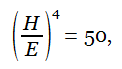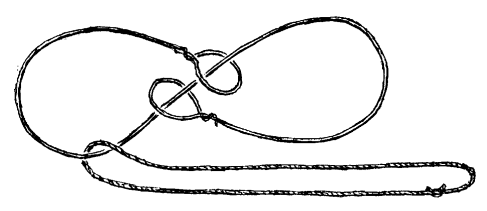
Can this loop of string be freed from its wire? Stewart Coffin, who devised the puzzle in 1974, writes, “I soon became convinced that this was impossible, but being a novice in the field of topology, I was at a loss for any sort of formal proof.” He published the challenge in a newsletter and has been receiving requests for a solution ever since. Adding to the confusion, in 1976 a British puzzle editor mistakenly claimed with that Coffin’s creation was equivalent to another puzzle with a known solution, and Pieter van Delft and Jack Botermans published an amusingly bewildering “solution” of their own in their 1978 book Creative Puzzles of the World.
In the meantime, fans around the world have continued to experiment, and mathematicians Inta Bertuccioni and Paul Melvin have both offered proofs that the puzzle is unsolvable. “Whoever would have guessed that this little bent piece of scrap wire and loop of string would launch itself on an odyssey that would carry it around the world?” Coffin writes. “Will it mischievously rise again, perhaps disguised in another form, as topological puzzles so often do?”