Lewis Carroll demonstrates a problem with deciding an election by plurality of votes:
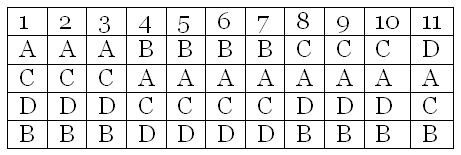
Four candidates are ranked by each of 11 electors, and each elector votes for his first choice. “Here A is considered best by three of the electors, and second by all the rest. It seems clear that he ought to be elected; and yet, by the above method, B would be the clear winner — a candidate who is considered worst by seven of the electors!”
“It is a matter for the deepest regret that Dodgson never completed the book he planned to write on this subject,” writes Michael Dummett. “Such was the lucidity of his exposition and mastery of this topic that it seems possible that, had he published it, the political history of Britain would have been significantly different.”
See The Voting Paradox, The Referendum Paradox, and Stump Trouble.