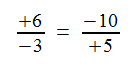- Canada’s coastline is six times as long as Australia’s.
- Rudyard Kipling invented snow golf.
- ENUMERATION = MOUNTAINEER
- Can you see your eyes move in a mirror?
- 26364 = 263 × 6/4
- “I want death to find me planting my cabbages.” — Montaigne
Science & Math
Some Mirror Puzzles

Hold a match horizontally before a mirror. If the match’s head is to the right, then so is that of its reflection — the match is not reversed left to right. But now hold up the matchbox. Its writing is reversed. Why?
“Here is a related puzzle,” writes psychologist Richard Gregory in Mirrors in Mind (1997). “Hold a mug with writing on it to a mirror. What do you see in the mirror? The reflection of the handle is unchanged — but the writing is right-left reversed. Can a mirror read?!”
Set two mirrors together at a 60° angle and rotate the pair around your line of sight. Your image is preserved despite the mirrors’ rotation. This is not the case if the mirrors are set at 45° or 90°. Why?
Misc
- Hastie Love was convicted of rape in Tennessee in 1968.
- Zebra stripes are white.
- 14641 = (1 + 4 + 6)4 × 1
- Spain’s national anthem has no words.
- LION + TIGER = LOITERING
- “Character is that which can do without success.” — Emerson
Uh-Oh
Can a fraction whose numerator is less than its denominator be equal to a fraction whose numerator is greater than its denominator? If not, how can
In the proportion
+6 : -3 :: -10 : +5
is not either extreme greater than either mean? What has become of the old rule, ‘greater is to less as greater is to less’?
— William Frank White, A Scrap-Book of Elementary Mathematics, 1908
The Ames Window
Psychologist Adelbert Ames, inventor of the Ames room, also devised this illusion. In which direction is the window turning?
“The Chemist to His Love”
I love thee, Mary, and thou lovest me–
Our mutual flame is like th’ affinity
That doth exist between two simple bodies:
I am Potassium to thine Oxygen.
‘Tis little that the holy marriage vow
Shall shortly make us one. That unity
Is, after all, but metaphysical.
Oh, would that I, my Mary, were an acid,
A living acid; thou an alkali
Endow’d with human sense, that, brought together,
We both might coalesce into one salt,
One homogeneous crystal. Oh, that thou
Wert Carbon, and myself were Hydrogen;
We would unite to form olefiant gas,
Or common coal, or naphtha–would to heaven
That I were Phosphorus, and thou wert Lime!
And we of Lime composed a Phosphuret.
I’d be content to be Sulphuric Acid,
So that thou might be Soda. In that case
We should be Glauber’s Salt. Wert thou Magnesia
Instead we’d form the salt that’s named from Epsom.
Couldst thou Potassa be, I Aqua-fortis,
Our happy union should that compound form,
Nitrate of Potash–otherwise Saltpetre.
And thus our several natures sweetly blent,
We’d live and love together, until death
Should decompose the fleshly tertium quid,
Leaving our souls to all eternity
Amalgamated. Sweet, thy name is Briggs
And mine is Johnson. Wherefore should not we
Agree to form a Johnsonate of Briggs?
— “A Rochester druggist,” quoted in The Medical Age, Oct. 11, 1886
Math Notes
512 × 1953125 = 1000000000
262144 × 3814697265625 = 1000000000000000000
8589934592 × 116415321826934814453125 = 1000000000000000000000000000000000
Great Men
Dr. Franklin had a party to dine with him one day at Passy, of whom one-half were Americans, the other half French, and among the last was the Abbé Raynal. During the dinner he got on his favorite theory of the degeneracy of animals, and even of man in America, and urged it with his usual eloquence. The Doctor, at length, noticing the accidental stature and position of his guests at table, ‘Come,’ says he, ‘M. l’Abbé, let us try this question by the fact before us. We are here one-half Americans and one-half French, and it happens that the Americans have placed themselves on one side of the table, and our French friends are on the other. Let both parties rise, and we will see on which side nature has degenerated.’ It happened that his American guests were Carmichael, Harmer, Humphreys, and others of the finest stature and form; while those on the other side were remarkably diminutive, and the Abbé himself particularly, was a mere shrimp. He parried the appeal by a complimentary admission of exceptions, among which the Doctor himself was a conspicuous one.
— Thomas Jefferson, quoted in James Parton, Life and Times of Benjamin Franklin, 1864
Skyward

In 1964, as the Apollo program prepared to land a man on the moon, it received unexpected news from Zambia. “I’ll have my first Zambian astronaut on the moon by 1965,” announced Edward Mukaka Nkoloso, a grade-school science teacher and director-general of the Zambian National Academy of Space Research.
“We are using our own system, derived from the catapult,” he explained. It would fire a 10-foot aluminum and copper rocket that would carry 10 Zambian astronauts ultimately to Mars.
“I’m getting them acclimatized to space travel by placing them in my space capsule every day. It’s a 40-gallon oil drum in which they sit, and I then roll them down a hill. This gives them the feeling of rushing through space. I also make them swing from the end of a long rope. When they reach the highest point, I cut the rope — this produces the feeling of free fall.”
Unfortunately, “I’ve had trouble with my spacemen and spacewomen,” Nkoloso complained. “They won’t concentrate on spaceflight; there’s too much lovemaking when they should be studying the moon. Matha Mwamba, the 17-year-old girl who has been chosen to be the first woman on Mars, has also to feed her 10 cats, who will be her companions on her long space flight.”
The U.N. denied the £700 million Nkoloso needed “to really get going,” but his enthusiasm remained undiminished. In 1968 he congratulated the returning Apollo 8 team but urged: “Let us make a Zambian rocket today. We shall never be content to remain behind other races. This is our heavenly destiny, our natural ambition and cultural hegemony.”
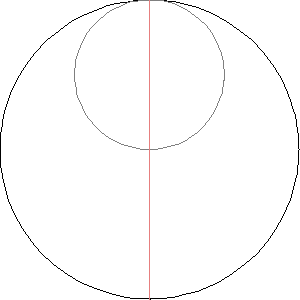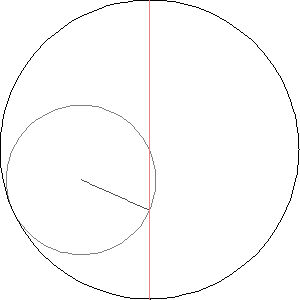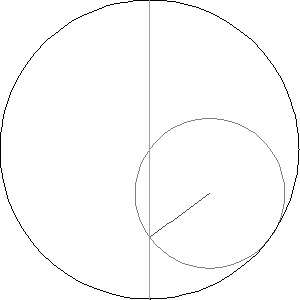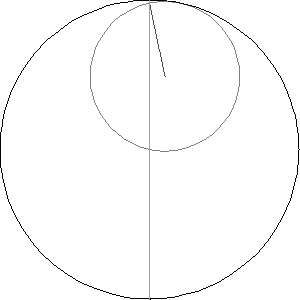
Rolling

Suppose we set a small circle rolling around the interior of a large circle of twice its diameter. If we follow a point on the small circle, what pattern will it draw?