59 + 39 + 49 + 49 + 99 + 49 + 89 + 39 + 69 = 534494836
Science & Math
One More Try
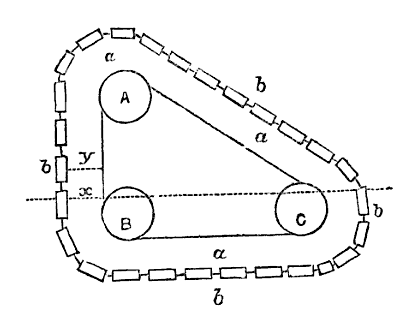
A perpetual-motion scheme by William Congreve. A, B, and C are three horizontal rollers fixed in a frame. They’re surrounded by a continuous band of sponge, a, and that’s surrounded by a chain of weights, b. Immerse the whole thing partially in a cistern. The sponge on the left will absorb water by capillary action, say from x to y; the sponge on the right will not (because the weights squeeze it out!). “The band will begin to move in the direction A B; and as it moves downwards, the accumulation of water will continue to rise, and thereby carry on a constant motion.”
From Henry Dircks, Perpetuum Mobile; or, Search for Self-Motive Power, 1861.
Can Do

In 1921, chemists at Arthur D. Little Inc. reduced 100 pounds of sows’ ears to glue, converted it to gelatin, forced it into fine strands, and wove these into a purse “of the sort which ladies of great estate carried in medieval days — their gold coin in one end and their silver coin in the other.”
“We made this silk purse from a sow’s ear because we wanted to, because it might serve as an example to clients who come to us with their ambitions or their troubles, and also as a contribution to philosophy,” they reported. “Things that everybody thinks he knows only because he has learned the words that say it, are poisons to progress.”
Canceling Zeros

Misc
- Christopher Lee is Ian Fleming’s cousin.
- £12.12s.8d = 12128 farthings
- ii is real.
- Shouldn’t Juliet have asked, “Wherefore art thou Montague?”
- “Of soup and love, the first is the best.” — Thomas Fuller
Speed Limits
The cheetah can reach speeds over 70 mph. In a dive, the peregrine falcon can reach 200 mph. But in 1927, entomologist Charles Townsend estimated that the deer botflies he’d observed in New Mexico surpassed both of these, reaching 400 yards per second. That’s 818 mph.
This claim stood for 11 years, until in 1938 chemist Irving Langmuir debunked it in Science:
- The power needed to maintain this speed amounts to 370 watts, or about half a horsepower. To deliver it, the fly would have to consume 1.5 times its own weight in food every second.
- Ballistics formulas show that the wind pressure on the fly’s head would amount to 8 pounds per square inch, probably enough to crush the fly.
- An 800 mph fly would strike the skin with a force of 310 pounds. “It is obvious that such a projectile would penetrate deeply into human flesh.”
- A supersonic fly would be invisible to the eye, not the “brownish blur” that Townsend had described.
Not to mention that an 800 mph fly would create its own sonic boom. After weighing the facts, Langmuir concluded, “The description given by Dr. Townsend of the appearance of the flies seems to correspond best with a speed in the neighborhood of 25 m/hr.”
Space Bills
When an explosion crippled Apollo 13’s command module, the crew used the lunar module as a “lifeboat.” The two modules had been built by different contractors, so when the mission was over Grumman sent a tongue-in-cheek bill to Rockwell for “towing” the ship to the moon and back:

The Associated Press reported that “North American Rockwell replied that the invoice had been examined by the company’s auditor, who pointed out that North American Rockwell had not yet received payment for ferrying LMs to the moon on previous missions.”
(Thanks, Perry.)
The Monster Study
In 1939, University of Iowa graduate student Mary Tudor began an experiment with local orphans, warning them that they were showing signs of stuttering and lecturing them whenever they repeated a word. The children became acutely self-conscious, and many began to stutter, fulfilling the theory that “the affliction is caused by the diagnosis.”
Sixty years later, when Tudor was 84, she received a letter from one of the orphans. It was addressed to “Mary Tudor Jacobs The Monster.”
“You destroyed my life,” it ran. “I could have been a scientist, archaeologist or even president. Instead I became a pitiful stutterer. The kids made fun of me, my grades fell off, I felt stupid. Clear into my adulthood, I still want to avoid people to this day.”
“I didn’t like what I was doing to those children,” Tudor told the San Jose Mercury News in 2001. “It was a hard, terrible thing. Today, I probably would have challenged it. Back then you did what you were told. It was an assignment. And I did it.”
Rigged Latin
When entomologist Paul Marsh was given the chance to name two wasp species in the genus Heerz, he called them Heerz tooya and Heerz lukenatcha.
The International Commission on Zoological Nomenclature insists that “A zoologist should not propose a name that, when spoken, suggests a bizarre, comical, or otherwise objectionable meaning.” But a few get through. Examples:
- Vini vidivici (parrot)
- Apopyllus now (spider)
- Lalapa lusa (wasp)
- Agra vation (beetle)
- Phthiria relativitae (bee fly)
- Pison eyvae (wasp)
- Eubetia bigaulae (“you betcha by golly”) (moth)
Three mythicomyiid flies are named Pieza kake, Pieza pi, and Pieza deresistans.
In 1912 the Zoological Society of London criticized entomologist George Kirkaldy for giving a series of hemipterans the generic names Polychisme, Elachisme, Marichisme, Dolichisme, Florichisme, and Ochisme (“Polly kiss me,” “Ella kiss me,” “Mary kiss me,” “Dolly kiss me,” “Flora kiss me,” “Oh, kiss me!”). In the same spirit, in 2002 a hopeful Neal Evenhuis named a fossil mythicomyiid Carmenelectra shechisme. “The offer’s still good,” he told the Chicago Tribune in 2008. “I’ll be willing to meet her.”
Head Games
The present King of France is bald.
Is this statement true or false? Well, it’s not true — France has no king presently. But if it’s false then its negation ought to seem true: The present King of France is not bald. That’s no better. Yet it’s not gibberish — the sentence seems to have a clear meaning that we can understand.
Bertrand Russell and Alfred North Whitehead spent much of their time at Cambridge debating this point. “It is astonishing what intricate and remote considerations can be brought to bear on this interesting question,” Russell wrote to his wife. “We finally decided that he isn’t, altho’ he has no hair of his own. Experienced people will infer that he wears a wig, but this would be a mistake.”
