
“Everyone believes in the normal law, the experimenters because they imagine that it is a mathematical theorem, and the mathematicians because they think it is an experimental fact.” — Gabriel Lippmann, in a letter to Henri Poincaré
(Thanks, Tom.)

“Everyone believes in the normal law, the experimenters because they imagine that it is a mathematical theorem, and the mathematicians because they think it is an experimental fact.” — Gabriel Lippmann, in a letter to Henri Poincaré
(Thanks, Tom.)
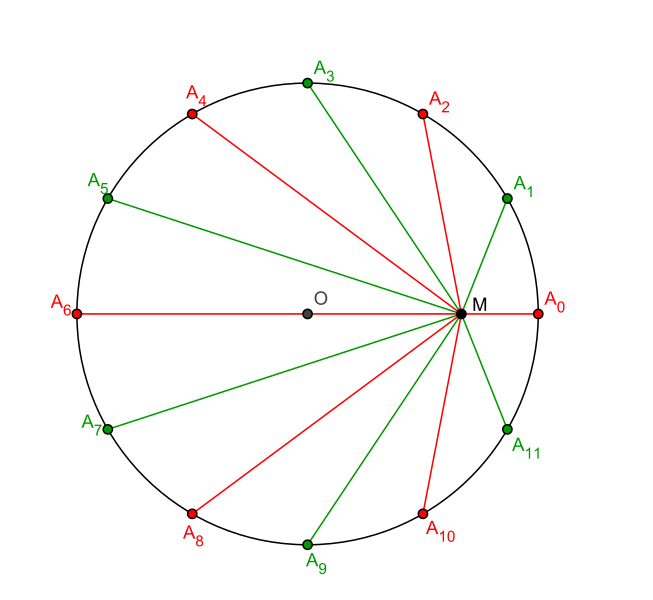
The product of the distances from N equally spaced points on a unit circle to a point M that lies on the x axis at distance x from circle origin O and on a line passing through the first point, A0, on the circle equals 1 – xN.
Discovered by Roger Cotes (1682-1716). Here’s a proof.
In 2006, German entomologist Jochen-P. Saltin discovered a new species of rhinoceros beetle in Peru, which he dubbed Megaceras briansaltini.
Remarkably, the insect’s horn closely resembles that of Dim, the blue rhinoceros beetle in the Disney film A Bug’s Life, which was released eight years earlier.
“I know of no dynastine head horn that has ever had the shape of the one seen in M. briansaltini, and so its resemblance to a movie character seems like a case of nature mimicking art … or what could be referred to as ‘the Dim Effect,'” wrote entomologist Brett C. Ratcliffe.
“There are numerous examples of art mimicking nature (paintings, sculpture, etc.), but that cannot be the case here, because there had never been a known rhinoceros beetle in nature upon which the creators of Dim could have used as a model for the head horn. In my experience, then, Dim was the first ‘rhinoceros beetle’ to display such a horn, and the discovery of M. briansaltini, a real rhinoceros beetle, came later.”
(Brett C. Ratcliffe, “A Remarkable New Species of Megaceras From Peru [Scarabaeidae: Dynastinae: Oryctini]. The ‘Dim Effect’: Nature Mimicking Art,” The Coleopterists Bulletin 61:3 [2007], 463-467.)

Absolutely hands down one of the most beautiful places to see from space is the Caribbean. You see an entire rainbow of blue. From the light emerald green to the green-blue to the blue-green to the aquamarine to the slowly increasingly darker shades of blue down to the really deep colors that come with the depths of a really deep ocean. You can see all that at one time from space. It’s very curvy, it’s not harsh geometric lines. It’s swirls and whirls and all kinds of wavy lines. It looks like a piece of modern art.
— Astronaut Sandra Magnus, quoted in Ariel Waldman, What’s It Like in Space?, 2016
Swedish botanist Elias Tillander (1640–1693) was so “harassed by Neptune” during a trip across the Gulf of Bothnia from Stockholm to Turku that he made the return journey overland and changed his name to Til-Landz (“to land”).
Linnaeus named the evergreen plant Tillandsia after him — it cannot tolerate a damp climate.
(From Wilfrid Blunt, Linnaeus: The Compleat Naturalist, 2001.)
In the 1967 Star Trek episode “The Trouble With Tribbles,” a small furry alien species is introduced on board the Enterprise and after three days grows to 1,771,561 individuals. In 2019 University of Leicester physics undergraduate Rosie Hodnett and her colleagues wondered how long it would take for the creatures to fill the whole starship. Using Mr. Spock’s estimate that each tribble produces 10 offspring every 12 hours and assuming that each tribble occupies 3.23 × 10-3 m3 and that the volume of the Enterprise is 5.94 × 106 m3, they found that the ship would reach its limit of 18.4 × 109 tribbles in 4.5 days.
A separate inquiry found that after 5.16 days the accumulated tribbles would be generating enough thermal energy to power the warp drive for 1 second.
(Rosie Hodnett et al., “Tribbling Times,” Journal of Physics Special Topics, Nov. 18, 2019.)
From reader Chris Smith:
Pick a three-digit number in which all the digits are different. Example: 314.
Now list every possible combination of two digits from the chosen number. In our example, these are 13, 14, 31, 34, 41, and 43.
Divide the sum of these two-digit numbers by the sum of the three digits in the original number, and you’ll always get 22. In our example, (13 + 14 + 31 + 34 + 41 + 43) / (3 + 1 + 4) = 176/8 = 22.
This works because 10a + b, 10a + c, 10b + a, 10b + c, 10c + a, and 10c + b sum to 22a + 22b + 22c = 22(a + b + c), so dividing by a + b + c will always give 22.
(Thanks, Chris.)
06/08/2024 Reader Tom Race points out that essentially the same trick can be performed using the entire number: If you add all six permutations of the original 3 digits, then divide that total by the sum of the 3 digits, the answer is always 222.
For example, using 561:
561 + 516 + 156 + 165 + 651 + 615 = 2664
5 + 6 + 1 = 12
2664 / 12 = 222
“This works because in the first sum each of the three digits (a, b and c) occurs twice in each of the three columns, so the sum is 222a + 222b + 222c = 222(a + b + c).” (Thanks, Tom.)

The upside-down catfish, Synodontis nigriventris, is right side up. Or, rather, it’s adapted to spend most of its time upside down — its belly is darker than its back, and it swims fastest in this inverted position. The behavior may have evolved to help it reach food on the undersides of submerged branches or to breathe dissolved oxygen near the surface.
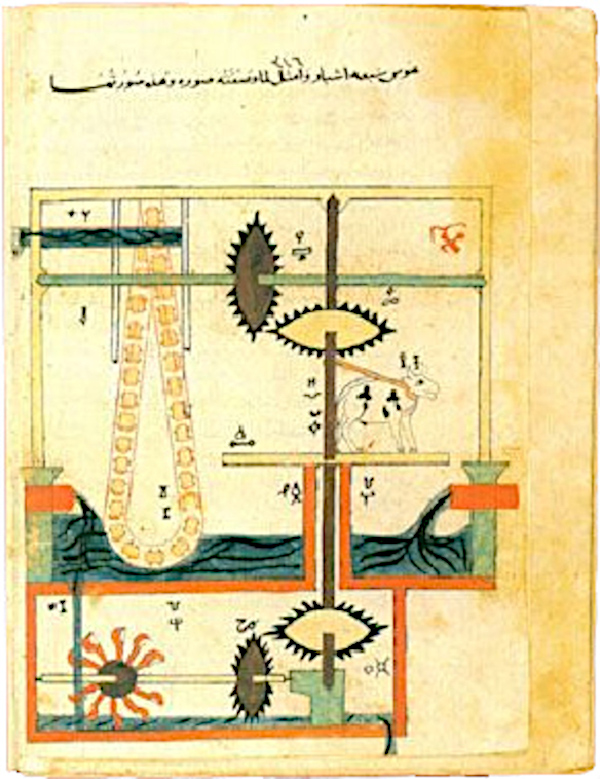
Here’s a surprise: The Book of Knowledge of Ingenious Mechanical Devices, a 1206 manuscript by the Turkish author Ismail al-Jazari, depicts a chain pump in the form of a Möbius strip. A rope bearing a chain of cups dips them successively into a water source at the bottom and then pours them into a course at the top. The single, continuous rope makes two passes through this route, describing the edges of a strip with a half twist so that the cups suspended between the loops are turned 180 degrees with each pass. This would permit the cups to last longer, since they’re worn more evenly, and even a broken cup might still convey some water with every second pass.
(Julyan H.E. Cartwright and Diego L. González, “Mobius Strips Before Mobius: Topological Hints in Ancient Representations,” Mathematical Intelligencer 38:2 [June 2016], 69-76.)