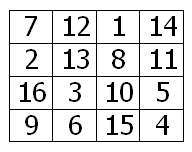
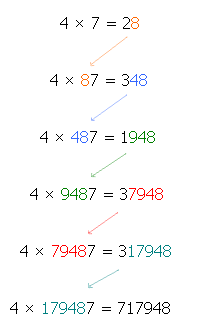
The following successful though illegal operation is reported by Professor A. Gloden in Sphinx, Volume VI, Number 7. In multiplying 6 2/3 by 4 4/5 a student first found the product of the integers, 6 × 4 = 24. He then reduced the fractions to the common denominator … and divided the product of the numerators 10 × 12 by the common denominator 15. The result 24 + 8 = 32 is correct.
In the same way he obtained the correct value of the product 9 3/5 × 2 2/4.
— “Curiosa,” Scripta Mathematica, October 1936