- River Phoenix was born River Bottom.
- Every natural number is the sum of four squares.
- What happens if Pinocchio says, “My nose will grow now”?
- Shakespeare has no living descendants.
- “All generalizations are dangerous — even this one.” — Dumas
Science & Math
Math Notes
1000000000000000000000000000000000000000000000000000000000000000
0000000000000000000000000000000000000000000000000000000000000000
0000000000000000000000000000000000000000000000000000000000000000
000002569 is prime.
You Are Here
In “Partial Magic in the Quixote,” Borges quotes philosopher Josiah Royce:
Let us imagine that a portion of the soil of England has been levelled off perfectly and that on it a cartographer traces a map of England. The job is perfect; there is no detail of the soil of England, no matter how minute, that is not registered on the map; everything has there its correspondence. This map, in such a case, should contain a map of the map, which should contain a map of the map of the map, and so on to infinity.
This sequence tends to a single point, the point on the map that corresponds directly to the point it represents in the territory.
Cover England entirely with a 1:1 map of itself, then crumple the map into a ball. So long as it remains in England, the balled map will always contain at least one point that lies directly above the corresponding point in England.

Related (sort of): In The Humor and Drama of Early Texas (2003), George U. Hubbard notes that one day in 1865, Thomas Jefferson Chambers was standing in this house in a room containing his portrait when someone fired a shot through the second-story window. “The bullet passed through Chambers’ body and lodged in his portrait on the wall. The citizens of Anahuac thought it very singular that the bullet that killed him struck the portrait in exactly the same place it had passed through his body.” The crime was never solved.
See Garganta and Papered Over.
Half-Hearted
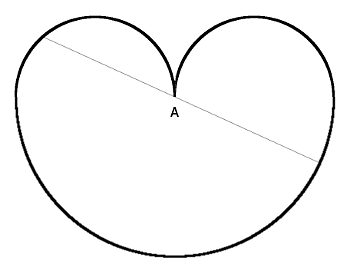
Draw a semicircle and surmount it with two smaller semicircles.
A line drawn through A, at any angle, will divide the perimeter precisely in half.
This probably has some romantic symbolism, but I’m not very good at that stuff.
06/19/2024 Very belated update: This is called the cardioid of Boscovich, after Roger Boscovich, 1711-1787. There’s a proof of the theorem in Alsina and Nelsen, Icons of Mathematics.
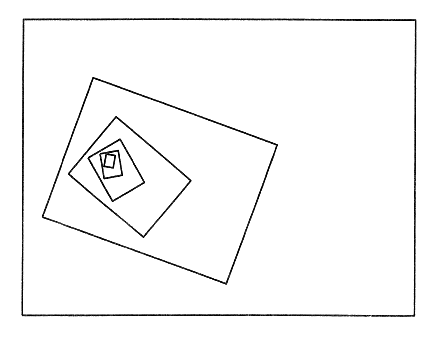
Squares Into Squares
Spirolaterals
Imagine you have a little robot that holds a pencil. Set it down on a sheet of paper and give it these instructions:
- Move forward 3 units and turn right.
- Move forward 1 unit and turn right.
- Move forward 2 units and turn left.
- Move forward 1 unit and turn left.
- Move forward 2 units and turn right.
- Repeat.
If the robot makes its turns at 90° angles, it will produce this figure:

But, remarkably, if it turns at 120° it will draw this:

The Grid Flower

Any pair of points define an infinity of ellipses and an infinity of hyperbolas.
The ellipses do not touch one another, nor do the hyperbolas.
But every ellipse meets every hyperbola at a right angle.
The Black Hole
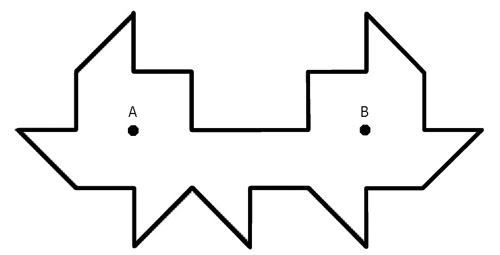
If Satan plays miniature golf, this is his favorite hole. A ball struck at A, in any direction, will never find the hole at B — even if it bounces forever.
The idea arose in the 1950s, when Ernst Straus wondered whether a room lined with mirrors would always be illuminated completely by a single match.
Straus’ question went unanswered until 1995, when George Tokarsky found a 26-sided room with a “dark” spot; two years later D. Castro offered the 24-sided improvement above. If a candle is placed at A, and you’re standing at B, you won’t see its reflection anywhere around you — even though you’re surrounded by mirrors.
The Franklin Square
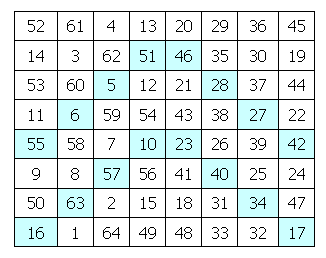
In a 1769 letter, Ben Franklin describes a magic square he devised in his youth. The magic total of 260 can be reached by adding the numbers in each row or column, as in a normal magic square. But “bent rows” (shaded) produce the same total, even when “wrapped across” the border of the table. This works in all four directions.
Further: Half of each row or column sums to half of 260, as does any 2×2 subsquare. And the four corners and the four center squares sum to 260. (Alas, the main diagonals don’t, so this doesn’t strictly qualify as a magic square by the modern definition.)
Interestingly, no one knows how Franklin created the square. Many methods have been devised, but none apparently as quick as his, which he claimed could generate them “as fast as he could write.”
The Perfect Family
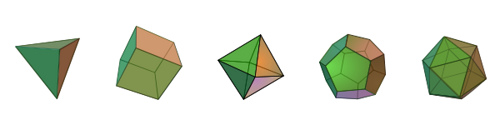
Take any Platonic solid, join the centers of its faces, and, charmingly, you get another Platonic solid. The cube and the octahedron produce one another, as do the dodecahedron and the icosahedron, and the tetrahedron produces another tetrahedron.
Bonus factoid: If you inscribe a dodecahedron and an icosahedron in the same sphere, the dodecahedron will occupy more of the sphere’s volume. It has fewer faces than the icosahedron, but its faces are more nearly circular, so it fits the sphere more snugly.
See The Pup Tent Problem.
