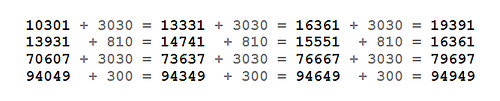Write down any natural number, reverse its digits to form a new number, and add the two:
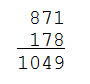
In most cases, repeating this procedure eventually yields a palindrome:
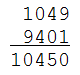

With 196, perversely, it does not — or, at least, it hasn’t in computer trials, which have repeated the process until it produced numbers 300 million digits long.
Is 196 somehow immune to producing palindromes? No one’s yet offered a conclusive proof — so we don’t know.