- Q is the only letter that does not appear in any U.S. state name.
- 6455 = (64 – 5) × 5
- North Dakota’s record high temperature (121°F) is higher than Florida’s (109°F).
- UNNOTICEABLY contains the vowels A, E, I, O, and U in reverse order.
- “An odd thought strikes me: We shall receive no letters in the grave.” — Samuel Johnson
Science & Math
No Vacancy
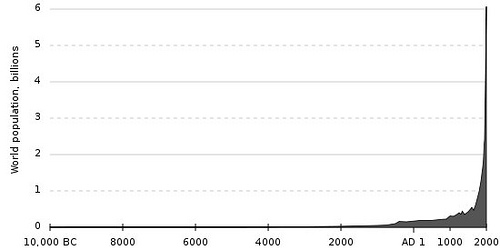
The world population has doubled between:
- 1181 and 1715
- 1715 and 1881
- 1881 and 1960
- 1960 and 1999
It’s expected to reach 9 billion by 2040.
Pandigital Squares
Square numbers containing all 10 digits unrepeated:
320432 = 1026753849
322862 = 1042385796
331442 = 1098524736
351722 = 1237069584
391472 = 1532487609
456242 = 2081549376
554462 = 3074258916
687632 = 4728350169
839192 = 7042398561
990662 = 9814072356
The Mirror
From Albert Beiler, Recreations in the Theory of Numbers (1964):
1 + 4 + 5 + 5 + 6 + 9 = 3 + 2 + 3 + 7 + 8 + 7
Pair each digit on the left with one on the right (for example, 13, 42, 53, 57, 68, 97). The sum of these six numbers will always equal its mirror image:
13 + 42 + 53 + 57 + 68 + 97 = 79 + 86 + 75 + 35 + 24 + 31
This works for all 720 possible combinations.
Most remarkably, you can square every term in these equations and they still hold:
132 + 422 + 532 + 572 + 682 + 972 = 792 + 862 + 752 + 352 + 242 + 312
Perpetual Notion
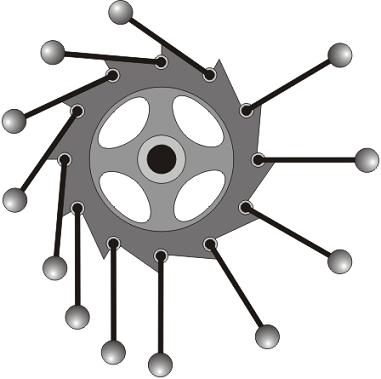
The balls on the right exert greater torque than those on the left, so the wheel ought to turn forever, right?
Sadly, the balls on the left are more numerous.
“If at first you don’t succeed,” wrote Quentin Crisp, “failure may be your style.”
Math Notes
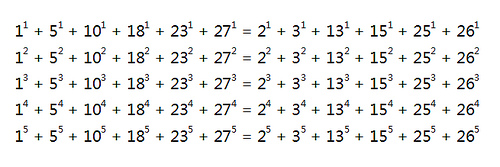
Multiplication Palindromes
12 × 42 = 24 × 21
12 × 63 = 36 × 21
12 × 84 = 48 × 21
13 × 62 = 26 × 31
23 × 96 = 69 × 32
24 × 63 = 36 × 42
24 × 84 = 48 × 42
26 × 93 = 39 × 62
36 × 84 = 48 × 63
46 × 96 = 69 × 64
14 × 82 = 28 × 41
23 × 64 = 46 × 32
34 × 86 = 68 × 43
13 × 93 = 39 × 31
Immortal Truth
In Scripta Mathematica, March 1955, Pedro A. Pisa offers an unkillably valid equation:
123789 + 561945 + 642864 = 242868 + 323787 + 761943
Hack away at its terms, from either end, and it remains true:
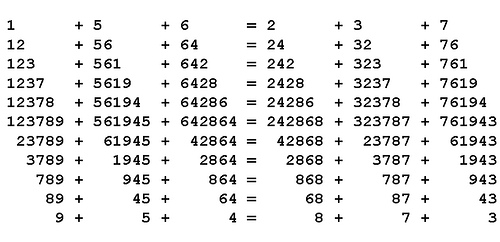
Stab it in the heart, removing the two center digits from each term, and it still balances:
1289 + 5645 + 6464 = 2468 + 3287 + 7643
Do this again and it still balances:
19 + 55 + 64 = 28 + 37 + 73
Most amazing: You can square every term above, in every equation, and they’ll all remain true.
12/03/2016 UPDATE: Reader Jean-Claude Georges discovered that the equalities remain valid when any combination of digits is removed consistently across terms. For example, starting from
123789 + 561945 + 642864 == 242868 + 323787 + 761943,
removing the 1st, 3rd and 5th digit from each number:
x2x7x9 + x6x9x5 + x4x8x4 == x4x8x8 + x2x7x7 + x6x9x3
gives
279 + 695 + 484 = 488 + 277 + 693 (= 1458)
and squaring each term gives
2792 + 6952 + 4842 = 4882 + 2772 + 6932 (=795122).
Amazingly, the same is true for any combination — for example, the equations remain valid when the 1st, 2nd, 4th, and 6th digits of each term are removed. (Thanks, Jean-Claude.)
Math Notes
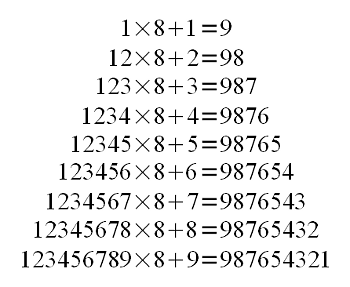
Nature, Nurture
Identical twins Jack Yufe and Oskar Stohr were born in 1932 to a Jewish father and a Catholic mother. Their parents divorced when the boys were six months old; Oskar was raised by his grandmother in Czechoslovakia, where he learned to love Hitler and hate Jews, and Jack was raised in Trinidad by his father, who taught him loyalty to the Jews and hatred of Hitler.
At 47 they were reunited by scientists at the University of Minnesota. Oskar was a conservative who enjoyed leisure, Jack a liberal workaholic. But both read magazines from back to front, both wore tight bathing suits, both wrapped rubber bands around their wrists, both liked sweet liqueur and spicy foods, both had difficulty with math, both flushed the toilet before and after using it — and both enjoyed sneezing suddenly in elevators to startle other passengers.
See Doppelgangers.
