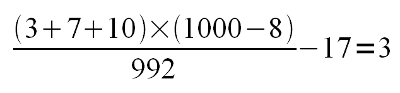- SCINTILLESCENT contains 7 pairs of letters.
- Rub two pennies together and you’ll see a third between them.
- Charles Darwin and Abraham Lincoln were born on the same day.
- 1285 = (1 + 28) × 5
- Squeeze an orange peel into a candle flame and you’ll produce a burst of fire.
Science & Math
A Candid Puzzle
Bertrand Russell admired G.E. Moore’s dedication to the truth.
“I have never but once succeeded in making him tell a lie,” he wrote, “and that was by a subterfuge.
“‘Moore,’ I said, ‘do you always speak the truth?’
“‘No,’ he replied.
“I believe this to be the only lie he ever told.”
Stop the World
Arguments against Galileo:
“Animals, which move, have limbs and muscles; the earth has no limbs or muscles, therefore it does not move.” — Scipio Chiaramonti, University of Pisa, 1633
“Buildings and the earth itself would fly off with such a rapid motion that men would have to be provided with claws like cats to enable them to hold fast to the earth’s surface.” — Libertus Fromundus, Anti-Aristarchus, 1631
“If we concede the motion of the earth, why is it that an arrow shot into the air falls back to the same spot, while the earth and all the things on it have in the meantime moved very rapidly toward the east? Who does not see that great confusion would result from this motion?” — Polacco, Anticopernicus Catholicus, 1644
More recent:
“[Astronomers give the rate of Earth’s rotation as 1,000 kilometers per hour.] An aircraft flying at this rate in the same direction as that of the rotation could not cover any ground at all. It would remain suspended in mid-air over the spot from which it took off, since both speeds are equal. There would, in addition, be no need to fly from one place to another situated on the same latitude. The aircraft could just rise and wait for the desired country to arrive in the ordinary course of the rotation, and then land; although it is difficult to see how any plane could manage to touch ground at all on an airfield which is slipping away at the rate of 1,000 kilometers per hour. It might certainly be useful to know what people who fly think of the rotation of the earth.” — Gabrielle Henriet, Heaven and Earth, 1957
See No Spin Zone.
Thanks Anyway

In 1776, Viennese schoolmaster Antonio Felkel factored every number up to 408,000. Few people bought the book, though, so the treasury recalled it and used the paper to make ammunition cartridges.
University of Prague professor J.P. Kulik spent 20 years extending the work to 100,000,000. He published it in six volumes in 1867.
Volume 2 has been lost.
The Collatz Conjecture
Think of any whole number greater than zero.
- If the number is even, divide it by two.
- If the number is odd, triple it and add one.
If you apply these rules repeatedly, will you always reach 1? Surprisingly, no one knows.
Paul Erdos said, “Mathematics is not yet ready for such confusing, troubling, and hard problems.”
An Artificial Aurora
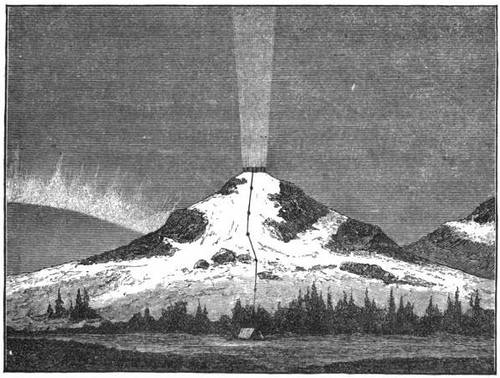
Karl Selim Lemström worked a quiet miracle in 1882: He strung conducting wire over the summit of a Lapland mountain and watched it draw down a shaft of light from the night sky — poetic proof that the aurora borealis is an electrical discharge from the upper atmosphere.
See Charged Words.
Satanic Compounds
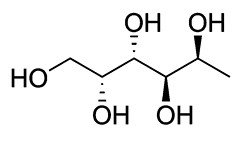
Here’s a sugar alcohol derived from the North Atlantic seaweed Fucus vesiculosus. It’s called fucitol.
And its optical isomers are called D-fuc-ol and L-fuc-ol.
The glycoprotein that vampire bats use to prevent their victims’ blood from clotting is called draculin.
And diethyl azodicarboxylate is explosive, shock-sensitive, carcinogenic, and an eye, skin, and respiratory irritant, which helps to justify its acronym: DEAD.
See Juvenile Chemistry.
Science Marches On
An “infallible remedy against epilepsy,” published in Paris in 1686:
Take of common polypody dried and powdered, of moss growing from the skull of a man who died by violent means (criminals preferred), of nail-filings from human hands and feet, two drachms each; piony root half an ounce, and of fresh misletoe half an ounce. Boil them together as the moon wanes; cool, strain, and administer in small doses.
Cited in Charles White, Three Years in Constantinople, 1846.
See Well, Hey!
Math Notes
88 + 88 + 58 + 98 + 38 + 48 + 78 + 78 = 88593477
Long Addition

In The Hunting of the Snark, the Butcher confirms for the Beaver that Two and One are Three:
Taking Three as the subject to reason about–
A convenient number to state–
We add Seven, and Ten, and then multiply out
By One Thousand diminished by Eight.
The result we proceed to divide, as you see,
By Nine Hundred and Ninety and Two:
Then subtract Seventeen, and the answer must be
Exactly and perfectly true.
Fittingly for Carroll, the math works: