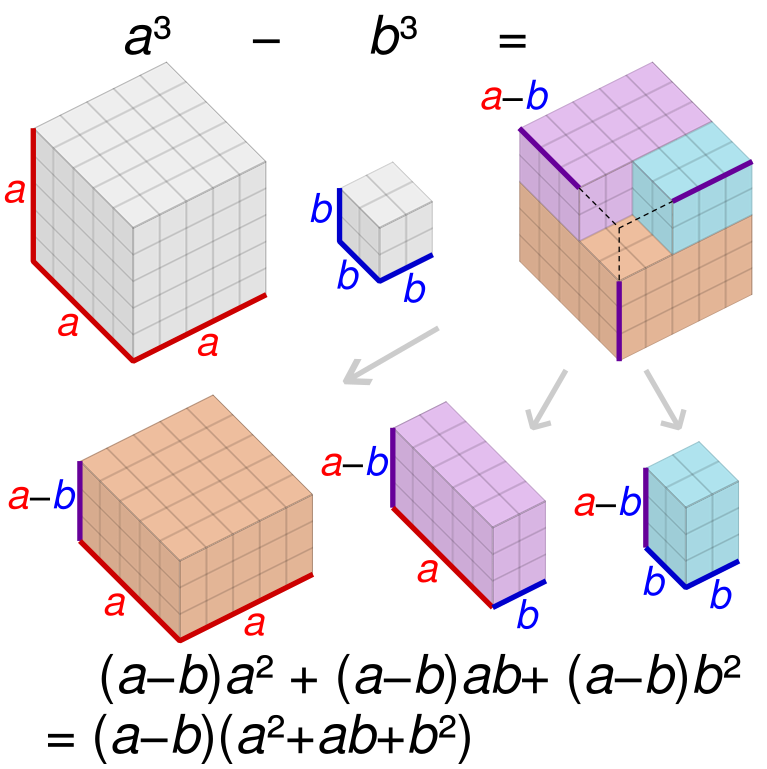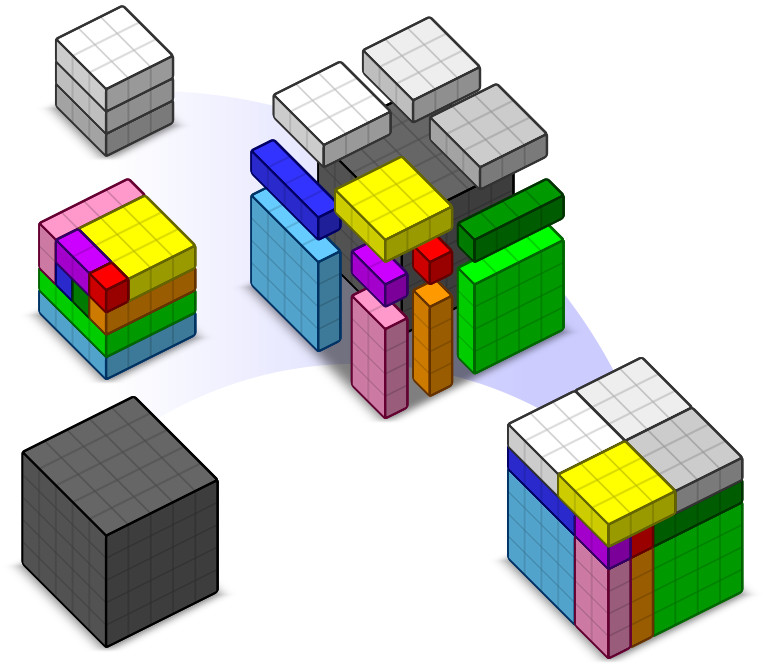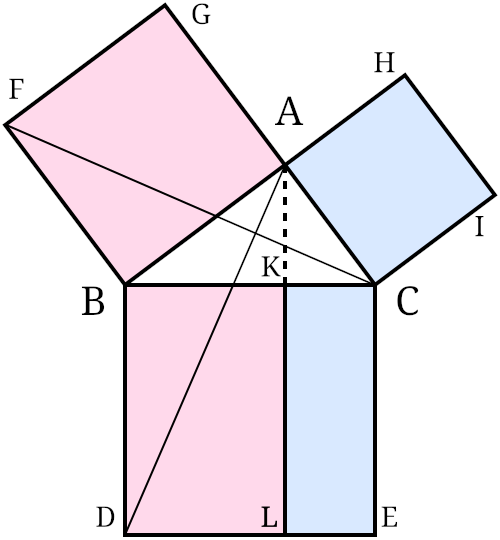
This is Euclid’s proof of the Pythagorean theorem — Schopenhauer called it a “brilliant piece of perversity” for its needless complexity:
- Erect a square on each leg of a right triangle. From the triangle’s right angle, A, draw a line parallel to BD and CE. This will intersect BC and DE perpendicularly at K and L.
- Draw segments CF and AD, forming triangles BCF and BDA.
- Because angles CAB and BAG are both right angles, C, A, and G are collinear.
- Because angles CBD and FBA are both right angles, angle ABD equals angle FBC, since each is the sum of a right angle and angle ABC.
- Since AB is equal to FB, BD is equal to BC, and angle ABD equals angle FBC, triangle ABD is congruent to triangle FBC.
- Since A-K-L is a straight line that’s parallel to BD, rectangle BDLK has twice the area of triangle ABD, because they share base BD and have the same altitude, BK, a line perpendicular to their common base and connecting parallel lines BD and AL.
- By similar reasoning, since C is collinear with A and G, and this line is parallel to FB, square BAGF must be twice the area of triangle FBC.
- Therefore, rectangle BDLK has the same area as square BAGF, AB2.
- By applying the same reasoning to the other side of the figure, it can be shown that rectangle CKLE has the same area as square ACIH, AC2.
- Adding these two results, we get AB2 + AC2 = BD × BK + KL × KC.
- Since BD = KL, BD × BK + KL × KC = BD(BK + KC) = BD × BC.
- Therefore, since CBDE is a square, AB2 + AC2 = BC2.
The diagram became known as the bride’s chair due to a confusion in translation between Greek and Arabic.