Is the question “What is an example of a question which is not its own answer?” its own answer?
Science & Math
The Paradox of the Knower
No one knows that this sentence is true.
That sentence can’t be false, because that would lead immediately to a contradiction.
But if it’s true, then omniscience is impossible.
Therefore there can be no all-knowing being.
The Ulam Spiral
Write the numbers from 41 to 440 in a square spiral:

Remarkably, all the numbers on the red diagonal are prime — even when the spiral is continued into a 20 × 20 square.
No one’s quite sure what to make of this. Polish mathematician Stanislaw Ulam discovered the pattern while doodling at a scientific meeting in 1963.
The Self-Flowing Flask
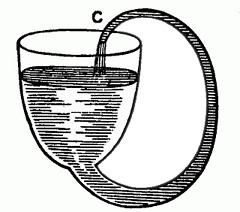
Denis Papin described this perpetual motion scheme in 1685. The pint of liquid in the goblet weighs more than the ounce in the tube, and so forces it down. When the liquid spills out the top of the tube and back into the goblet, it starts a cycle that must continue until the liquid finally evaporates. Where is the error?
Pieces of Pi
In the decimal expansion of π:
- the digits 27182818 — the first eight digits of e — appear at position 1,526,800.
- the digits 14142135 — the first eight digits of the square root of 2 — appear at position 52,638.
- the first eight digits of π itself — 31415926 — reappear at position 50,366,472.
- 16470 appears at position 16470.
- there are seven 7s at position 3,346,228, eight 8s at position 46,663,520, and six 9s at position 762.
- The White House switchboard number (456-1414) is at position 3,193,808, the population of France (65,073,482) is at position 98,709,092, and Disneyland’s zip code (92802) is at position 41,112.
Write out the alphabet starting with J:
JKLMNOPQRSTUVWXYZABCDEFGHI
Erase all letters that have left-right symmetry (such as A) and count the letters in each of the five groups that remain. (James Davis)
Proof That 4 Equals 5
The Bystander Paradox

You and I are walking through a dark alley. You are explaining that it’s wrong to kill an innocent person, but that it’s morally permissible to kill someone who is threatening your life.
At that moment an attacker leaps out of the shadows — and you find that, because of my position, you cannot defend yourself without also killing me.
In that instant, what is your moral obligation? You must not kill an innocent man. But my presence is threatening your life, so in that sense I am not innocent and can be sacrificed.
But if there’s no imperative to spare my life, then I’m no longer a hindrance to your self-defense … in which case I’m innocent again and you’re not justified in killing me.
And so on.
Euclid, Schmeuclid
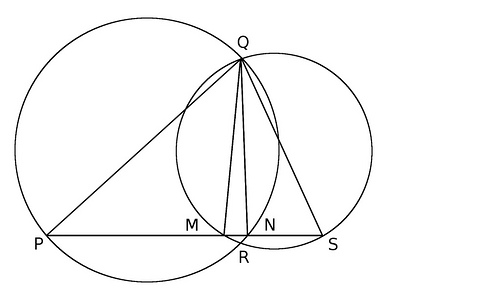
Consider any two circles intersecting at points Q and R. Their diameters are QP and QS. PS intersects the circles at M and N.
Now, any angle inscribed in a semicircle is a right angle. This means that both QNP and QMS are right angles — and thus that there are two perpendiculars from Q to PS. Doesn’t it?
Mrs. Miniver’s Problem

One of Jan Struther’s popular stories of the 1930s included the following passage:
She saw every relationship as a pair of intersecting circles. It would seem at first glance that the more they overlapped the better the relationship; but this is not so. Beyond a certain point the law of diminishing returns sets in, and there are not enough private resources left on either side to enrich the life that is shared. Probably perfection is reached when the area of the two outer crescents, added together, is exactly equal to that of the leaf-shaped piece in the middle. On paper there must be some neat mathematical formula for arriving at this; in life, none.
Interestingly, mathematicians who pursued the problem found that no precise solution is possible. With circles, as with relationships, we have to do the best we can.
Hard Bargaining

In Robert Louis Stevenson’s story “The Bottle Imp,” the titular imp will grant its owner (almost) any wish, but if the owner dies with the bottle then he burns in hell. He may sell the bottle, but he must charge less than he paid for it, and the new buyer must understand these conditions.
Now, no one would buy such a bottle for 1 cent, as he could not then sell it again. (The imp can’t make you immortal, or support prices smaller than one cent, or alter the conditions.) And if 1 cent is too low a price, then so is 2 cents, for the same reason. And so on, apparently forever. It would be irrational to buy the bottle for any price.
But intuitively most people would consider $1,000 a reasonable price to pay for the use of a wish-granting genie. Who’s right?
See also Tug of War.

