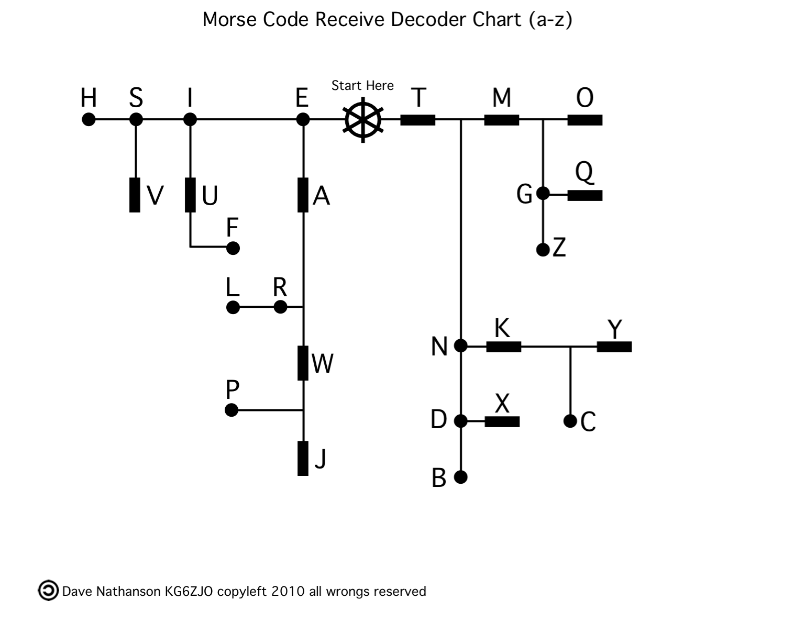
Science & Math
Misc
- Dorothy Parker said that James Thurber’s cartoon figures had the “semblance of unbaked cookies.”
- In typing AUTHENTICITY, the left and right hands alternate.
- P.G. Wodehouse wrote the last 26 pages of Thank You, Jeeves in one day.
- INTERROGATIVES is an anagram of both REINVESTIGATOR and TERGIVERSATION.
- 1232882 + 3287682 = 123288328768
(Thanks, Steve.)
Spotted
In a 1963 issue of Bokmakierie, a magazine for birdwatchers, Frank A. Goodliffe described a curiously familiar species he called Clericus polydenominata, the “dog-collared sombre blackbird”:
Identification: Similar to common laity but plumage and behaviour should serve to differentiate. Plumage black with narrow white collar — unbroken at throat. Feet black, of leathery appearance. Beak pink — often with blueish tint during winter months. When in groups are often seen with wings folded behind rump. … Habits: Usually found congregating with flocks of common laity, the females of which are frequently seen with plumage of vivid colours. Nesting: This usually occurs close to old buildings with spires. They are usually very friendly and may be seen around nesting sites of common laity at tea-time. … Call: The voice is distinctive, commencing ‘Brrrrr–rethren’ and continuing low and pleasant — often prolonged. Usually sings in congregations.
In a private booklet published four years later, M.A. Traylor suggested that the species belonged in the family of bishop birds.
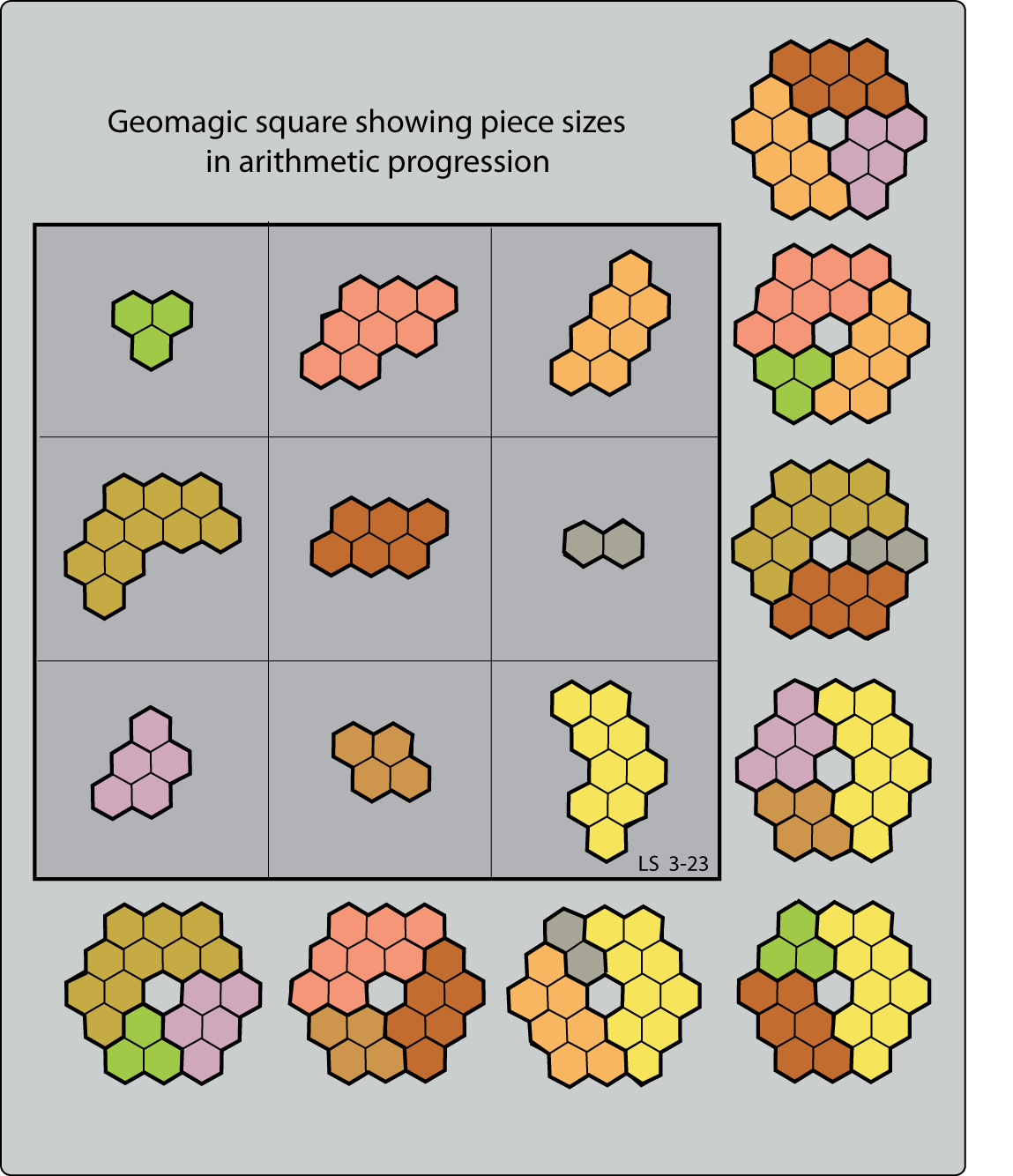
A Polyhex Square
Amalgamation
Putting mercury in contact with an aluminum plate has some surprising consequences (2:05).
“A Whisky Puzzle”
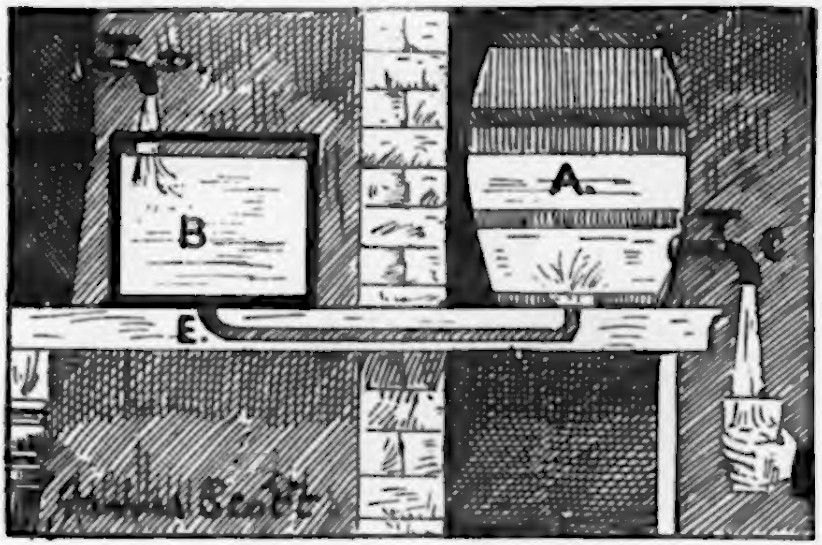
In 1895 a London shopkeeper attracted customers with a glass cask of whiskey — they were puzzled to find that no matter how much liquid they drew off, the level in the cask never dropped. The container could be viewed from any angle, and it stood well away from the wall. How was this possible?
A hidden pipe connected the bottom of the cask to a tank in another room. When a customer drew a glass of whisky, a confederate there would open a tap to replenish tank B, and the liquid, seeking its own level, would maintain the same height in the cask.
(James Scott, “Shopkeepers’ Advertising Novelties,” Strand, November 1895. See Desert Downpour.)
Pandigital Products
From reader Hans Havermann: Each of these equations uses each of the digits 0-9 exactly once:
8596 = 2×14×307
8790 = 2×3×1465
9360 = 2×4×15×78
9380 = 2×5×14×67
9870 = 2×3×1645
10752 = 3×4×896
12780 = 4×5×639
14760 = 5×9×328
14820 = 5×39×76
15628 = 4×3907
15678 = 39×402
16038 = 27×594 (= 54×297)
16704 = 9×32×58
17082 = 3×5694
17820 = 36×495 (= 45×396)
17920 = 8×35×64
18720 = 4×5×936
19084 = 52×367
19240 = 8×37×65
20457 = 3×6819
20574 = 6×9×381
20754 = 3×6918
21658 = 7×3094
24056 = 8×31×97
24507 = 3×8169
25803 = 9×47×61
26180 = 4×7×935
26910 = 78×345
27504 = 3×9168
28156 = 4×7039
28651 = 7×4093
30296 = 7×8×541
30576 = 8×42×91
30752 = 4×8×961
31920 = 5×76×84
32760 = 8×45×91
32890 = 46×715
34902 = 6×5817
36508 = 4×9127
47320 = 8×65×91
58401 = 63×927
65128 = 7×9304
65821 = 7×9403
(Thanks, Hans.)
Constitutional Crisis
Outcry
A curious observation by a British ornithologist during World War I:
The Zeppelin raids … were nearly always heralded in this country by the crowing of pheasants, and the sensitiveness of this species to distant sounds was frequently a subject of comment. There seems no reason to suppose that pheasants have keener powers of hearing than men; it appears more probable that these birds are alarmed by the sudden quivering of the trees, on which they happen to be perched, at the time of an explosion … During the first Zeppelin raid in January 1915, pheasants … thirty-five to forty miles from the area over which the Zeppelins flew, shrieked themselves hoarse. In one of the early battles in the North Sea … Gamekeepers on the east coast used to say that they always knew when enemy raids had commenced, ‘for the pheasants call us day and night’.
On the Western Front, a starling learned to imitate the whistle that warned of enemy aeroplanes. One artillery officer wrote, “It was great fun to see everyone diving for cover, and I was nearly deceived myself one day.” A gun commander wrote of an owl, “The beastly bird learnt to imitate the alarm whistle to a nicety; on several occasions he turned me out in pyjamas and, when the crew had manned the gun, gave vent to a decided chuckle.” See Onlookers.
(Joy Damousi, Deborah Tout-Smith, and Bart Ziino, eds., Museums, History and the Intimate Experience of the Great War, 2020.)
The Peirce-Putnam Paradox
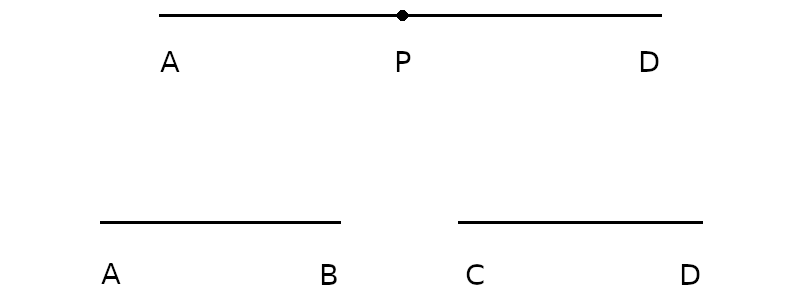
Divide line interval AD at point P and separate the halves by a short distance.
What’s happened to point P? Did it become point B or C? It seems wrong to say that it’s neither of these, or that it’s only one of them.
But if the single point P has “become” the two points B and C, how can we say it was a dimensionless object?
(Hilary Putnam, “Peirce’s Continuum,” in Kenneth Laine Ketner, ed., Peirce and Contemporary Thought: Philosophical Inquiries, 1995, via Piotr Łukowski, Paradoxes, 2011.)