A digital animation by Catherine Leah Palmer.
Science & Math
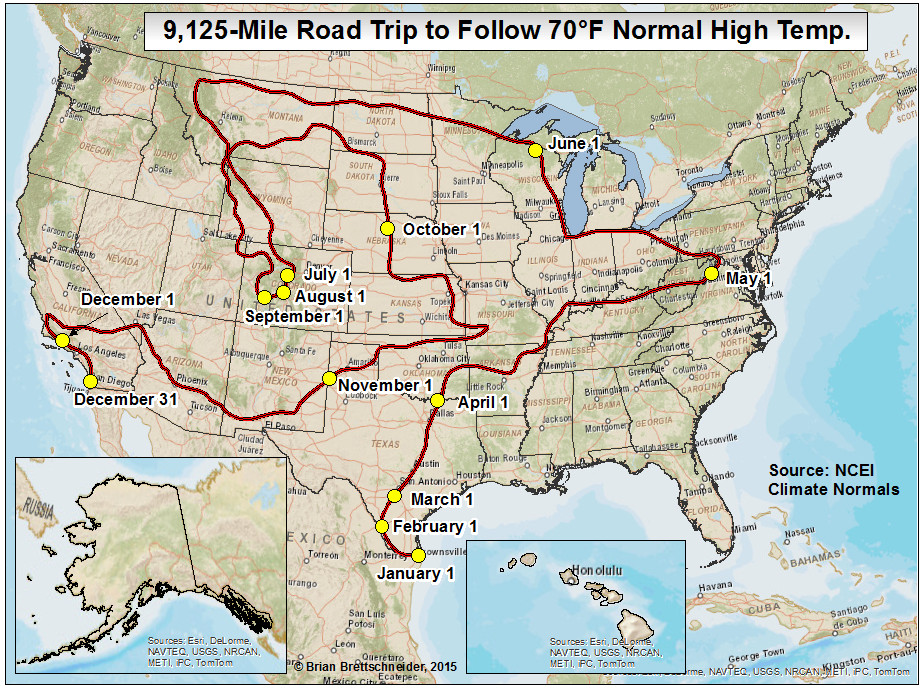
A Cool Journey
Reddit user Climatologist49 offered this map in 2015: By starting in Brownsville, Texas, on New Year’s Day and arriving at each waypoint on the day indicated, a heat-sensitive tourist could travel 9,125 miles (14,685 km) through the contiguous United States while experiencing a constant normal daytime high temperature of 70°F (21°C). They’d arrive in San Diego on New Year’s Eve. I wonder how much these temperatures have changed in eight years.
Crash Course

What is this? It’s the history of 800 successive unsteered bicycles, each traveling from left to right until it falls over. Caltech computer scientist Matthew Cook modeled the behavior in 2004, hoping to learn how we balance, steer, and correct our paths on two wheels. He found that just two artificial neurons were enough to control a bicycle competently — the system even learned to thread a series of waypoints:
(Matthew Cook, “It Takes Two Neurons to Ride a Bicycle,” Demonstration at NIPS 4, 2004.) (Thanks, Dan.)
Insight
One other interesting item from Paul Halmos’ Problems for Mathematicians, Young and Old (1991): Pick a point in the first quadrant and draw a downward-sloping line through it. This line makes a triangle with the coordinate axes. At what angle should we set the line to minimize the area of the triangle?
This problem yields to calculus, but there’s a simple geometric solution. Reflect the axes through the point to make a box:
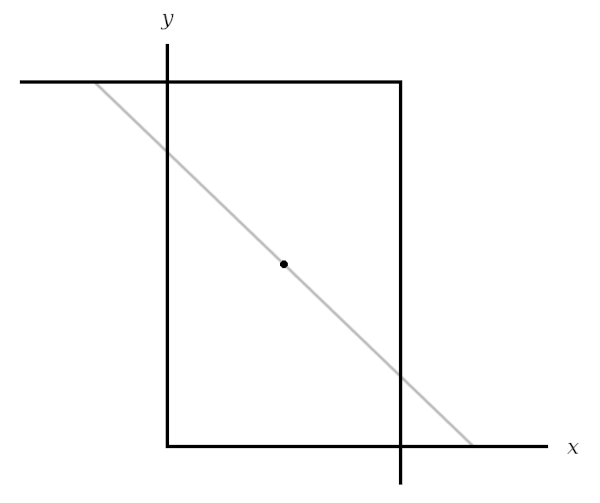
Now as we swivel our line through the point, it defines two triangles, one against each set of axes. The area of the combined triangles is equal to or greater than the area of the box. So, intuitively, it reaches a minimum just as the swiveling line becomes a diagonal of the box. That’s the answer.
Recombination
Harry Mathews assembled lines from 14 existing sonnets to make a new one:
Shall I compare thee to a summer’s day
And dig deep trenches in thy beauty’s field?
Why lov’st thou that which thou receiv’st not gladly,
Bare ruin’d choirs where late the sweet birds sang?
Anon permit the basest clouds to ride
And do whate’er thou wilt, swift-footed Time:
Nor Mars his sword, nor war’s quick fire, shall burn
Even such a beauty as you master now.
Love’s not Time’s fool, though rosy lips and cheeks
(When other petty griefs have done their spite,
And heavily) from woe to woe tell o’er
That Time will come and take my love away;
For thy sweet love remembered such wealth brings
As any she belied beyond compare.
“This new poem sheds light on the structure and movement of the Shakespearean sonnet,” he wrote. “Nothing any longer can be taken for granted; every word has become a banana peel.”
(Harry Mathews, “Mathews’s Algorithm,” in Warren F. Motte, ed., Oulipo: A Primer of Potential Literature, 1998.)
Misc

- The Dutch word for cease-fire negotiations is wapenstilstandsonderhandelingen.
- Rearrange the letters in ONE THOUSAND KILOS and you get OH, SOUNDS LIKE A TON! (Hans-Peter Reich)
- 1167882 + 3211682 = 116788321168
- The Irish for chess, ficheall, derives from the Old Irish fidchell, “wood intelligence.”
- “Life is a school of probability.” — Walter Bagehot
A tiny detail that I hope is true: In Time in World History (2019), historian Peter Stearns writes that before watches became affordable, some European soldiers “took their own roosters with them so they would wake up on time.”
Round Numbers
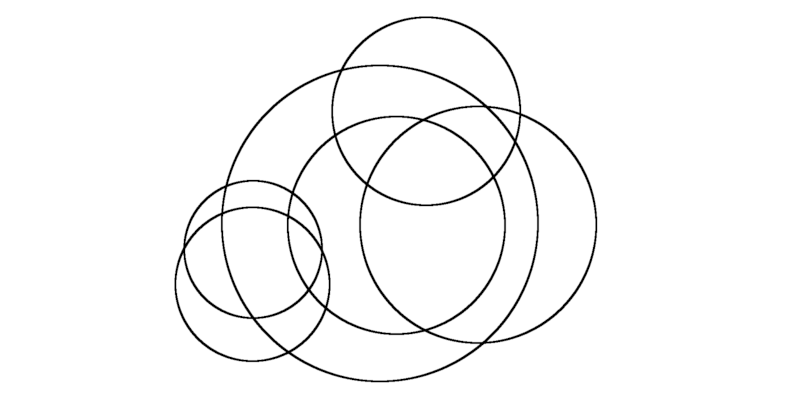
A bit more on map coloring: Suppose a map consists of a number of overlapping circles, like this, so that the borders of each “country” are all arcs of circles. How many colors would we need to color this map, again with the proviso that no two countries that share a border will receive the same color?
Here we need only two. Each country occupies the interior of some number of circles. If that number is even, color the country white; if odd, black. Crossing a border always changes the number by 1, so each border will divide countries of opposite colors.
From Paul R. Halmos, Problems for Mathematicians, Young and Old, 1991.
The Region Beta Paradox
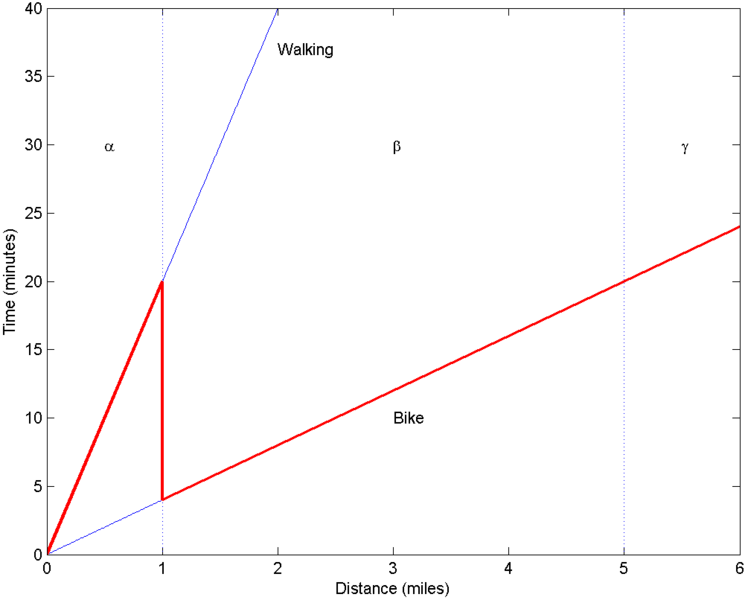
Suppose you decide that you’ll walk (at 3 mph) to any destination that’s within a mile of your house, and bike (at 15 mph) to any destination that’s farther away. That’s a reasonable choice, but it has a surprising result: You’ll actually arrive more quickly at moderately distant points (1 to 5 miles away) than at most points closer to home (less than 1 mile away).
Psychologist Daniel Gilbert uses this example to illustrate a phenomenon in our reactions to stressful events: Sometimes we’ll recover more quickly from particularly distressing experiences because they’re strong enough to invoke defense processes that attentuate stress.
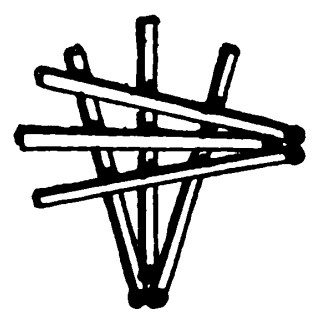
The Six Submarines
A puzzle by Henry Dudeney:
If five submarines, sunk on the same day, all went down at the same spot where another had previously been sunk, how might they all lie at rest so that every one of the six U-boats should touch every other one? To simplify we will say, place six ordinary wooden matches so that every match shall touch every other match. No bending or breaking allowed.
Boo!

J.H. Brown’s 1864 book Spectropia: Or, Surprising Spectral Illusions promises to show “ghosts everywhere, and of any colour.” It accomplishes this by relying on two simple principles: persistence of vision and complementary colors. Readers are directed to stare at any of the figures for 15 seconds and then turn their eyes to a white surface (or the sky); “the spectre will soon begin to make its appearance, increasing in intensity, and then gradually vanishing,” in the color complementary to that of the stimulus.