In David Hayes and Tatiana Shubin’s Mathematical Adventures (2004), University of California-Davis mathematician Don Chakerian describes a method used in antiquity for solving an equation in one unknown. He illustrates it with a problem from Daboll’s Schoolmaster’s Assistant (1800):
A, B, and C built a house which cost $500, of which A paid a certain sum, B paid 10 dollars more than A, and C paid as much as A and B both; how much did each man pay?
We’ll make two guesses as to how much A paid, check them, and plug the “errors” into a formula to get the right answer. First, suppose A pays $80. That means that B pays $90 and C pays $170, giving a total of $340. That’s 500 – 340 = $160 short of the goal, so our guess of $80 yields an “error” of $160. As a second guess, suppose that A pays $150. In that case B pays $160, C pays $310, and the total is now $620. This time the “error” is 500 – 620 = -$120. The false position method (technically here the double false position method) offers this formula for finding the right answer:
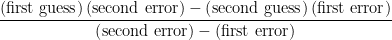
In this case it gives

When A pays $120 then B pays $130, C pays 250, and together they pay $500, so this solution works.
This is hardly the most efficient way to solve a simple linear equation given the tools we have today, but it served for centuries. In his Ground of Artes of 1542, Robert Recorde offered a rule:
Gesse at this woorke as happe doth leade.
By chaunce to truthe you may procede.
And firste woorke by the question,
Although no truthe therein be don.
Suche falsehode is so good a grounde,
That truth by it will soone be founde.
From many bate to many mo,
From to fewe take to fewe also.
With to much ioyne to fewe againe,
To to fewe adde to manye plaine.
In crossewaies multiplye contrary kinde,
All truthe by falsehode for to fynde.



