Kenya and Uganda both lie on the equator, so the sun rises around 6 a.m. and sets around 6 p.m. throughout the year. Given such a reliable natural timekeeper, it’s customary to reckon time by counting hours of light or hours of darkness: 7 a.m. is called 1 o’clock (saa moja, or one hour of light), and 11 a.m. is called 5 o’clock (saa tano) (moja means 1 and tano 5 in Swahili). Similarly, 7 p.m. is called 1 o’clock (one hour of darkness), and 11 p.m. is 5 o’clock.

Confusingly for newcomers, clocks themselves are set to Western time, but they’re read aloud in “Swahili time.” Increasingly, though, Africans are simply conforming to Western conventions.

Take any two rational numbers whose product is 2, and add 2 to each. The results are the legs of a right triangle with rational sides.

For example, 13/17 × 34/13 = 2. If we add 2 to each of these we get 47/17 and 60/13 or, clearing fractions, 611 and 1020. The hypotenuse is 1189.

Because (z + 2)² + [(2/z) + 2]² = [z + 2 + (2/z)]², if z is rational, so are all three sides.

(R.S. Williamson, “A Formula for Rational Right-Angle Triangles,” Mathematical Gazette 37:322 [December 1953], 289-290, via Claudi Alsina and Roger B. Nelsen, Icons of Mathematics, 2011.)

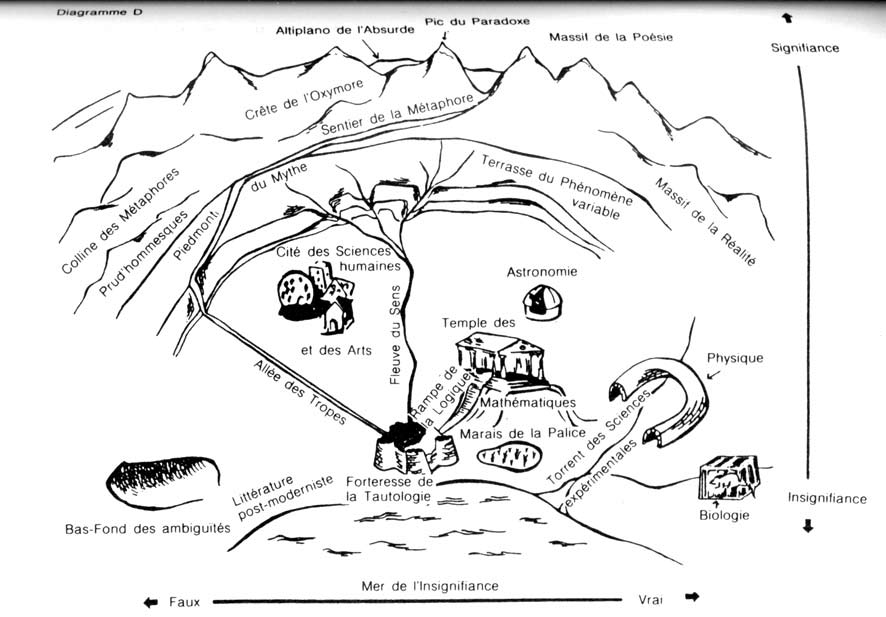
In To Predict Is Not to Explain, mathematician René Thom describes a lunch at which psychoanalyst Jacques Lacan responded strongly to his statement that “Truth is not limited by falsity, but by insignificance.” Thom described the idea later in a drawing:

At the base, one finds an ocean, the Sea of the Insignificance. On the continent, Truth is on one side, Falsehood on the other. They are separated by a river, the River of Discernment. It is indeed the faculty of discernment that separates truth from falsehood. It’s Aristotle’s notion: the capacity for contradiction. It’s what separates us from animals: When information is received by them, it’s instantly accepted and it triggers obedience to its message. Human beings, however, have the capacity to withdraw and to question its veracity.

Following the banks of this river, which flows into the Sea of Insignificance, one travels along a coastline that is slightly concave: Situated at one end is the Slough of Ambiguity; at the other end is the Swamp of La Palice. At the head of the river delta, one sees the Stronghold of Tautology: That’s the stronghold of the logicians. One climbs a rampart towards a small temple, a kind of Parthenon: that’s Mathematics.

To the right, one finds the Exact Sciences: Up in the mountains that surround the bay is Astronomy, with an observatory topping its temple; at the far right stand the giant machines of Physics, the accelerator rings at CERN; the animals in their cages indicate the laboratories of Biology. Out of all this, there emerges a creek that feeds into the Torrent of Experimental Science, which flows into the Sea of Insignificance.

To the left is a wide path climbing towards the north west, up to the City of Human Arts and Sciences. Continuing along it one comes to the foothills of Myth. We’ve entered the kingdom of anthropology. Up at the top is the High Plateau of the Absurd. The spine signifies the loss of the ability to discern contraries, something like an excess of universal understanding which makes life impossible.

He explains the central idea in more detail starting on page 173 of the PDF linked above. “It’s something I’ve done to amuse myself, but it reflects something real, I think: The Logos, the possibility of representation by language, only comes into play for humanity in a rather limited number of situations … [O]ne begins to manufacture linguistic entities which do not correspond to real things. … That’s where the River of Discernment runs into the Fortress of Tautology, into the sewers. It’s become invisible, but at the surface it can smell pretty bad.”

How I need a drink, alcoholic of course, after the tough chapters involving quantum mechanics!

That sentence is often offered as a mnemonic for pi — if we count the letters in each word we get 3.14159265358979. But systems like this are a bit treacherous: The mnemonic presents a memorable idea, but that’s of no value unless you can always recall exactly the right words to express it.

In 1996 Princeton mathematician John Horton Conway suggested that a better way is to focus on the sound and rhythm of the spoken digits themselves, arranging them into groups based on “rhymes” and “alliteration”:

                        _     _   _
            3 point  1415  9265  35
                     ^ ^
             _ _  _ _    _ _   __
            8979  3238  4626  4338   3279
              **  **^^          ^^   ****
             .   _    _   __   _    _      _ . _ .
       502 884  197 169  399 375  105 820  974 944
        ^  ^                       ^  ^
                59230 78164
                 _     _    _    _
              0628  6208  998  6280
               ^^   ^^         ^^
             .. _  .._
             34825 34211 70679
                         ^  ^

He walks through the first 100 digits here.

“I have often maintained that any person of normal intelligence can memorize 50 places in half-an-hour, and often been challenged by people who think THEY won’t be able to, and have then promptly proved them wrong,” he writes. “On such occasions, they are usually easily persuaded to go on up to 100 places in the next half-hour.”

“Anyone who does this should note that the initial process of ‘getting them in’ is quite easy; but that the digits won’t then ‘stick’ for a long time unless one recites them a dozen or more times in the first day, half-a-dozen times per day thereafter for about a week, a few times a week for the next month or so, and every now and then thereafter.” But then, with the occasional brushing up, you’ll know pi to 100 places!

Western Michigan University mathematician Allen J. Schwenk discovered this oddity in 2000: Consider three fair six-sided dice of different colors, marked with the following numbers:

• Red: 2, 2, 2, 11, 11, 14
• Blue: 0, 3, 3, 12, 12, 12
• Green: 1, 1, 1, 13, 13, 13

Now:

• The red die beats the green die 7/12 of the time.
• The blue die beats the red die 7/12 of the time.
• The green die beats the blue die 7/12 of the time.

We’ve seen that before. But look at this:

• A pair of green dice beats a pair of red dice 693/1296 of the time.
• A pair of red dice beats a pair of blue dice 675/1296 of the time.
• A pair of blue dice beats a pair of green dice 693/1296 of the time.

The favored color in each pairing has changed! Schwenk writes, “I call this a perverse reversal.”

(And a bonus: It turns out that a pair of Schwenk dice of any one color is an even match against a mixed pair of the other two colors.)

(Allen J. Schwenk, “Beware of Geeks Bearing Grifts,” Math Horizons 7:4 [April 2000], 10-13, via Jennifer Beineke and Lowell Beineke, “Some ABCs of Graphs and Games,” in Jennifer Beineke and Jason Rosenhouse, eds., The Mathematics of Various Entertaining Subjects, 2016.)