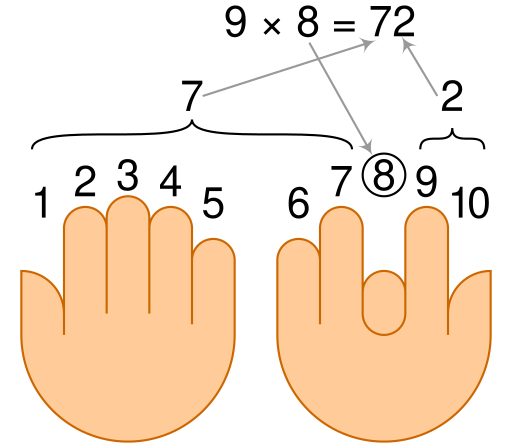
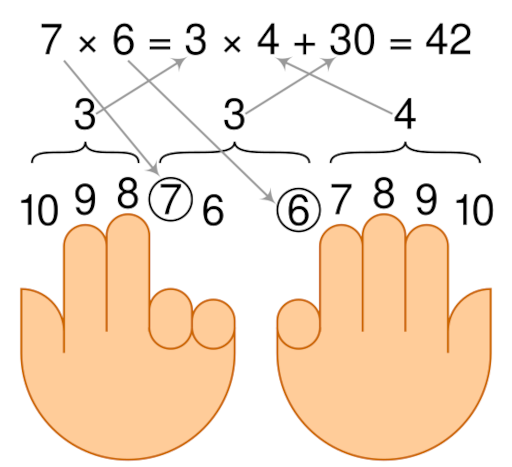
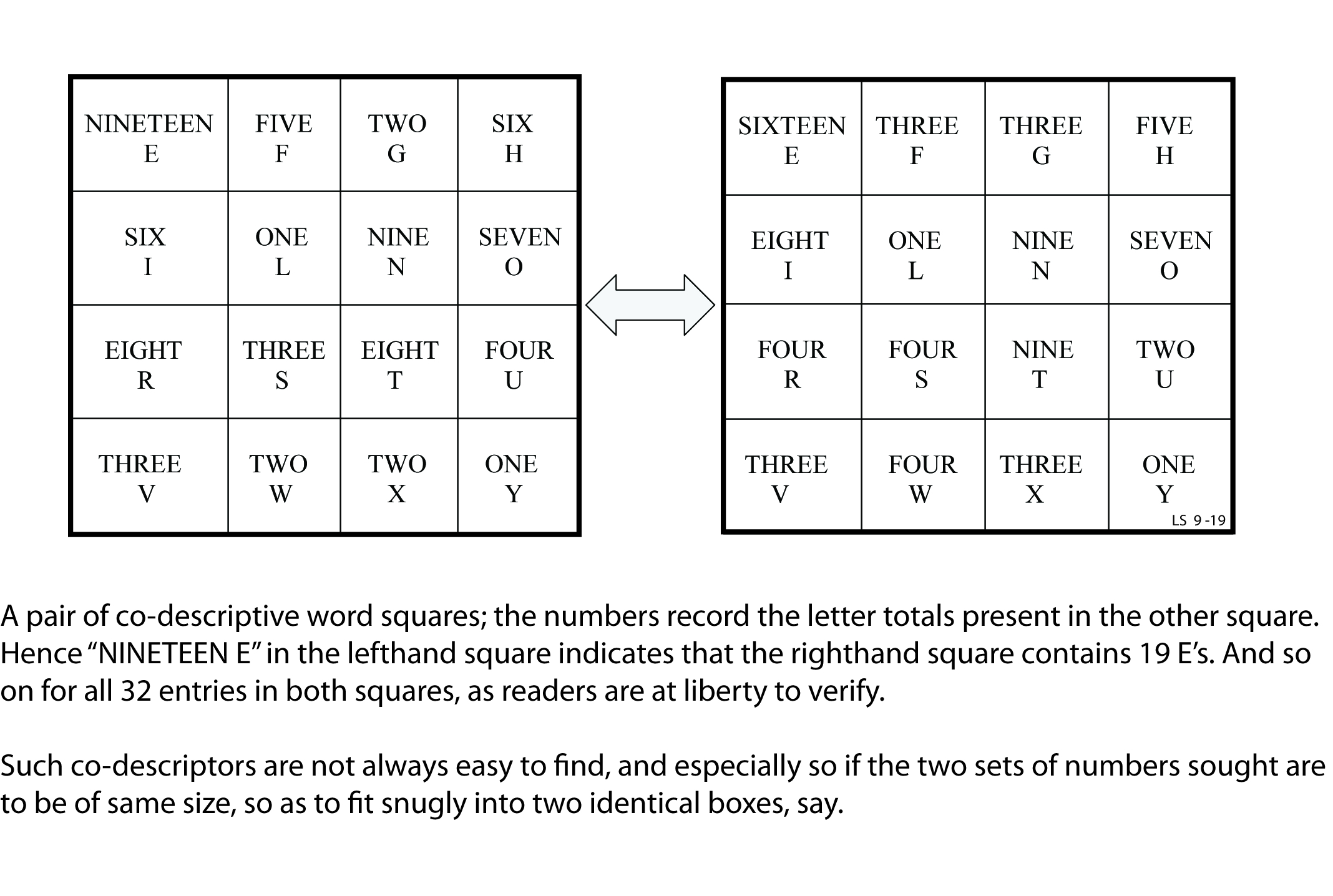
Into an empty vase drop balls numbered 1 to 10. Remove ball 1. Add balls numbered 11 to 20. Remove ball 2. Continue in this way, spending half an hour on the first transaction, 15 minutes on the next, and so on. After one hour all the transactions will be finished.
Obviously, in the end the vase will contain infinitely many balls, since with each step more balls have been added than removed.
But, equally obviously, after an hour the vase will be empty — since the time of each ball’s removal is known.