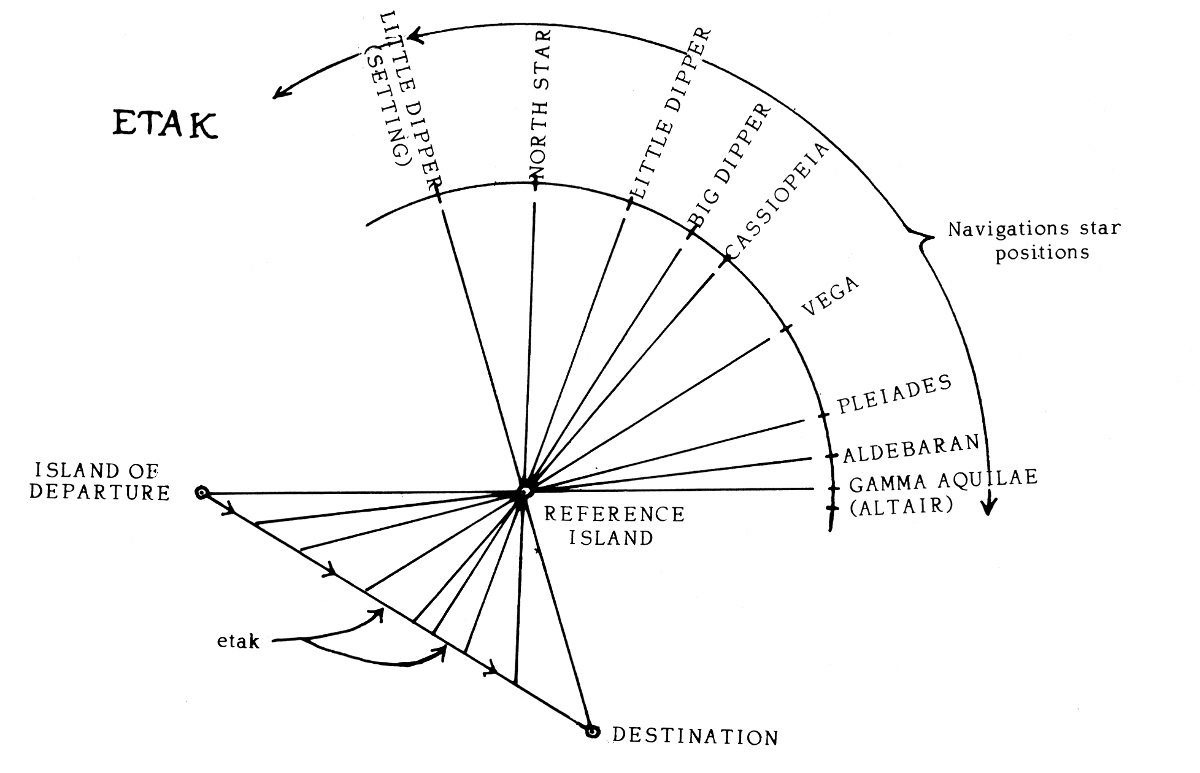
Navigators from the Poluwat atoll of Micronesia find their way among islands by appealing to parallax — a reference island at one side of their course will appear to pass beneath a succession of stars:
The star bearings of the reference island from both the starting and ending points of the trip are known, since on another occasion the reference island may itself become a destination. In between there are other navigation star positions under which the reference island will pass as it ‘moves’ backwards. Its passage under each of these stars marks the end of one etak and the beginning of another. Thus the number of star positions which lie between the bearing of the reference island as seen from the island of origin and its bearing as seen from the island of destination determine the number of etak, which can here be called segments, into which the voyage is conceptually divided. When the navigator envisions in his mind’s eye that the reference island is passing under a particular star he notes that a certain number of segments have completed and a certain proportion of the voyage has therefore been accomplished.
This is a dynamic model: Where Western navigators think of a vessel moving among stationary islands, the Poluwatese find it more natural to think of the canoe as stationary and the islands as moving around it. “Etak is perfectly adapted for its use by navigators who have no instruments, charts, or even a dry place in which to spread a chart if they had one,” writes Stephen D. Thomas in The Last Navigator. “Etak allows the Micronesian navigator to process all his information — course, speed, current drift, and so on — through a single, sea-level perspective.”
(Thomas Gladwin, East Is a Big Bird: Navigation and Logic on Puluwat Atoll, 1970, quoted in Lorenzo Magnani, Philosophy and Geometry, 2001.)