Thomas Jefferson coined the word pedicure (at least in the sense “a person responsible for pedicure”).
His memorandum book for February 7, 1785, reads “P[ai]d La Forest, pedicure 12f.”
(Thanks, Joseph.)

Thomas Jefferson coined the word pedicure (at least in the sense “a person responsible for pedicure”).
His memorandum book for February 7, 1785, reads “P[ai]d La Forest, pedicure 12f.”
(Thanks, Joseph.)
The sum of 2k – 4
From one to thirteen plus a score,
Over eleven,
Plus eighteen times seven,
Equals six cubed and not a bit more.
(Will Nediger, “Can Math Limericks Survive?”, Word Ways 37:3 [August 2004], 238.)
A tromino is a domino of three panels in a row, sized to cover three successive orthogonal squares of a checkerboard.
A monomino covers one square.
Is it possible to cover an 8×8 checkerboard with 21 trominoes and 1 monomino?
Prescott, press my Ascot waistcoat —
Let’s not risk it
Just to whisk it:
Yes, my Ascot waistcoat, Prescott.
Worn subfusc, it’s
Cool and dusk: it
Might be grass-cut
But it’s Ascot,
And it fits me like a gasket —
Ascot is the waistcoat, Prescott!
Please get
Off the spot of grease. Get
Going, Prescott —
Where’s that waistcoat?
It’s no task at
All, an Ascot:
Easy as to clean a musket
Or to dust an ivory tusk. It
Doesn’t take a lot of fuss. Get
To it, Prescott,
Since I ask it:
We can’t risk it —
Let’s not whisk it.
That’s the waistcoat;
Thank you, Prescott.
— David McCord
“Let us take a typical case. A gentleman and his wife, calling on friends, find them not at home. The gentleman decides to leave a note of regret couched in a few well-chosen words, and the first thing he knows he is involved in this:
We would have liked to have found you in.
“Reading it over, the gentleman is assailed by the suspicion that he has too many ‘haves,’ and that the whole business has somehow been put too far into the past. … He takes an envelope out of his pocket and grimly makes a list of all the possible combinations, thus getting:”
“If he has married the right kind of woman, she will hastily scratch a brief word on a calling card, shove it under the door, and drag her husband away.”
— James Thurber, “Ladies’ and Gentlemen’s Guide to Modern English Usage,” 1931
In his Canterbury Puzzles of 1907, Henry Dudeney posed a now-famous challenge: How can you cut an equilateral triangle into four pieces that can be reassembled to form a perfect square?
Dudeney’s beautiful solution was accompanied by a rather involved geometric derivation. It seems unlikely that he worked this out laboriously in approaching an answer to the problem, but how then did he reach it?
Here’s one possibility: If a strip of squares is draped adroitly over a strip of triangles, their intersection forms a wordless proof of the task’s feasibility:
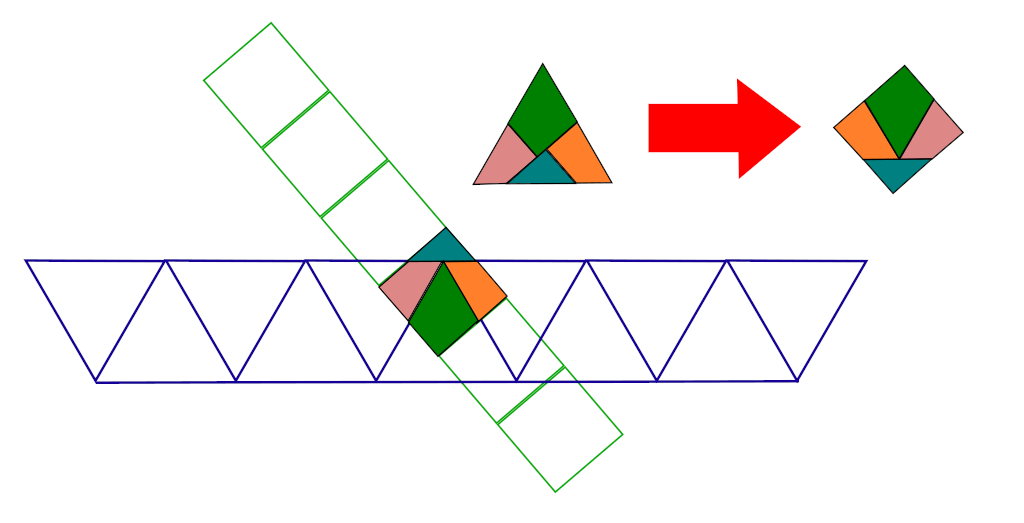
Whether that was Dudeney’s path to the solution is not known, but it appears at least plausible.
Unusual names of racehorses, collected by Paul Dickson in What’s in a Name?, 1996:
After the Jockey Club rejected several names for one filly in the 1960s, the exasperated owner wrote “You Name It” on the application form. “We did,” said registrar Alfred Garcia. “We approved the name You Name It, and I think she turned out to be a winner, too.”
This race, run at Monmouth Park in 2010, seems to take on a deeper significance near the end:
The recipe for “Groper, Head and Shoulders Boiled” in Mrs. Beeton’s Everyday Cookery (1923) concludes with the warning “Great care should be taken of the immense gelatinous lips, as these are considered the best part.” In 1948 New Statesman challenged its readers to invent a recipe with a more disgusting last line. L.G. Udall obliged:
GRILLED GORILLA’S FOOT
One foot will suffice for each person.
First shave the upper part of the foot and wash in warm water. With a gimlet (for preference as the skin is very hard) bore a number of holes through the thick skin of the under part of the foot. Grease liberally with lard. Grill slowly for about twenty minutes with the under surface downwards. Then turn the foot over and continue to grill steadily. From time to time place a fork on the foot. When it is quite done it will be found that the toes will curl up firmly over the fork, so that it can be lifted up and put on a hot plate. Leave the fork in the toes and serve immediately.
The other winners are here.

The joists in the tower in which Montaigne wrote his Essays are inscribed with his favorite quotations from Greek and Latin authors, many of which appear in his writings: “It is not so much things that torment man, as the opinions he has of things.” “Every reasoning has its contrary.” “Wind swells bladders, opinion swells men.”
He wrote, “The room pleases me because it is somewhat difficult of access, and retired, as much on account of the utility of the exercise, as because I there avoid the crowd. Here is my seat, my place, my rest. I try to make it purely my own, and to free this single corner from conjugal, filial, and civil community.”
The numbers in the diagram below correspond to this table in the German Wikipedia. English translations are here.
In large Latin letters on the central rafter are the words “I DO NOT UNDERSTAND. I PAUSE. I EXAMINE.”