The joists in the tower in which Montaigne wrote his Essays are inscribed with his favorite quotations from Greek and Latin authors, many of which appear in his writings: “It is not so much things that torment man, as the opinions he has of things.” “Every reasoning has its contrary.” “Wind swells bladders, opinion swells men.”
He wrote, “The room pleases me because it is somewhat difficult of access, and retired, as much on account of the utility of the exercise, as because I there avoid the crowd. Here is my seat, my place, my rest. I try to make it purely my own, and to free this single corner from conjugal, filial, and civil community.”
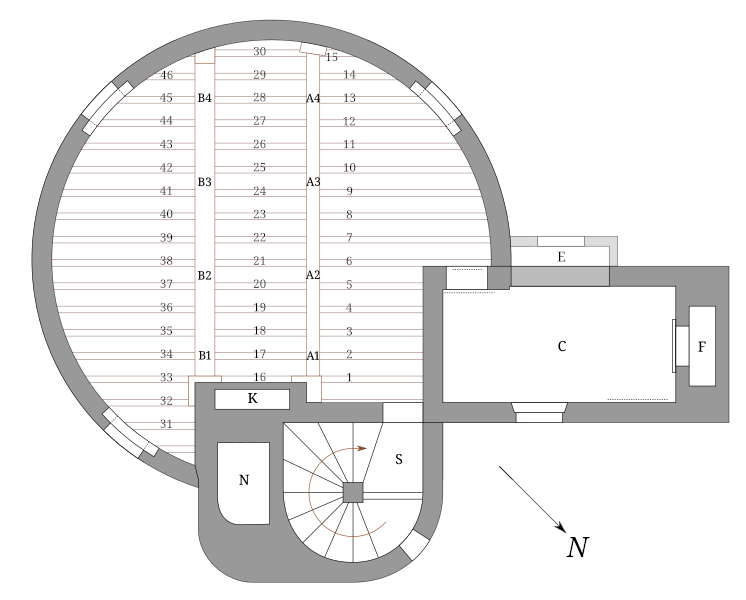
The numbers in the diagram below correspond to this table in the German Wikipedia. English translations are here.
In large Latin letters on the central rafter are the words “I DO NOT UNDERSTAND. I PAUSE. I EXAMINE.”