A problem from the Sixth International Mathematical Olympiad, 1964: Seventeen people correspond by mail, each with all the rest. They discuss only three topics, and each pair of correspondents addresses only one of these. Prove that there are at least three people who write to each other about the same topic.
Undefined
In 1941, biochemist Alexander Todd visited the Defence Research Establishment at Porton to see a demonstration of a new chemical weapon to be used against tanks. Afterward, “I proceeded to the bar where — believe it or not — there was a white-coated barman who was not only serving drinks but also cigarettes!”
I hastened forward and rather timidly said ‘Can I have some cigarettes?’
‘What’s your rank?’ was the slightly unexpected reply.
‘I am afraid I haven’t got one,’ I answered.
‘Nonsense — everyone who comes here has a rank.’
‘I’m sorry but I just don’t have one.’
‘Now that puts me in a spot,’ said the barman, ‘for orders about cigarettes in this camp are clear — twenty for officers and ten for other ranks. Tell me what exactly are you?’
Now I really wanted those cigarettes so I drew myself up and said ‘I am the Professor of Chemistry at Manchester University.’
The barman contemplated me for about thirty seconds and then said ‘I’ll give you five.’
Todd added, “Since that day I have had few illusions about the important of professors!”
(From his 1983 autobiography, A Time to Remember.)
Black and White
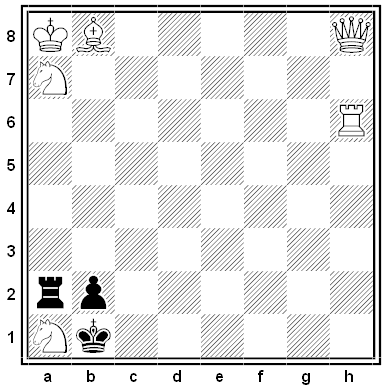
A curious chess problem from the Jamaica Gleaner. White has just moved. If he retracts this move, he can play another that forces Black to checkmate White immediately. What move does he retract?
Home Primes
Pick an integer greater than 1 (say, 14). List its prime factors in order from smallest to greatest (2 7), and then “paste” those factors together to create a new number (27). Apply the same procedure to that number, and keep going until you reach a prime number:
27 = 3 × 3 × 3 → 333
333 = 3 × 3 × 37 → 3337
3337 = 47 × 71 → 4771
4771 = 13 × 367 → 13367
13367 is prime, so that’s the end of that sequence: 13367 is the home prime of 14.
A home prime should exist for every positive integer, but finding them can be stupendously hard. The sequence starting with 49 has been pressed through 118 steps now without producing a prime; the search continues. Details are maintained at Patrick De Geest’s website World of Numbers.
Unquote
“We become innocent when we are unfortunate.” — La Fontaine
Podcast Episode 325: Lateral Thinking Puzzles

Here are eight new lateral thinking puzzles — play along with us as we try to untangle some perplexing situations using yes-or-no questions.
De Bruijn’s Theorem
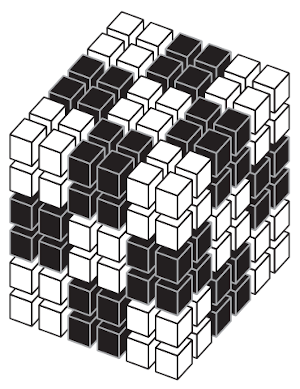
At age 7, F.W. de Bruijn found himself unable to pack a box measuring 6 × 6 × 6 quite completely with bricks measuring 1 × 2 × 4. The box had volume 216, so it might be expected to accommodate exactly 27 bricks, but he found there was no way to pack more than 26.
He mentioned this to his father, who happened to be mathematician Nicolaas Govert de Bruijn, and Nicolaas found that a “harmonic brick” (one in which the length of each side is a multiple of the next smaller side length) can be packed efficiently only into a box whose dimensions are multiples of the brick’s dimensions.
This can seen intuitively by imagining the 6 × 6 × 6 box filled with small colored cubes as shown here. No matter where it’s placed, each 1 × 2 × 4 brick must now displace an equal number of white and black cubes. But the box contains 112 white cubes and 104 black ones. So the task is impossible.
(Nicholas G. de Bruijn, “Filling Boxes With Bricks,” American Mathematical Monthly 76:1 [1969], 37-40.)
Two Christmas Quizzes
King William’s College, on the Isle of Man, has posted the 2020 edition of “The World’s Most Difficult Quiz,” with its customary epigraph, Scire ubi aliquid invenire possis ea demum maxima pars eruditionis est (“The greatest part of knowledge is knowing where to find something”). Answers will be posted on January 20; as usual, MetaFilter is maintaining a Google spreadsheet of communal guesses.
And the Royal Statistical Society has posted its own 2020 Christmas quiz, which it describes as “brain-melting.” “You’ll need a combination of general knowledge, logic, and lateral thinking skills to successfully crack these puzzles — but as always, no specialist mathematical knowledge is required.” Solutions are due by the end of January; the top prize is £150 in Wiley book vouchers.
Fortunate Numbers
Multiply the first n prime numbers:
2 × 3 × 5 × 7 × 11 × 13 = 30030
Now find the smallest integer greater than 1 that will produce a prime number when it’s added to that product. In this example it’s 17:
30030 + 17 = 30047,
which is prime. This makes 17 a Fortunate number, named for Reo Fortune, the social anthropologist who first studied this. The first few Fortunate numbers are
3, 5, 7, 13, 23, 17, 19, 23, 37, 61, 67, 61, 71, 47, 107, 59, 61, 109, 89, 103, 79, 151 …
Are all Fortunate numbers prime? Fortune conjectured so, but whether it’s true remains an open problem.
The Coinage Shield

The “tails” sides of British coins less than £1 can be arranged to depict the Royal Shield from the monarch’s coat of arms.
The full Royal Shield appears on the £1 coin.
