ingeminate
v. to utter (a sound) twice or oftener
stoundmeal
adv. at intervals; from time to time
anamnesis
n. the recalling of things past
concinnate
v. to arrange or blend together skillfully
ingeminate
v. to utter (a sound) twice or oftener
stoundmeal
adv. at intervals; from time to time
anamnesis
n. the recalling of things past
concinnate
v. to arrange or blend together skillfully
New York zoning rules limit the height of skyscrapers, so Oiio Studio has proposed an innovative solution: Bend the building into a horseshoe. Designer Ioannis Oikonomou’s “Big Bend” building would be the “longest” building in the world, at 4,000 feet, but it would stand only 200 feet taller than One World Trade Center, currently the city’s tallest building.
“If we manage to bend our structure instead of bending the zoning rules of New York we would be able to create one of the most prestigious buildings in Manhattan,” the firm says in its building proposal. “The Big Bend can become a modest architectural solution to the height limitations of Manhattan.”
Whether that can be done remains to be seen. The project remains in the proposal stage.
In 1882 Anton Chekhov published eight “Questions Posed by a Mad Mathematician.” Here are the first three:
The full list appears in The Undiscovered Chekhov, translated by Peter Constantine (1998). No answers are provided.
At the 1961 Solvay conference on physics, Abdus Salam overheard this conversation between Richard Feynman and Paul Dirac:
Feynman extended his hand towards Dirac and said: ‘I am Feynman.’ It was clear from his tone that it was the first time they were meeting. Dirac extended his hand and said: ‘I am Dirac.’ There was silence, which from Feynman was rather remarkable. Then Feynman, like a schoolboy in the presence of a Master, said to Dirac: ‘It must have felt good to have invented that equation.’ And Dirac said: ‘But that was a long time ago.’ Silence again. To break this, Dirac asked Feynman: ‘What are you yourself working on?’ Feynman said: ‘Meson theories’ and Dirac said: ‘Are you trying to invent a similar equation?’ Feynman said: ‘That would be very difficult.’ And Dirac, in an anxious voice, said: ‘But one must try.’
“At that point the conversation finished because the meeting had started.”
(Abdus Salam, “Physics and the Excellences of the Life It Brings,” in Ideals and Realities: Selected Essays of Abdus Salam, 1987.)
In 1890, French inventor Louis Le Prince vanished just as he was preparing to debut his early motion pictures. He was never seen again. In this week’s episode of the Futility Closet podcast we’ll consider the possible causes of Le Prince’s disappearance and his place in the history of cinema.
We’ll also reflect on a murderous lawyer and puzzle over the vagaries of snake milking.
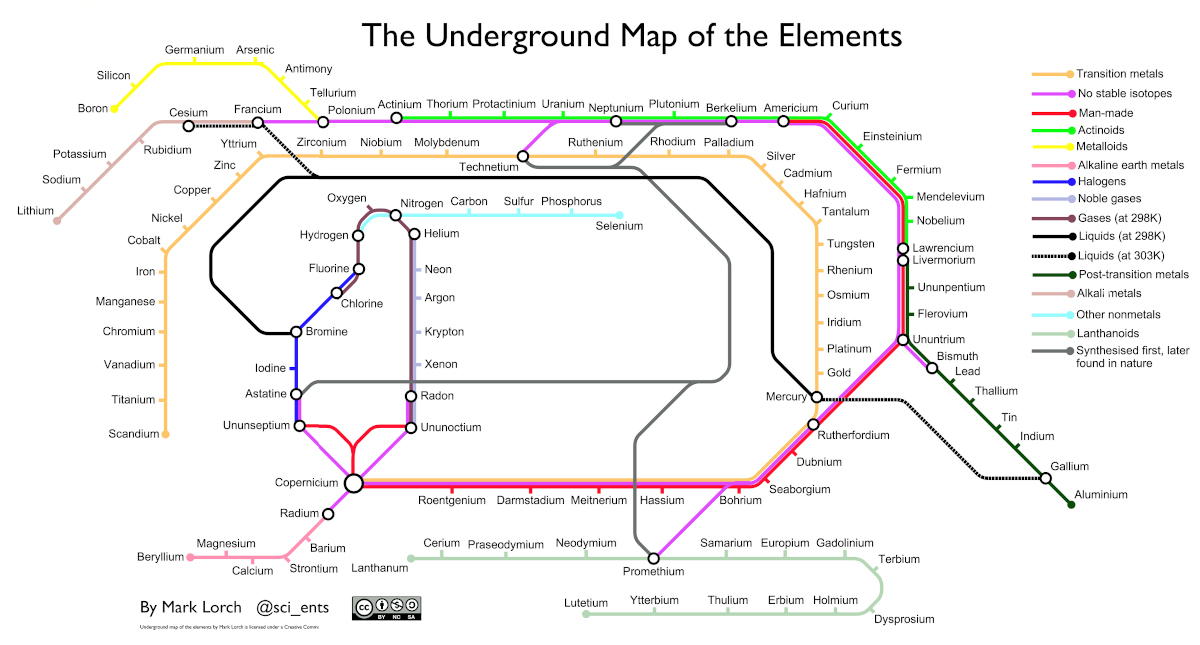
This is brilliant: University of Hull chemist Mark Lorch has combined the periodic table with London’s classic Tube map to create an Underground Map of the Elements.
“My son loves trains. So I came up with a train related twist to an inspection of the periodic table. We sat and cut up a copy of the table and then rearranged each element as a ‘station’ on an underground rail system. Each line represents a characteristic shared by the elements on that line.”
More details at the Guardian and at Chemistry Blog.

Henry Irving, actor-manager at the West End’s Lyceum Theatre, was powerful, imperious, self-absorbed, and manipulative — qualities that made a fateful impression on his theater’s business manager, Bram Stoker. University of California historian Louis S. Warren writes:
Scholars have long agreed that keys to the Dracula tale’s origin and meaning lie in the manager’s relationship with Irving in the 1880s. … There is virtual unanimity on the point that the figure of Dracula — which Stoker began to write notes for in 1890 — was inspired by Henry Irving himself. … Stoker’s numerous descriptions of Irving correspond so closely to his rendering of the fictional count that contemporaries commented on the resemblance. … But Bram Stoker also internalized the fear and animosity his employer inspired in him, making them the foundations of his gothic fiction.
The two worked together for 28 years. Warren writes, “Understandably, Stoker felt most secure when Irving took an interest in him personally, as he did in the early 1880s; and he became anxious and jealous when Irving turned his gaze to other men, as he did by 1885.”
One contemporary wrote, “To Bram, Irving is as a god, and can do no wrong.”
(Louis S. Warren, “Buffalo Bill Meets Dracula: William F. Cody, Bram Stoker, and the Frontiers of Racial Decay,” American Historical Review 107:4 [October 2002], 1124–1157.)
Hi, all. My libraries are beginning to open now, so I can start writing again. Thanks for your patience!
Greg

Marooned in Florida in 1528, four Spanish colonists made an extraordinary journey across the unexplored continent. Their experiences changed their conception of the New World and its people. In this week’s episode of the Futility Closet podcast we’ll describe the remarkable odyssey of Álvar Núñez Cabeza de Vaca and his reformed perspective on the Spanish conquest.
We’ll also copy the Mona Lisa and puzzle over a deficient pinball machine.

The only dog ever enlisted in the Royal Navy was a Great Dane who befriended the sailors of Cape Town in the 1930s. Given the rank of able seaman, he boosted the morale of British sailors around the world. In this week’s episode of the Futility Closet podcast we’ll tell the story of Just Nuisance and his adventures among the sailors who loved him.
We’ll also examine early concentration camps and puzzle over a weighty fashion.