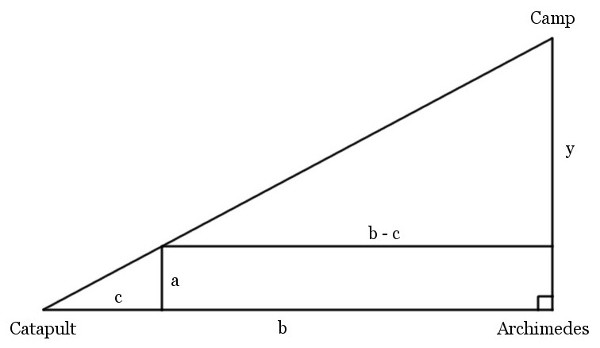
When I heard the learn’d astronomer,
When the proofs, the figures, were ranged in columns before me,
When I was shown the charts and diagrams, to add, divide, and measure them,
When I sitting heard the astronomer where he lectured with much applause in the lecture-room,
How soon unaccountable I became tired and sick,
Till rising and gliding out I wander’d off by myself,
In the mystical moist night-air, and from time to time,
Look’d up in perfect silence at the stars.
That’s Walt Whitman. In 2000, mathematician Mike Keith noted a similar idea in Psalm 19:1-6:
The heavens declare the glory of God;
And the firmament sheweth his handywork.
Day unto day uttereth speech,
And night unto night sheweth knowledge.
There is no speech nor language,
Where their voice is not heard.
Their line is gone out through all the earth,
And their words to the end of the world.
In them hath he set a tabernacle for the sun,
Which is as a bridegroom coming out of his chamber,
And rejoiceth as a strong man to run a race.
His going forth is from the end of the heaven,
And his circuit unto the ends of it:
And there is nothing hid from the heat thereof.
So he married them by rearranging the psalm’s letters:
When I had listened to the erudite astronomer,
When his high thoughts were arranged and charted before me,
When I was shown the length and breadth and height of it,
The Earth, the horned Moon, the chariot of fire,
The hundredth flight of the shuttle through heavyish air,
How soon, mysteriously, I became sad and sick,
Had to wander out, ousted, charging through the forest,
Joining the sure chaos here in a foreign heath,
Having forgotten the vocation of the learned man,
And in the mystic clearing, once more looked up
In perfect silence at the sermon in the stars.
(Michael Keith, “Anagramming the Bible,” Word Ways 33:3 [August 2000], 180-185.)