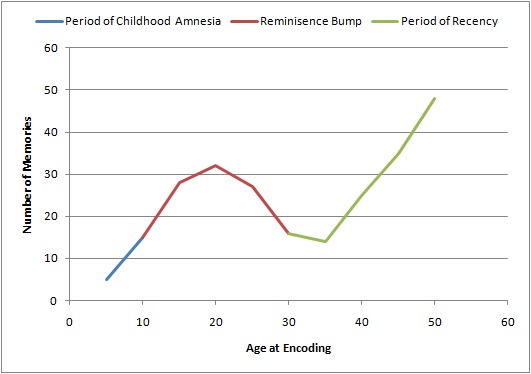
If you seem to recall your adolescence and early adulthood years more clearly than your later life, that’s normal. Most of us can recall a disproportionate number of autobiographical memories made between ages 10 and 30, perhaps because of the important changes in identity, goals, attitudes, and beliefs that most of us went through in those years. (Also, that’s the span in which many of us have novel experiences such as graduation, marriage, and the birth of a child.)
Interestingly, this phenomenon extends to favorite books, movies, and records. In a 2007 study, psychologist Steve M.J. Janssen and his colleagues at the University of Amsterdam found that subjects best recorded memories of these things between 11 and 25. This is particularly true of music: Items that aren’t revisited frequently, such as books, are more likely to be forgotten, but records have a strong “reminiscence bump.”
“Books are read two or three times, movies are watched more frequently, whereas records are listened to numerous times. The results suggest that differential encoding initially causes the reminiscence bump and that re-sampling increases the bump further.” See the appendices for lists of favorite books, movies, and records and the average ages at which subjects first encountered them.
(Steve M.J. Janssen, Antonio G. Chessa, and Jaap M.J. Murre, “Temporal Distribution of Favourite Books, Movies, and Records: Differential Encoding and Re-Sampling,” Memory 15:7 [2007], 755-767.)