In 2014 I described the Peaucellier–Lipkin linkage, a mechanism that transforms a rotary motion into a perfect straight-line motion:
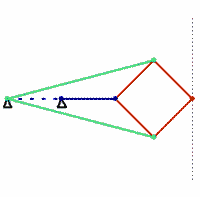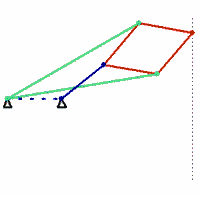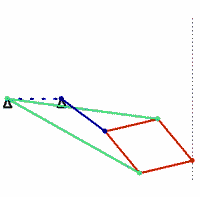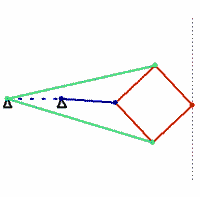
That linkage was invented in 1864 by French army engineer Charles-Nicolas Peaucellier. A decade later, Harry Hart invented two more. “Hart’s inversor” is a six-bar linkage — links of the same color are the same length. The fixed point on the left is at the midpoint of the red link, and the “input” and “output” are at the midpoints of the two blue links:
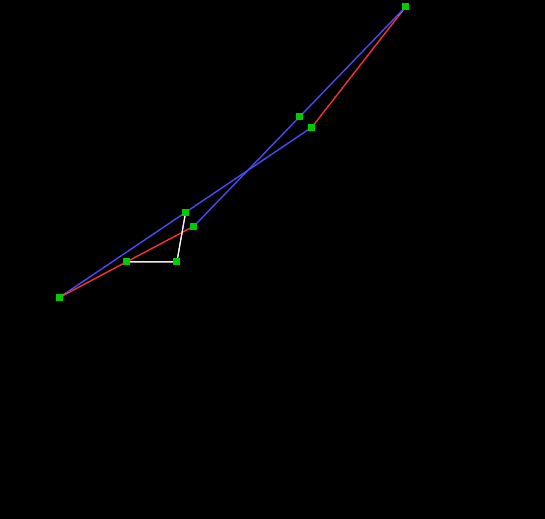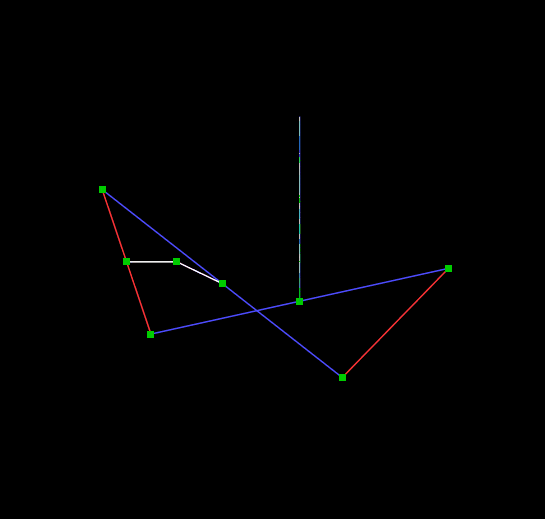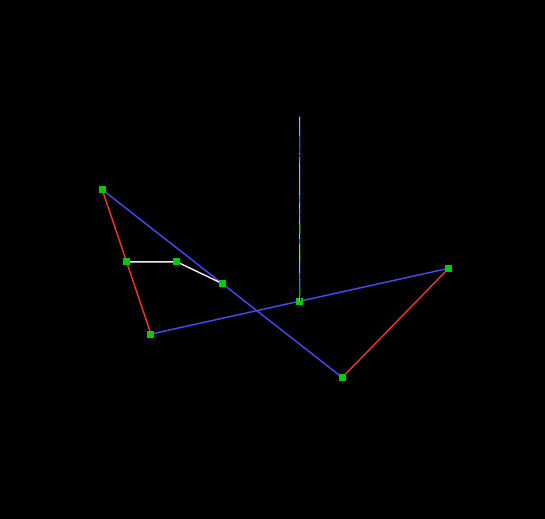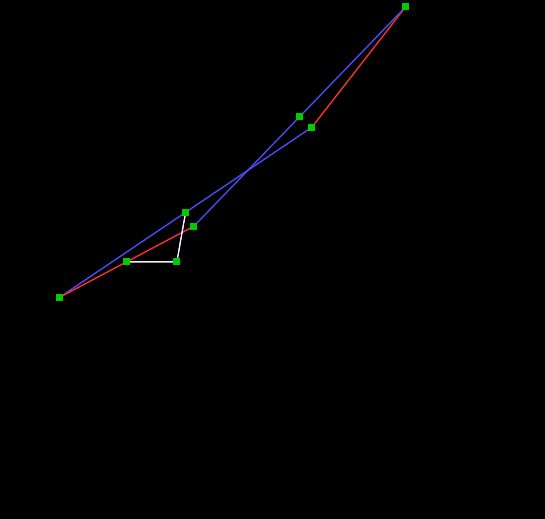
In “Hart’s A-frame,” the short links are half the length of the long ones, and the center link is a quarter of the way down the long links:
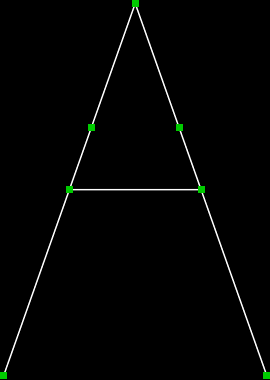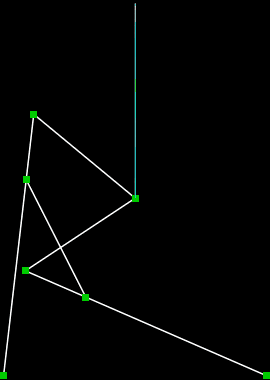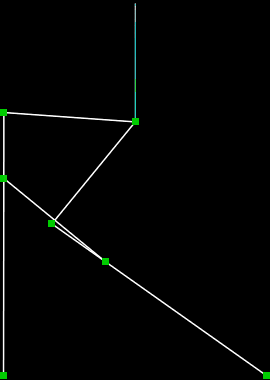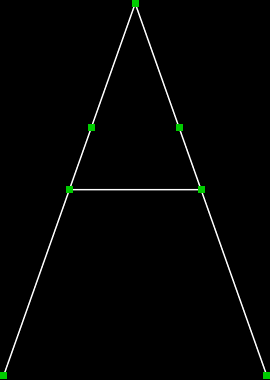
Pleasingly, the motion perpendicularly bisects a fixed link across the bottom, which is the same length as the long links.







