The Lonely Runner

https://commons.wikimedia.org/wiki/File:Lonely_runner.gif
Image: Wikimedia Commons

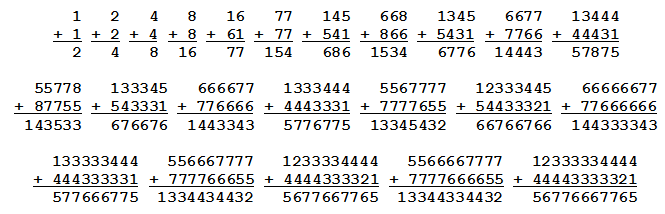
Suppose k runners are running around a circular track that’s 1 unit long. All the runners start at the same point, but they run at different speeds. A runner is said to be “lonely” if he’s at least distance 1/k along the track from every other runner. The lonely runner conjecture states that each of the runners will be lonely at some point.

This is obviously true for low values of k. If there’s a single runner, then he’s lonely before he even leaves the starting line. And if there are two runners, at some point they’ll occupy diametrically opposite points on the track, at which point both will be lonely.

But whether it’s always true, for any number of runners, remains an unsolved problem in mathematics.

| Science & Math

Good Night Lights

Six years ago, Providence restaurant The Hot Club started the custom of flashing its lights each night at 8:30, as a way to say good night to the children in the six-story Hasbro Children’s Hospital across the Providence River. The club would flash its neon sign, and patrons would gather on the waterfront deck to wave flashlights and cell phones.

Now hotels, boat traffic, skyscrapers, and police cruisers have begun to join in, some with permanent signals but many using handheld lights. About two dozen children are in the hospital on any given night, and they’ve taken to flashing back with lights of their own.

“No one knows who’s on the other side of the gesture,” hospital cartoonist Steve Brosnihan told the Associated Press. “People often say, ‘I get goosebumps hearing about this.'”

“They don’t know me; they could skip the step of flicking the lights, but they do it anyway,” said 13-year-old lupus patient Olivia Stephenson. “I hope they saw the thank you.”

| Society

Conway’s RATS Sequence

Write down an integer. Remove any zeros and sort the digits in increasing order. Now add this number to its reversal to produce a new number, and perform the same operations on that:

conway's rats sequence

In the example above, we’ve arrived at a pattern, alternating between 12333334444 and 5566667777 but adding a 3 and a 6 (respectively) with each iteration. (So the next two sums, after their digits are sorted, will be 123333334444 and 55666667777, and so on.)

Princeton mathematician John Horton Conway calls this the RATS sequence (for “reverse, add, then sort”) and in 1989 conjectured that no matter what number you start with (in base 10), you’ll either enter the divergent pattern above or find yourself in some cycle. For example, starting with 3 gives:

3, 6, 12, 33, 66, 123, 444, 888, 1677, 3489, 12333, 44556, 111, 222, 444, …

… and now we’re in a loop — the last eight terms will just repeat forever.

Conway’s colleague at Princeton, Curt McMullen, showed that the conjecture is true for all numbers less than a hundred million, and himself conjectured that every RATS sequence in bases smaller than 10 is eventually periodic. Are they right? So far neither conjecture has been disproved.

(Richard K. Guy, “Conway’s RATS and Other Reversals,” American Mathematical Monthly 96:5 [May 1989], 425-428.)

| Science & Math

Monte Kaolino

When Hirschau, Bavaria starting mining kaolinite a century ago, it faced a problem — one of the byproducts of kaolinite is quartz sand, which began piling up in enormous quantities. Fortunately sand itself has multiple uses — in the early 1950s an enterprising skier tried slaloming down the mountain, and soon the dune had its own ski club.

Today the 35-million-tonne “Monte Kaolino” even hosts the Sandboarding World Championships. And, unlike other ski resorts, it’s open in summer.

| Oddities

Breath Control

In 1999 the German state of Mecklenburg-Vorpommern passed a law governing the labeling of beef; its short title was Rinderkennzeichnungs- und Rindfleischetikettierungsüberwachungsaufgabenübertragungsgesetz (PDF). The hyphen indicates that the first word would have the same ending as the second; thus the two words are Rinderkennzeichnungsüberwachungsaufgabenübertragungsgesetz and Rindfleischetikettierungsüberwachungsaufgabenübertragungsgesetz. (The law’s formal long title is Gesetz zur Übertragung der Aufgaben für die Überwachung der Rinderkennzeichnung und Rindfleischetikettierung, or “Law on Delegation of Duties for Supervision of Cattle Marking and Beef Labeling.”)

Rindfleischetikettierungsüberwachungsaufgabenübertragungsgesetz was nominated for Word of the Year by the German Language Society. Here it is sung by the Australian National University’s UniLodge Choir:

There’s more: In 2003 a decree was passed modifying real estate regulations; its short title was Grundstücksverkehrsgenehmigungszuständigkeitsübertragungsverordnung. Can someone sing that?

| Language

A Hat Puzzle

khovanova hats

A puzzle by MIT mathematician Tanya Khovanova:

Three logicians walk into a bar. Each is wearing a hat that’s either red or blue. Each logician knows that the hats were drawn from a set of three red and two blue hats; she doesn’t know the color of her own hat but can see those of her companions.

The waiter asks, “Do you know the color of your own hat?”

The first logician answers, “I do not know.”

The second logician answers, “I do not know.”

The third logician answers, “Yes.”

What is the color of the third logician’s hat?

Click for Answer
| Puzzles

Old Words

Since it’s deliberately constructed, Esperanto doesn’t have the complex history of a natural language. But we can invent one! Manuel Halvelik created “Arcaicam Esperantom,” a fictional early form of the language akin to Old English. Here’s the Lord’s Prayer in standard Esperanto:

Patro nia, kiu estas en Ĉielo,
Estu sanktigita Via Nomo.
Venu Via regno,
Plenumiĝu Via volo
Kiel en Ĉielo, tiel ankaŭ sur Tero.
Al ni donu hodiaŭ panon nian ĉiutagan,
Kaj al ni pardonu niajn pekojn
Kiel ankaŭ ni tiujn, kiuj kontraŭ ni pekas, pardonas.
Kaj nin ne konduku en tenton
Sed nin liberigu el malbono.
Amen.

And here it is in Halvelik’s “archaic” form:

Patrom nosam, cuyu estas in Chielom,
Estu sanctiguitam Tuam Nomom.
Wenu Tuam Regnom,
Plenumizzu Tuam Wolom,
Cuyel in Chielom, ityel anquez sobrez Terom.
Nosid donu hodiez Panon nosan cheyutagan,
Ed nosid pardonu nosayn Pecoyn,
Cuyel anquez nos ityuyd cuyuy contrez nos pecait pardonaims.
Ed nosin ned conducu in Tentod,
Sed nosin liberigu ex Malbonom.
Amen.

Here’s Halvelik’s full description (PDF, in Esperanto).

| Language

Podcast Episode 147: The Call of Mount Kenya

https://commons.wikimedia.org/wiki/File:Mount_Kenya_1936.jpg

Stuck in an East African prison camp in 1943, Italian POW Felice Benuzzi needed a challenge to regain his sense of purpose. He made a plan that seemed crazy — to break out of the camp, climb Mount Kenya, and break back in. In this week’s episode of the Futility Closet podcast we’ll follow Benuzzi and two companions as they try to climb the second-highest mountain in Africa using homemade equipment.

We’ll also consider whether mirages may have doomed the Titanic and puzzle over an ineffective oath.

See full show notes …

| History · Podcast

Tommy

https://commons.wikimedia.org/wiki/File:Pet-Squirrel-Grid-LIFE-1944.jpg

During World War II one of the most surprising advocates of war bonds was Tommy Tucker, an Eastern gray squirrel who toured the nation in a humiliating wardrobe of 30 dainty costumes. (“THOUGH TOMMY IS A MALE SQUIRREL HE HAS TO WEAR FEMININE CLOTHES BECAUSE TAIL INTERFERES WITH HIS WEARING PANTS,” Life reported defensively.)

Tommy had been adopted in 1942 by the Bullis family of Washington D.C., who took him on the road in their Packard automobile, where he performed for schoolchildren, visited hospitals, and gave uninspiring radio interviews. Between appearances Zaidee Bullis would bathe him and place him in a specially made bed. At the height of his fame his fan club numbered 30,000 members.

Tommy retired after the war but gamely endured further travels with the family. When he died in 1949 he was stuffed and mounted “with his arms out so you could pull the clothes over him,” and his nightmarish fate pursued him even into the grave. He stands today in a display case in a Maryland law office — in a pink satin dress and pearls.

| Oddities