The motto of the U.S. Army Corps of Engineers is Essayons (“Let us try”).

The motto of the U.S. Army Corps of Engineers is Essayons (“Let us try”).
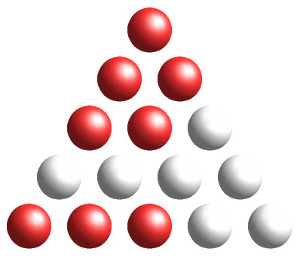
Aachen University physicist Jörg Pretz has devised a binary clock in the shape of a triangular array of 15 lamps. Here’s how to read it:
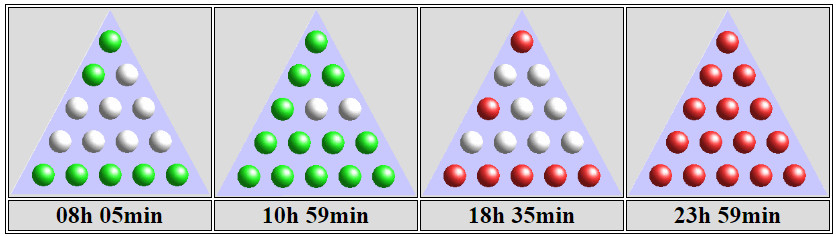
So the clock above shows 6 hours + (2 × 2 hours) + (2 × 30 minutes) + (3 × 1 minute) = 11:03. The lamps’ color, red, shows that it’s after noon, or 11:03 p.m. The same array displayed in green would mean 11:03 a.m. A few more examples:
The time value assigned to each lamp is the total time value of the row below if that row contained one additional lamp.
On each row the lamps light up from left to right, so a row with n lamps can display n + 1 states (all lamps off to all lamps on). So for a triangular array with n lamps on the bottom row, the total number of states is
(n + 1) × ((n – 1) + 1) × ((n – 2) + 1) × · · · × (1 + 1) = (n + 1)!
That is, it’s a factorial of a natural number. And by a happy coincidence, the total number of minutes in 12 hours is such a factorial (720 = 6!).
“Thus the whole concept works because our system of time divisions is based on a sexagesimal system, dating back to the Babylonians, rather than a decimal system, as proposed during the French Revolution.”
There’s more info in Pretz’s article, and you can play with the clock using this applet.
(Jörg Pretz, “The Triangular Binary Clock,” Recreational Mathematics Magazine, March 2016.)
A golden cork is, mirror-wise,
shown by a polished shelf;
yet, even if endowed with eyes,
it could not see itself.
This is because it stands aligned
with its reflected view;
but if it sideways is inclined,
such is no longer true.
O man, suppose you did reflect
straight up, let’s say, in space:
Would this not have the same effect
as in the stated case?
— Christian Morgenstern, 1905

One last puzzle from Henry Dudeney’s Canterbury Puzzles:
Abbott Francis sends for his cellarman and complains that a particular bottling of wine is not to his taste. He asks how many bottles he had produced. The cellarman tells him that there had been 12 large and 12 small bottles, and that 5 of each have been drunk. The abbot replies that three men are waiting at the gate, and orders the cellarman to give each of them some combination of full and empty bottles so that each man receives the same quantity of wine and combination of bottles.
How can the cellarman do this? He has seven large and seven small bottles full of wine, and five large and five small bottles that are empty. A large bottle holds twice as much wine as a small one, but a large bottle when empty is not worth two small ones — hence the abbot’s order that each man must take away the same number of bottles of each size.
The Italian Futurist painter Luigi Russolo had no training as a composer, but in 1913 he argued that music had become “a fantastic world superimposed on the real one,” a collection of “gentle harmonies” that pursued “purity, limpidity and sweetness of sound” but had nothing to do with the real world.
He proposed that “this limited circle of pure sounds must be broken, and the infinite variety of ‘noise-sound’ conquered.” “We find far more enjoyment in the combination of the noises of trams, backfiring motors, carriages and bawling crowds than in rehearing, for example, the ‘Eroica’ or the ‘Pastoral’.”
Accordingly he invented a new set of experimental instruments, the intonarumori, or “noise makers.” There were 27 varieties, all acoustic. Typically a performer turned a handle that rattled or bowed a set of strings, and the surrounding box and horn amplified the sound.
When Russolo and Filippo Tommaso Marinetti debuted their “noise orchestra” in April 1914, it caused a riot, but Russolo was undisturbed. “I am not a musician,” he wrote. “I have therefore no acoustical predilections, nor any works to defend.”

That’s the final entry in a minutes book discovered in May 2015 at the YMCA Buffalo Niagara in Buffalo, N.Y. The management committee of the local railroad department had met there in December 1899.
Who knows what it means? University at Buffalo archivist-in-training Matthew Oliver found it while reorganizing the YMCA’s records. Details are here.
02/20/2017 A number of readers have written in with a likely answer: The reference is to 1 Samuel 7:12, “Then Samuel took a stone and set it up between Mizpah and Shen. He named it Ebenezer, saying, ‘Thus far the LORD has helped us.'” The Israelites erected the stone to commemorate their victory over the Philistines. This meeting took place during the Third Great Awakening, when the reference would have been well understood.
Reader Phil Moberg Jr. writes, “The ‘Railroad Ys,’ as they were known to those of us in the business, were a great improvement in the general living conditions to crews between runs, being a more than welcome change from the seedy flophouses and saloons that preceded them. The last of them in Southern New England closed in the early ’70s, with the building that housed the New Haven (CT) Railroad Y having been torn down late last year.”
(Thanks also to Delyth Yabar and Anthony Douglas.)
Reader Joe Antognini sent this in: Brazilian mathematician Inder Taneja has found a way to render every number from 1 to 11,111 by starting with either of these strings:
1 2 3 4 5 6 7 8 9
9 8 7 6 5 4 3 2 1
and applying any of the operations addition, subtraction, multiplication, division, and exponentiation. Brackets are permitted. For example:
6439 = 1 + 2 × (34 × 5 × 6 + 789)
and
6439 = 9 × (8 + 7 + 6) + 54 × (32 + 1)
Intriguingly, there’s one hole: There doesn’t seem to be a way to render 10958 from the increasing sequence.
Taneja’s paper is here. (Thanks, Joe.)
01/29/2017 UPDATE: Taneja tells me that, while it can’t be calculated using only basic operations, 10958 can be reached using factorials or square roots. Here are two factorials:
10958 = 1 + 2 + 3!! + (-4 + 5! + 6 – 7) × 89
10958 = 1 × 2 × (3!! – 4! × (5 + 6) + 7! – 8 – 9)

U.S. senator Alan Cranston once lost a copyright suit to Adolf Hitler. Cranston, who had begun his career in journalism, spotted an abridged translation of Mein Kampf in a New York bookstore in 1939. He had read the full text in German and was concerned that the English adaptation omitted Hitler’s anti-Semitism and ambitions to dominate Europe.
To publicize the truth, Cranston worked with a friend to publish an anti-Nazi version of the book. “I wrote this, dictated it [from Hitler’s German text] in about eight days, to a battery of secretaries in a loft in Manhattan,” Cranston told the Los Angeles Times in 1988. They produced a tabloid edition of 32 pages, reducing Hitler’s 270,000 words to 70,000 to yield a “Reader’s Digest-like version [showing] the worst of Hitler.”
At 10 cents apiece, Cranston’s version sold half a million copies in 10 days. But by that time the original was a best-seller in Germany, and the publishers sued Cranston for undercutting the market. In June the U.S. Circuit Court of Appeals in New York ordered the presses stopped. The truth had gotten out, Cranston said, but “we had to throw away half a million copies.”

Should parents be licensed? We ask teachers to study full-time for years and to pass qualifying exams before we let them educate children for six hours a day. And we carefully assess the suitability of adoptive and foster parents. But anyone has the right to become a biological parent without any training at all in child development.
Philosopher Peg Tittle writes, “How many children have been punished because they could not do what their parents mistakenly thought they should be able to do at a certain age — remember X, carry Y, say Z? How many have been disadvantaged because they grew up on junk food — for their bodies as well as their minds? How many have been neglected because their parents didn’t notice the seeds of some talent?”
Today’s children are tomorrow’s citizens, so the public has a legitimate concern in this. Psychiatrist Jack Westman writes, “The way children are parented plays a vital role in the quality of all our lives. We no longer can afford to avoid defining and confronting incompetent parenting.”
Psychologist Roger McIntire writes, “We already license pilots, salesmen, scuba divers, plumbers, electricians, teachers, veterinarians, cab drivers, soil testers, and television repairmen. … Are our TV sets and toilets more important to us than our children?”
(Peg Tittle, ed., Should Parents Be Licensed?, 2004.)