When Orville Wright died, Neil Armstrong was already 17 years old.

When Orville Wright died, Neil Armstrong was already 17 years old.
José Paluzie offered this chess poser in 1910. White is to move and mate in 1:
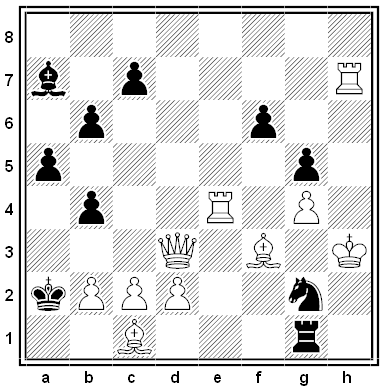
The key is to notice that the position is illegal: There’s no legal way for the black king to have arrived at a2. Black, desperate to avoid mate, must have put it there when White wasn’t looking.
Where did it come from? It doesn’t matter: White can place the black king on any legal square and mate in 1.
(From Burt Hochberg’s Chess Braintwisters, 1999.)

During wargames in Louisiana in September 1941, the U.S. Army found itself drawn into a tense firefight with an unseen enemy across the Cane River. The attacker turned out to be three boys with a toy cannon. In this week’s episode of the Futility Closet podcast we’ll revisit the Battle of Bermuda Bridge and the Prudhomme brothers’ account of their historic engagement.
We’ll also rhapsodize on guinea pigs and puzzle over some praiseworthy incompetence.
During World War I, cable censors would sometimes change a word here and there in a telegram, preserving the meaning but hoping to interfere with any enemy codes the messages might contain.
‘Father is dead,’ ran a cablegram from Sweden to New York which passed through the British censorship.
For some inexplicable reason the censor didn’t like the word ‘dead.’ He changed it to ‘deceased.’
Within a short time this question, sent from New York to Sweden, passed through the hands of the same censor: ‘Is father dead or deceased?’
“What did that word ‘dead’ mean? It might have covered a whole volume of enemy news; it might have provoked a disaster on land or sea. And yet the censor had no better reason for cutting it out than a certain ‘hunch’ which came over him that the word ought to be changed.”
(“Our Dear Friend, the Censor,” American Printer, June 5, 1917.)
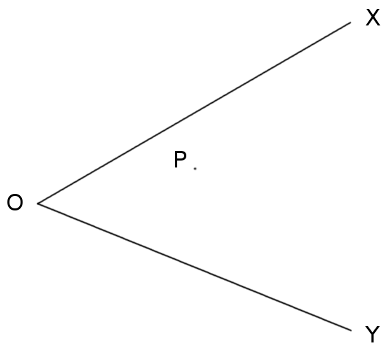
Point P lies within acute angle XOY. How can we find a point A on OX and a point B on OY such that P is the midpoint of a segment drawn between them?

In James Joyce’s Ulysses, the events of Bloomsday are so carefully worked out that even incidents of weather can be recognized across the various episodes. Episode 1, at the tower:
A cloud began to cover the sun slowly, wholly, shadowing the bay in deeper green.
In Episode 4, Bloom notices the same thing as he walks home from Dlugacz’s shop:
A cloud began to cover the sun slowly, wholly. Grey. Far.
Four paragraphs later the cloud has passed:
Quick warm sunlight came running from Berkeley road, swiftly, in slim sandals, along the brightening footpath.
And back at the tower it passes as well:
Stephen, still trembling at his soul’s cry, heard warm running sunlight and in the air behind him friendly words.
“The breeze is therefore approximately from the west, that being the prevailing direction of winds in the British Isles,” observes Ian Gunn in James Joyce’s Dublin (2004).
A Quaker objector in the Civil War:
I was ordered out and required to fall in line with the company and drill, but I refused. They tried to make me and I sat down on the ground. They reminded me of the orders to shoot me, but I told them my God said to fear them not that kill the body, but are not able to kill the soul; but rather to fear him that is able to destroy both soul and body in hell. The company was then ordered to fall back eight paces, leaving me in front of them. They were then ordered by Colonel Kirkland to ‘Load; Present arms; Aim,’ and their guns were pointed directly at my breast. I raised my arms and prayed: ‘Father, forgive them; they know not what they do.’ Not a gun was fired. They lowered them without orders, and some of them were heard to say that they ‘could not shoot such a man.’ The order was then given, ‘Ground arms.’
After weeks of such punishment, William Hockett was captured at Gettysburg and released to live in Philadelphia. He remained there until the end of the war.

In 1895, stung by charges that boxing is a brutal sport, Joseph Donovan patented the training rig on the left. Each boxer wears a harness and headgear with electrical contacts at each of the classic vulnerable points: the heart, the pit of the stomach, the chin, the nose, etc. When a sparring partner hits one of these points, a bell sounds and points are scored.
Donovan argued that this makes the scoring more objective and the sport more civilized. “It renders one of the healthiest and most fascinating athletic exercises absolutely safe,” he wrote, “doing away completely with roughing, bloodletting, brutality, knockdowns, and knockouts, and reducing boxing and the manly art of self-defense to a science, in which rapidity of arm and leg work, endurance, and quick conception are the only factors.”
In the same spirit, in 1956 Willie Roberson patented a glove with a built-in counter (right): “Each time a blow above a predetermined force is struck, such blow will be recorded, whereby the total number of effective blows struck during a boxing match will be readily available to the referee and judges judging the boxing match.” If we combine the two then we can even confirm the counts!

Writing in American Speech in 1931, L.W. Merryweather predicted that “hell fills so large a part in the American vulgate that it will probably be worn out in a few years.” He proposed that “clerical circles should take it upon themselves, as a public duty, to invest some other theological term with a shuddering fearsomeness that will qualify it as a successor to hell, when the lamentable decease of the latter actually takes place.” He counted 14 usages:
Fourteen years later, in The American Language, H.L. Mencken wrote, “Fortunately, his fears have not been borne out by the event. Hell still flourishes in the Republic, in so far as profanity flourishes at all, and every one of the combinations and permutations of it that he listed remains in use.”
(L.W. Merryweather, “Hell in American Speech,” American Speech 6:6 [August 1931], 433-435.)

“I love criticism just so long as it’s unqualified praise.” — Noël Coward