
Four be the things I’d been better without:
Love, curiosity, freckles, and doubt.
— Dorothy Parker

Four be the things I’d been better without:
Love, curiosity, freckles, and doubt.
— Dorothy Parker
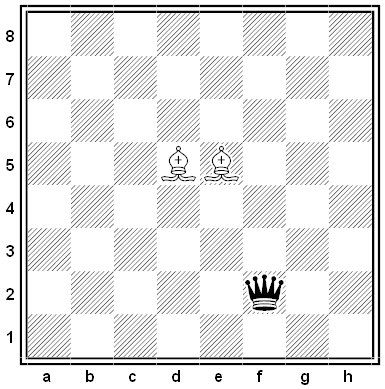
By M. Techritz. Place the black and white kings on the board so that White can mate in one move.

You exist because of a fragile string of circumstances: Your parents had to meet and procreate at a particular time, and so did their parents, and so on. If any of these things had not happened, you would not be here.
But the past that produced you also produced a whole series of historical and natural calamities — the Holocaust, World War I, and slavery, for example. Very likely those calamities influenced the delicate causal chain that leads to your existence. Without them, your ancestors would not have met and had children when they did. Properly speaking, then, shouldn’t you regret your own existence, since it required these tragedies to bring it about?
University of Haifa philosopher Saul Smilansky writes: “A ‘package deal’ is involved here: those events, together with oneself; or, the absence of the historical calamity, and the absence of oneself. So, all considered, ought one to prefer never to have existed, and to regret that one exists?”
(Saul Smilansky, “Morally, Should We Prefer Never to Have Existed?”, Australasian Journal of Philosophy 91:4, 655-666.)
If two people want to split up amicably, the easiest solution is to divide their assets equally, with each partner getting 0.5. But suppose that one partner goes to a lawyer who charges a fee f but promises to get more, by an amount m + f, leaving his client better off by the amount m. If this happens, then the second partner will get only 0.5 – m – f. If the second partner engages their own lawyer then the split is equal again, except that now the lawyers’ fees must be paid:
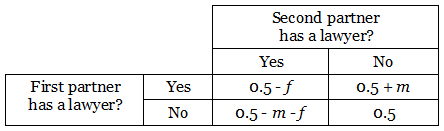
This is an example of the so-called prisoner’s dilemma: Both sides would be better off if they left the lawyers out of it, but if one engages a lawyer than the other had better do so as well.
Now suppose that each partner can choose the amount of lawyer time to buy, and that they get a payoff that’s proportional to the amount they spend. If one spends x on lawyers and the other spends y, each measured as a fraction of the total assets, then the first partner should receive an amount given by:
An industrious divorcee can now use calculus to maximize this expression, varying x and keeping y constant. The optimum value of x turns out to be . If my partner spends 9%, or 0.09, of our assets on lawyers, then I should spend
. Then my partner will get 0.21 of the assets, and I’ll get 0.49, and the lawyers get the rest.
Well, now what? Knowing all this, what’s our best course? If we could trust each other then we’d each pay a pittance on lawyers and get nearly 0.5 each. But I’m aware that if you pay a millionth and I pay a thousandth (still nearly a pittance), I’ll get nearly 99.9% of our assets. And simply resolving to outspend you won’t work: If you spend 0.36 then I should spend 0.24; I’ll come away with less than you, but this is the best I can do.
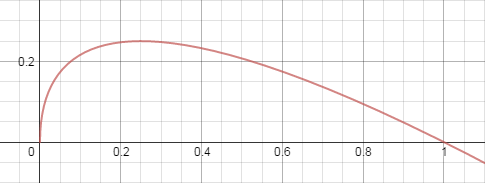
“Looking at the graph of , above, we (the author and reader) see that y = 0.25 gives us x = 0.25, and this gives us a sort of stability,” writes Anthony C. Robin in the Mathematical Gazette. “Neither partner can pull a fast one over the other, and it results in the assets being equally shared between us, them, and the lawyers. No doubt this is the reason why lawyers are so rich in our society!”
(Anthony C. Robin, “How Lawyers Make a Living,” Mathematical Gazette 88:512 [July 2004], 313-315.)
“I have seen a thousand graves opened, and always perceived that whatever was gone, the teeth and the hair remained of those who had died with them. Is this not odd? They go the very first things in youth and yet last the longest in the dust.” — Lord Byron, letter to John Murray, Nov. 18, 1820
ditation
n. enrichment
improficuous
adj. unprofitable
Another puzzle by Yakov Perelman:
“Two fathers gave their two sons some money. One gave his son 150 rubles and the other 100 rubles. When the two sons counted their finances, they found that together they had become richer by only 150 rubles. What is the explanation?”
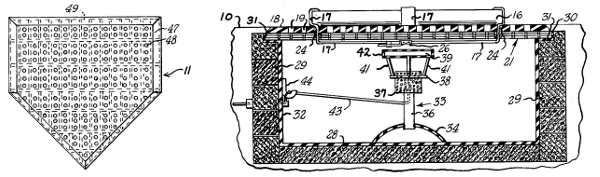
“It is highly desirable for the spectators at a baseball game to hear what is transpiring on the playing field,” observed inventor James Sellers in 1959, “such as arguments at the bases between opposing players, and discussions between the umpires and players.”
Accordingly he patented an “apparatus for transmitting sound from a baseball field.” Each base is fitted with a hidden microphone, which sends its signal to the announcers’ PA system.
“The sounds on the playing field can thus be transmitted through the control booth to the public address system so that spectators in the grandstand may hear what is taking place on the playing field.”
“By transmitting the sounds from the playing field to the grandstand, the spectators feel that they are taking part in the game. Also, it enables the spectators to judge a play better as they can hear the baseball strike the glove or mitt of a player.”
In the backing vocals on “Paperback Writer” (1:02 above), John and George are singing “Frère Jacques.”

What do René Descartes, Joseph Haydn, and Oliver Cromwell have in common? All three lost their heads after death. In this week’s episode of the Futility Closet podcast, we’ll run down a list of notable corpses whose parts have gone wandering.
We’ll also hear readers chime in on John Lennon, knitting, diaries and Hitchcock, and puzzle over why a pilot would choose to land in a field of grazing livestock.

Painted on the east side of the Lani Nalu Plaza building in Honolulu, trompe-l’oeil artist John Pugh’s mural Mana Nalu (Power of the Wave) depicts Liliuokalani, the last monarch of the Hawaiian Islands, and surfing pioneer Duke Kahanamoku.
Pugh took a year and a half to create the image, working with 14 other artists. The whole scene is painted, including the wave, the skylight, the balcony, the urns, the children, and the staircase.
“After the mural was near completion,” Pugh writes, “a fire truck with crew stopped in the middle of traffic and jumped out to rescue the children in the mural. They got about 15 feet away and then doubled over laughing that they were fooled into an emergency response mode. I don’t think that there were any liability issues for a false report.”
More of Pugh’s work here and on his website.
(Thanks, Ron.)