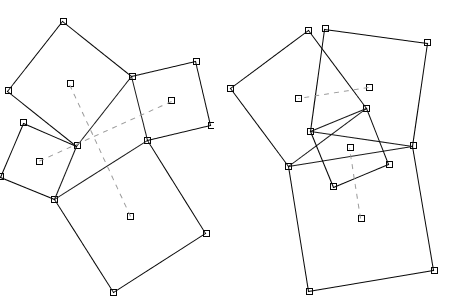
Draw any quadrilateral and build a square on each of its sides.
Connect the centers of opposite squares, and these two line segments will be perpendicular and of equal length.

Draw any quadrilateral and build a square on each of its sides.
Connect the centers of opposite squares, and these two line segments will be perpendicular and of equal length.

Glimpses from the first day of the Battle of the Somme, July 1, 1916:
“I was ordered to fetch the breakfast from the kitchens about a mile away. On the way back, in the trench, we came across a covey of young partridges and, as we walked along, we were driving them in front of us. A lot fell in a sump which was full of water so they would surely drown. But I could not see them drown, so I pulled the top off and got them out, put them in my steel helmet and lifted it up to the top of the trench. There, their mother was waiting and she chuckled them all together and off they went, never to be seen by us again.” — Pte F.G. Foskett, 7th Bedfords
“I suppose a shell hole is not the best place from which to admire anything but, believe it or not, waving about just over my head were two full-blown red poppies which stood out in pleasant contrast against the azure blue sky.” — Pte G.E. Waller, Glasgow Boys’ Brigade Battalion
“The worst sights were in our own trenches where some of the badly wounded had managed to crawl. We were not allowed to help any of them, but kept our machine-gun mounted on the parapet in case of a counter-attack. The wounded were trying to patch each other up with their field dressings. A chaplain tore his dog collar off in front of me and, with curses, said, ‘It is a mockery to wear it.'” — Pte C.A. Turner, 97th Brigade Machine Gun Company
“I could see, away to my left and right, long lines of men. Then I heard the ‘patter, patter’ of machine-guns in the distance. By the time I’d gone another ten yards there seemed to be only a few men left around me; by the time I had gone twenty yards, I seemed to be on my own. Then I was hit myself.” — Sgt. J. Galloway, 3rd Tyneside Irish
“Now we came on to a German machine-gun post and there were all the twelve of the crew lying dead around the machine-gun; a short distance away we saw the body of one of our sergeants, formerly one of the king’s footmen who joined up with us at Norwich. He had obviously accounted for the machine-gun crew, before he himself received his death blow. A strange feeling possesses one at such a moment. It seems as if one is detached and merely looking at a scene of carnage from a great distance.” — Pte WC. Bennett, 8th Norfolks
“I then went on to the second-line trench and jumped in, to see a German soldier lying on the parapet. With fixed bayonet I approached, then I saw his putty-coloured face which convinced me he was mortally wounded. The German brought up an arm and actually saluted me. I understood no German language but the poor chap kept muttering two words ‘Wasser, Wasser,’ and ‘Mutter, Mutter.’ It took me a minute or so to realize he wanted a drink of water. The second word I could not cotton on to. I am glad to this day that I gave him a drink from my precious water.” — Pte G.R.S. Mayne 11th Royal Fusiliers
“We are filled with a terrible hate. Our actions are born of a terrible fear, the will to survive. Some of the Germans were getting out of their trenches, their hands up in surrender; others were running back to their reserve trenches. To us they had to be killed. Kill or be killed. You are not normal.” — L/Cpl J.J. Cousins, 7th Bedfords
A company commander in the London Division’s Pioneer battalion was left out of the battle: “My recollection, after all these years, is of being in a trench discussing the rumours, helping with the wounded (we had four men killed) and occasionally lying in a bit of shelter, reading Pickwick Papers and watching the activities of a fat and grey rat.” — Capt. P.H. Jolliffe, 1/5th Cheshires
(From Martin Middlebrook, The First Day on the Somme, 1971.)

“When anyone asks me how I can best describe my experience in nearly 40 years at sea, I merely say uneventful.” — Edward Smith, captain of the Titanic, in 1907

More maxims of La Rochefoucauld:
I give you now Professor Twist
A conscientious scientist.
Trustees exclaimed “He never bungles,”
And sent him off to distant jungles.
Camped by a tropic riverside,
One day he missed his loving bride.
She had, the guide informed him later,
Been eaten by an alligator.
Professor Twist could not but smile.
“You mean,” he said, “a crocodile.”
— Ogden Nash
(Thanks, Steve.)

English essayist A.C. Benson had rich, elaborate dreams, a trait common in his family. “Sometimes they would be processions and high ceremonies, diversified by the intervention of old Eton friends, who would whisper dark words more suo during some strange liturgy,” recalled his friend Geoffrey Madan. “Sometimes the distant past would rush upon him and old ecclesiastics, summoned up from the mists of Addington, became involved with him in situations of infinite absurdity; sometimes it would be oneself with whom the drama was played, till its recital at breakfast made one helpless with laughter.”
From one dream he awoke recalling only a strange epigram, “The riddle of life is solved by gliding, and not sliding.” On another morning he found that he had scribbled down these lines in the middle of the night:
A bold and cheerful company of Ogres, Ghosts, and Ghouls
Attacked and smashed to little bits the City of Tomfools:
The Tomfools sailed to Araby, and raised another state;
I can’t say how refined they were, and how considerate.
And now in High Tomfoolery they’re very fond of telling
What an almighty hash the ghosts made of their former dwelling;
They chaunt their great deliverance: they teach and preach and say
How good it was of God to take their former pride away.
He composed his poem “The Phoenix” entirely in his sleep. “I dreamed the whole poem in a dream, in 1894, I think, and wrote it down in the middle of the night on a scrap of paper by my bedside,” he wrote. “It is a lyric of a style which I have never attempted before or since. … I really can offer no explanation either of the idea of the poem or its interpretation. It came to me so (apparently) without any definite volition of my own that I don’t profess to understand or to be able to interpret the symbolism.”
By feathers green, across Casbeen,
The pilgrims track the Phoenix flown,
By gems he strewed in waste and wood
And jewelled plumes at random thrown.
Till wandering far, by moon and star,
They stand beside the fruitful pyre,
Whence breaking bright with sanguine light,
The impulsive bird forgets his sire.
Those ashes shine like ruby wine,
Like bag of Tyrian murex spilt;
The claw, the jowl of the flying fowl
Are with the glorious anguish gilt.
So rare the light, so rich the sight,
Those pilgrim men, on profit bent,
Drop hands and eyes and merchandise,
And are with gazing most content.
Madan added, “I have preserved in one of his letters the concluding stanza which he wrote in waking hours to round it off, but omitted later on the advice of a friend who felt it to be ‘incongruous’; this pleased him very much indeed.”
(From “A Later Friendship,” by Geoffrey Madan, in Arthur Christopher Benson as Seen by Some Friends, 1925.)
cultrivorous
adj. devouring knives
In June 1799, having watched a French mountebank pretend to swallow clasped knives, 23-year-old American sailor John Cummings boasted that he could do the same, and “after drinking freely” he proceeded to swallow his own pocketknife and three others offered by his friends.
Thus began a memorable career. According to George Budd in the Medical Times & Gazette, Cummings recounted his exploit in Boston six years later and was immediately challenged to repeat it. He swallowed six more knives, and an additional eight the following morning, “so that he had swallowed a knife for every day that the month was old.”
Nine months later, again inebriated, he made the same boast in England and swallowed five knives on Dec. 4 and nine clasp knives on Dec. 5 (plus, he was told, another four that he was too drunk to remember).
Through the next four years, in great pain and continually vomiting, Cummings applied to a number of doctors, at least one of whom dismissed his story as incredible. But when he died finally in March 1809, his stomach was opened and “a great many portions of blades, knife-springs, and handles were found in it, and were carefully collected for the museum at Guy’s Hospital, in which they are now preserved,” Budd notes — Cummings’ contribution to medical science.
After taking part in the attack on Pearl Harbor, Japanese fighter pilot Shigenori Nishikaichi crash-landed on the isolated Hawaiian island of Niihau. In this episode of the Futility Closet podcast we’ll recount the six days of escalating drama that unfolded between the desperate pilot and the terrified islanders.
We’ll also hear a list of open questions from Greg’s research and puzzle over why a man can’t sell a solid gold letter opener.
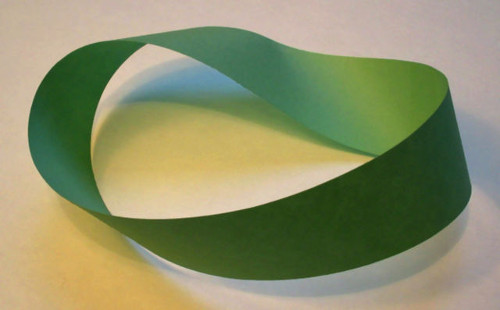
Gabriel Josipovici’s 1974 short story “Mobius the Stripper” is subtitled “A Topological Exercise.” The text is written in two strips, which tell two ostensibly different stories.
The first strip tells the story of Mobius, a man of uncertain origin who feels a metaphysical need to strip, “to take off what society has put on me” and discover his true self. He takes a job at a London club, where he talks as he performs and feels his essential self emerging. In the end, though, he comes to an existential crisis, unable to find any ultimate meaning, and shoots himself in his room to provide “an example to all.”
The second strip describes the troubles of an unnamed writer who shuts the world away, eager to write something new but overcome with writer’s block and intimidated by the writers of the past. His friend Jenny urges him to see a stripper named Mobius. “It’ll change your ideas,” she says. “Give it a break and you’ll all of a sudden see the light.” In the end, desperate to overcome the block, he begins to write a story about Mobius, whom he has never seen. “Perhaps it was only one story, arbitrary, incomplete, but suddenly I knew that it would make its own necessity and in the process give me back my lost self.”
If these tales are written on either side of a strip of paper, and one end of the strip is given a half-turn and then attached to the other, they create one unending story in which Mobius’ example frees the writer, who in his story gives new fictional depth to Mobius’ struggle, which lends it greater meaning and inspiration, and so on. Mobius is described differently in the two stories, suggesting that the Mobius of the first story is largely an invention of the writer in the second story. So where does the inspiration come from?