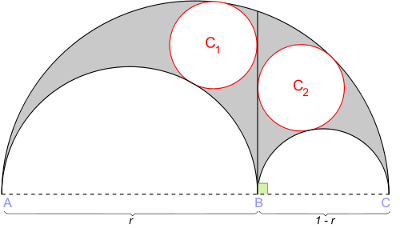
Pick any three points on a line and use each pair of them to define a semicircle, as shown.
Now draw a perpendicular between the two smaller semicircles.
Circles c1 and c2 will always have the same area.

Pick any three points on a line and use each pair of them to define a semicircle, as shown.
Now draw a perpendicular between the two smaller semicircles.
Circles c1 and c2 will always have the same area.
Lesser-known maxims from Poor Richard’s Almanack:
“Sin is not hurtful because it is forbidden, but it is forbidden because it is hurtful. Nor is a duty beneficial because it is commanded, but it is commanded because it is beneficial.”
bellytimber
n. food, provisions
magirology
n. the art or science of cookery
gastrosoph
n. an expert or skilled eater

In 1880, Sidney Lanier made an important announcement to his 11-year-old son Charley:
West Chester, Pa.
August 15, 1880My dear Charley:
A young man came to our house yesterday morning who claims that he is a brother of yours and Sidney’s and Harry’s and that he is entitled to all the rights and privileges appertaining unto that honorable connection. … He is a most exemplary young man. He never stays out late at night; neither chews, smokes, nor uses snuff; abstains from all intoxicating liquors, and does not touch even tea or coffee; however much preserves and fruit-cake there may be on the supper-table, he never asks for any; he does no kind of work on the Sabbath; he honors his father and mother, particularly his mother; he plays no games of hazard, not even marbles for winnance; and I am positively certain that in the whole course of his life he has never uttered a single angry or ungentlemanly word. I am bound to admit that he has his shortcomings: he isn’t as particular about his clothes as I would like to see him; he has a way of trying to get both fists in his mouth which certainly does look odd in company; and he wants his breakfast in the morning at four o’clock — an hour at which it is very inconvenient, with our household arrangements, to furnish it to him. …
Earnestly hoping that this lovely little (for I omitted to mention that he is small of stature) brother Rob may find a good warm place in your three hearts without being obliged to resort to extreme measures, and with a hundred embraces for you, me dear big Charley,
I am
Your &c &c &c.
When Arizona copper prospector James Kidd disappeared in 1949, he left behind a curious will:
this is my first and only will and is dated the second day in January 1946. I have no heirs have not married in my life, after all my funeral expenses have been paid and #100, one hundred dollars to some preacher of the gospital to say fare well at my grave sell all my property which is all in cash and stocks with E F Hutton Co Phoenix some in safety deposit box, and have this balance money go into a reserach or some scientific proof of a soul of the human body which leaves at death I think in time their can be a Photograph of soul leaving the human at death, James Kidd
He left it in a safe deposit box, so it didn’t come to light until 1963, by which time Kidd’s estate had appreciated to nearly $200,000. This attracted more than 100 claimants, each of which argued it was best qualified to find the human soul. The Neurological Sciences Foundation of Phoenix, for example, said that it was working with hallucinogenic agents, biochemical controls of the brain, and the nervous system. “To the extent that the ‘soul’ is a function of the human body,” it insisted, “to this extent our work … is relevant to the intent of the will.”
Arizona superior court judge Robert L. Myers finally awarded the legacy to a local neurological institute, but after an additional six years of litigation it went to the American Society for Psychical Research. “The Kidd legacy was not only a windfall,” wrote Nicholas Wade in Science, “but proved the parapsychologists could at least convince a court of the seriousness of their intentions.”
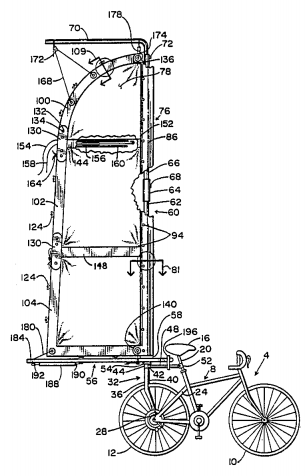
For the conservation-minded, in 1988 Joseph Beck patented a “sail attachment” for a bicycle. The mast extends upward from the rear wheel, and it’s mounted on a pivot so that it will swing out of the way if you run into something. I’m not sure how you’d store it, though.
Related: In 1826 George Pocock invented a buggy drawn by kites.
An American, named Sanborn, living at Medford, Mass., in his will, dated 1871, bequeathed his body to Harvard University, and ‘especially to the manipulation of Oliver Wendell Holmes and Louis Agassiz.’ He requested that his skin be made into two drumheads, to become the property of his life-long friend, Warren Simpson, leader of a drum corps, of Cohasset, on condition that on Bunker Hill at sunrise, June 17th, each year, he should beat on the said drum the tune of ‘Yankee Doodle.’ On one drum-head was to be inscribed Pope’s ‘Universal Prayer,’ and on the other the ‘Declaration of Independence.’
‘The remainder of my body,’ he continues, ‘unless for anatomical purposes, to be composted for a fertilizer to contribute to the growth of an American elm, to be planted in some rural thoroughfare, that the weary wayfarer may rest, and innocent children play beneath its umbrageous branches rendered luxuriant by my remains.’
— Current Opinion, 1902

When the British Army went ashore at Normandy, private Bill Millin was wearing a Cameron tartan kilt and playing “Hielan’ Laddie” on his bagpipes. Unarmed except for a ceremonial dagger, he marched up and down the water’s edge, blasting out tunes, and miraculously was not hit. Millin was personal piper to Lord Lovat, commander of 1st Special Service Brigade. The War Office had banned pipers from leading soldiers into battle after many were lost in World War I. “But that’s the English War Office,” Lovat told him. “You and I are both Scottish, and that doesn’t apply.”
“Mad Jack” Churchill enjoyed danger so much that he fought World War II with arrows and a broadsword — that’s him on the far right below, leading a training exercise in Scotland.
“Any officer who goes into action without his sword,” he said, “is improperly dressed.”
Churchill charged through the whole war this way — he’s the only British soldier to fell an enemy with a longbow — and yet he lived to be 90. He died peacefully in Surrey in 1996.


William Howard Taft weighed as much as 340 pounds during his presidency, but after leaving office he lost 70 pounds and kept it off for the remainder of his life. He shared his diet on the front page of the New York Times:
“I have dropped potatoes entirely from my bill of fare, and also bread in all forms. Pork is also tabooed, as well as other meats in which there is a large percentage of fat. All vegetables except potatoes are permitted, and of meats, that of all fowls is permitted. In the fish line I abstain from salmon and bluefish, which are the fat members of the fish family. I am also careful not to drink more than two glasses of water at each meal. I abstain from wines and liquors of all kinds, as well as tobacco in every form.”
“I can truthfully say that I never felt any younger in all my life,” he said. “Too much flesh is bad for any man.”
Today we’re launching a weekly podcast, on which we’ll answer listener questions, discuss recent popular posts on Futility Closet, share readers’ contributions on previous topics, present intriguing leads that we’ve encountered in our research, and offer a challenge in which listeners can match their wits.

Will New Year’s Day fall on a weekend in the year 2063? If calendar reformer Moses Cotsworth had succeeded, anyone in the world could have answered that question instantly — any of us could name the day of the week on which any future date would fall, no matter how distant. In this first episode we examine Cotsworth’s plan and similar efforts to improve our clocks and calendars.
We also look at how an antique dollhouse offers a surprising window into 17th-century Dutch history, explore a curious puzzle in an Alfred Hitchcock film, and invite you to participate in the first Futility Closet Challenge.