-ESS changes POET to POETESS, -ETTE changes BACHELOR to BACHELORETTE, -INA changes CZAR to CZARINA, and -INE changes HERO to HEROINE. What suffix changes a word from feminine to masculine?
In Other Words
Lexicon Recentis Latinitas, published by the Vatican, invents Latin versions of modern words and phrases, so students can refer to items that didn’t exist in the ancient world:
bestseller: liber maxime divenditus
car wash: autocinetorum lavatrix
Christmas tree: arbor natalicia
disc brakes: sufflamen disci forma
dishwasher: escariorum lavator
to flirt: lusorie amare
leased property: locatio in emptionem convertibilis
pinball machine: sphaeriludium electricum nomismate actum
refrigerator: cella frigorifera
to slack off on the job: neglegenter operor
television: instrumentum televisificum
traffic jam: fluxus interclusio
washing machine: machina linteorum lavatoria
These examples are from a selection published in 1991 in Harper’s, which said that 75 percent of the 18,000 entries in that year’s edition were terms that had never had Latin equivalents. I can’t find the whole book, but the Vatican website offers an Italian-Latin glossary with some entries in English (hot pants are brevíssimae bracae femíneae).
Black and White
Second Senses
Entries from the Complete Uxbridge English Dictionary:
beehive: what Australian teachers tell you to do
blistering: someone you enjoy calling on the phone
cannelloni: Scots refusal to give one an overdraft
cherish: rather like a chair
colliery: sort of like a collie but even more so
emboss: to promote to the top
female: chemical name for Iron Man
flatulence: an emergency vehicle that picks you up after you have been run over by a steamroller
Icelander: to tell lies about Apple
ivy: the Roman for four
lamb shank: Sean Connery’s sheep has drowned
laundress: grass skirt
pastrami: the art of meat folding
quick: noise made by a New Zealand duck
splint: to run very fast with a broken leg
Venezuela: a gondola with a harpoon
wisteria: a nostalgic form of panic
xylophone: the Greek goddess of Scrabble
A foible is “something coughed up by a New York cat.”
Retro Cinema
The 1984 action comedy Top Secret! contains an odd sequence set in a Swedish bookstore. Val Kilmer, Lucy Gutteridge, and Peter Cushing acted the entire scene backward, and the filmmakers then reversed this performance to produce a dreamlike atmosphere in which impossible things happen.
The scene required 17 takes and four dogs, co-director Jim Abrahams told ScreenCrush. “Each dog stopped being hungry.”
A Pretty Find
Write the word CESAROLITE in a circle and then trace out the letters in its anagram ESOTERICAL — the result is a perfect 10-pointed star:
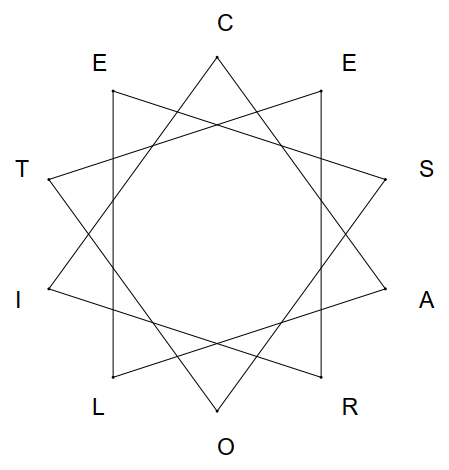
Only 5.7 percent of anagrams in English are “maximally shuffled,” meaning that no letter retains its original neighbors. And even those rarely produce such pleasing symmetry when they’re diagramed like this. This is the largest “perfect” star anagram found in a systematic search by Jason Parker and Dan Barker; for more, see the link below.
(Jason Parker and Dan Barker, “Star Anagram Detection and Classification,” Recreational Mathematics Magazine 12:20 [June 2025], 19-40.)
“In Memoriam: Dizzy Gillespie and Rudolf Nureyev”
So. Farewell
Then
Dizzy Gillespie
Famous jazz
Trumpeter.
You were known
For your
Bulging cheeks.
Rudolf Nureyev
So were
You.
— E.J. Thribb
The Positivist Calendar
In 1849, to serve as “an introduction to the abstract worship of Humanity,” Auguste Comte proposed a new calendar with 13 months of 28 days. A festival day commemorating the dead brought the total to 365 days, but the extra day fell outside the regular cycle of days of the week, so the first of each month always fell on a Monday. Months were named after great figures in the history of Western Europe:
- Moses
- Homer
- Aristotle
- Archimedes
- Caesar
- Saint Paul
- Charlemagne
- Dante
- Gutenberg
- Shakespeare
- Descartes
- Frederick
- Bichat
To keep things on track, leap years added a second festival day, commemorating holy women. The calendar “contains the names of 558 great men of all periods, classified according to their field of activity,” and villains of history, notably Napoleon, were held up to “perpetual execration.”
The scheme has a pleasing mathematical tidiness: Each year contains exactly 52 weeks falling into 13 months, and each month has exactly 28 days comprising four weeks. The whole thing remains consistent from year to year — if you were born on a Wednesday, your birthday would always fall on a Wednesday. And since all months contain the same number of business days and weekends, statistical comparisons by month are more accurate.
It never caught on, in part because of those month names. Science writer Duncan Steel notes that “it would seem strange to give the date as the third day of Homer, and with a month named for the bard a reference to ‘Shakespeare’s Twelfth Night’ would be ambiguous.”
The Three Utilities Problem
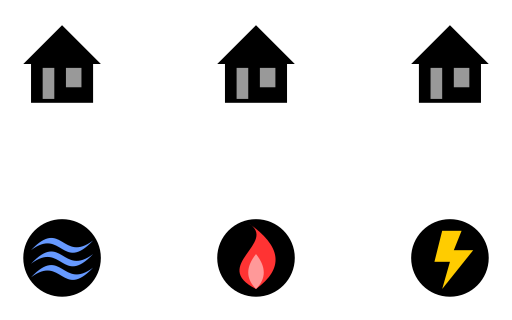
Each of three houses must receive water, gas, and electricity. Is it possible to arrange the connections so that no lines cross?
No, it ain’t. Remove one house and draw connections to the other two:
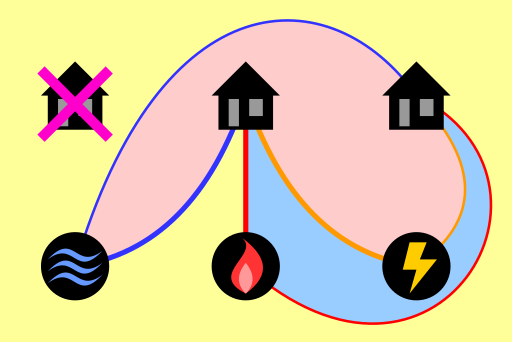
This divides the plane into three regions, here colored red, yellow, and blue. Placing the third house into any of these regions denies it access to the correspondingly colored utility. So the task is impossible.
Pleasingly, the task can be accomplished on a Möbius strip:

And a torus can accommodate up to four houses and four utilities:
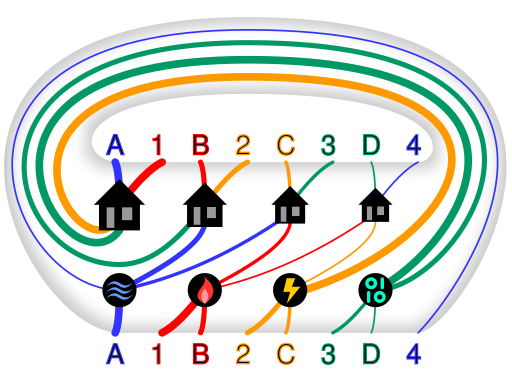
(By Wikimedia user CMG Lee.)
08/31/2025 UPDATE: Reader Guy Bolton King points out that Mathsgear sells a mug embossed with the puzzle. The joke here is that this makes the puzzle solvable — like the torus, the mug is of topological genus 1, “a blob with 1 hole in it,” so it admits the same solution.
And reader Shane Speck writes, “My sneaky solution … has always hinged on the fact that in reality, houses don’t all have separate pipes, and popping on a shared water pipe instantly reduces the problem to the status of incredibly trivial”:
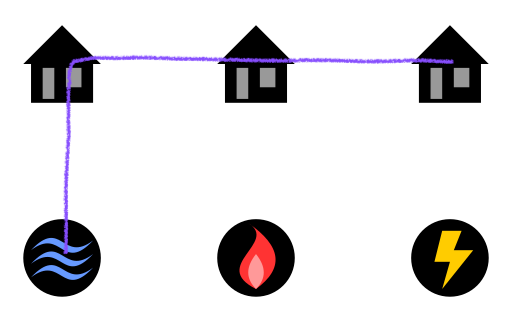
“The problem doesn’t, after all, say you can’t do that… :)”
(Thanks, Guy and Shane.)
Quickie
What’s the final digit in the product of all the odd numbers from 1 to 99?