Imagine a game of tic-tac-toe (noughts and crosses) played in three dimensions in an 8×8×8 cube. A player wins by marking some straight line of eight cells through the large cube. How many such winning lines are there?
Keep Away

A notable adaptation: The forewing tips of the Atlas moth, Attacus atlas, resemble the heads of snakes.
When the moth encounters predators in its native Asian forests, the movement of its wings exaggerates the resemblance.
Insight

“Ideas often flash across our minds more complete than we could make them after much labor.” — La Rochefoucauld
“After investigating a problem in all directions, happy ideas come unexpectedly, without effort, like an inspiration. So far as I am concerned, they have never come to me when my mind was fatigued, or when I was at my working table. … They came particularly readily during the slow ascent of wooded hills on a sunny day.” — Hermann von Helmholtz
“You cannot, with your best deliberation and heed, come so close to any question as your spontaneous glance shall bring you while you rise from your bed or walk abroad in the morning after meditating the matter before sleep on the previous night.” — Emerson
“Three Threes Are Ten”
This little trick often puzzles many:–
Place three matches, coins, or other articles on the table, and by picking each one up and placing it back three times, counting each time to finish with number 10, instead of 9. Pick up the first match and return it to the table saying 1; the same with the second and third, saying 2 and 3; repeat this counting 4; but the fifth match must be held in the hand, saying at the time it is picked up, 5; the other two are also picked up and held in hand, making 6 and 7; the three matches are then returned to the table as 8, 9, and 10. If done quickly few are able to see through it.
— John Scott, The Puzzle King, 1899
04/20/2024 Reader Vladamir Tsepis adds, “This reminds me of the way to convince children you have 11 fingers. Start by showing your left hand splayed, curl down the thumb and index finger counting ‘one, two…’, then of the remaining say ‘let’s skip these three’. Move to your right hand, bend each finger in turn as you count ‘four, five, six, seven, eight…’. Return to the left hand counting off the three we skipped ‘nine, ten, eleven.'”
Fair and Square
From Lee Sallows:
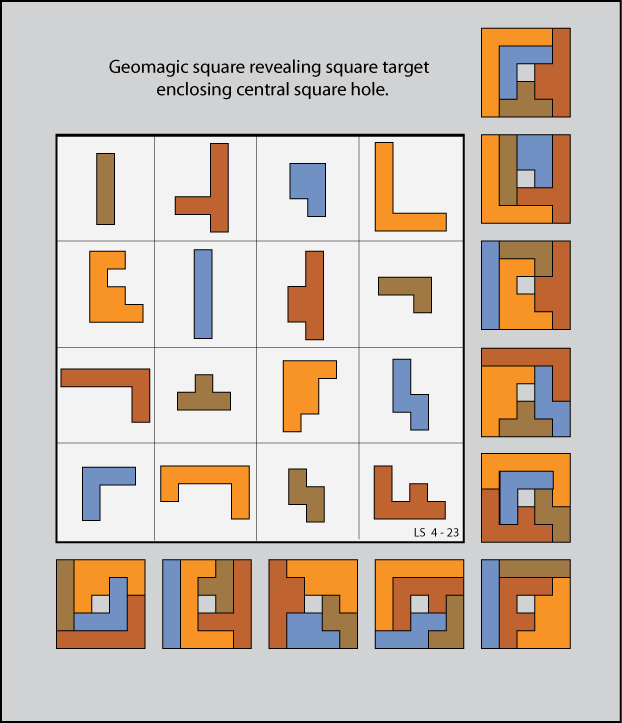
(Thanks, Lee!)
Memorial
From The Book of 500 Curious Puzzles, 1859:
Following is the epitaph of Ellinor Bachellor, an old pie woman. How should we read it?
Bene A. Thin Thed Ustt HEMO. Uld yo
L.D.C. RUSTO! Fnel L.B.
Ach El Lor. Lat. ELY,
Wa. S. shove N. W. How — Ass! kill’d I. N. T. H.
Ear T. Sofp, I, Escu Star.
D. San D T Art. San D K. N E. W. E
Ver — Yus E. — Oft He ove N, W. Hens He
‘Dli V’DL. on geno
Ug H S hem A.D.E. he R. la Stp. Uf — fap
Uf. F. B Y he. R hu
S. Ban D. M.
Uch pra is ‘D. No. Wheres Hedot
HL. i. e. Tom. A kead I.R.T.P. Yein hop Esthathe
R. C. RUSTWI,
L L B. Era is ‘–D!
The Stroke
When John Hollander claimed that English has no rhyme for bilge, George Starbuck imagined advancing on him, calling him a killjoy.
“No don’t!” Hollander cried. “I’m not a killj–“
“Canopus”

When quacks with pills political would dope us,
When politics absorbs the livelong day,
I like to think about that star Canopus,
So far, so far away.
Greatest of visioned suns, they say who list ’em;
To weigh it science always must despair.
Its shell would hold our whole dinged solar system,
Nor even know ’twas there.
When temporary chairmen utter speeches,
And frenzied henchmen howl their battle hymns,
My thoughts float out across the cosmic reaches
To where Canopus swims.
When men are calling names and making faces,
And all the world’s ajangle and ajar,
I meditate on interstellar spaces
And smoke a mild seegar.
For after one has had about a week of
The argument of friends as well as foes,
A star that has no parallax to speak of
Conduces to repose.
— Bert Leston Taylor
In a Word

gliff
n. an unexpected view of something that startles one; a sudden fear
irrision
n. the act of sneering or laughing derisively; mockery; derision
mortiferous
adj. bringing or producing death
proceleusmatic
adj. inciting, animating, or inspiring
Photographer Philippe Halsman took three hours to pose seven women in the shape of a skull for his surrealistic portrait In Voluptas Mors, after a sketch by Salvador Dalí, who’s seen in the foreground. Director Jonathan Demme borrowed the idea for the one-sheet poster for his 1991 film The Silence of the Lambs — the skull image on the “death’s head moth” is a miniature version of the same tableau.
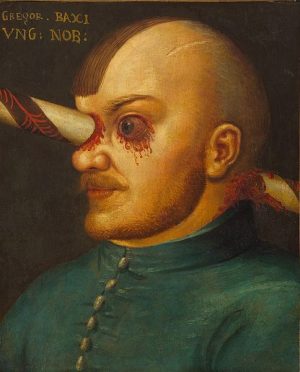
A Remarkable Injury
This gruesome portrait illustrates an unconfirmed story: It’s said that in the 16th century a Hungarian nobleman named Gregor Baci survived for a year after being impaled through the head during a joust.
Did this really happen? We don’t know, but in 2010 surgeon Martin Missmann and his colleagues showed that it could have.
They had been presented with a craftsman whose head was transfixed by a metal bar that had fallen from a height of 14 meters. After two surgeries and a year of headaches and double vision, they said, the patient was symptom-free.
“This case shows that even severe penetrating traumas of the head and neck can be survived without sequelae of serious physiological dysfunction,” they wrote.