Epitaph of the eminent barrister Sir John Strange:
Here lies an honest lawyer,–
that is Strange.
From Epitaphiana: or, The Curiosities of Churchyard Literature, 1873.
Epitaph of the eminent barrister Sir John Strange:
Here lies an honest lawyer,–
that is Strange.
From Epitaphiana: or, The Curiosities of Churchyard Literature, 1873.

In December 1924, a postal inspector from Corinth, Miss., leveled a series of charges against the postmaster at the University of Mississippi. “You mistreat mail of all classes,” he wrote, “including registered mail; … you have thrown mail with return postage guaranteed and all other classes into the garbage can by the side entrance,” and “some patrons have gone to this garbage can to get their magazines.”
The slothful postmaster was William Faulkner. He had accepted the position in 1921 while trying to establish himself as a writer, but he spent most of his time in the back of the office, as far as possible from the service windows, in what he called the “reading room.” When he wasn’t reading or writing there he was playing bridge with friends; he would rise grumpily only when a patron rapped on the glass with a coin.
It was a brief career. Shortly after the inspector’s complaint, Faulkner wrote to the postmaster general: “As long as I live under the capitalistic system, I expect to have my life influenced by the demands of moneyed people. But I will be damned if I propose to be at the beck and call of every itinerant scoundrel who has two cents to invest in a postage stamp. This, sir, is my resignation.”
Ding Dong, Texas, is in Bell County.
Houston, Missouri, is in Texas County.
Java, Alabama, is in Coffee County.
Like Nabokov, Lord Dunsany was fond of composing chess problems. This example was published in Hubert Phillips’ Week-end Problems Book of 1932:
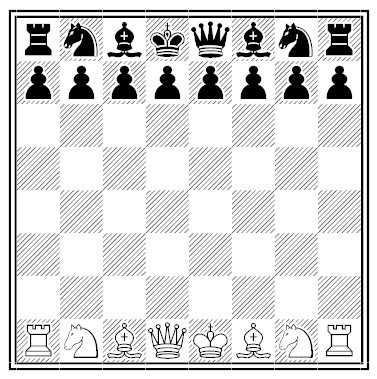
Two eccentric gentlemen abandoned this position at a chess club — White had announced mate in four. What was the mate?
The key is to notice that Black’s king and queen have changed positions. This is not possible if the black pawns are on their home squares. And this means that we’ve been viewing the position upside down:

Now it’s clear that Black is hemmed in by his own pawns — White can mate him in four moves with the b8 knight, e.g. by 1. Nc6 Nf3 2. Nb4 (threatening Nd3#) Ne5 3. Qxe5 (any) 4. Nd3#.
Dunsany drew a game against Capablanca in a simultaneous exhibition in London in April 1929. He later wrote:
One art they say is of no use;
The mellow evenings spent at chess,
The thrill, the triumph, and the truce
To every care, are valueless.
And yet, if all whose hopes were set
On harming man played chess instead,
We should have cities standing yet
Which now are dust upon the dead.
“I see men ordinarily more eager to discover a reason for things than to find out whether the things are so.” — Montaigne
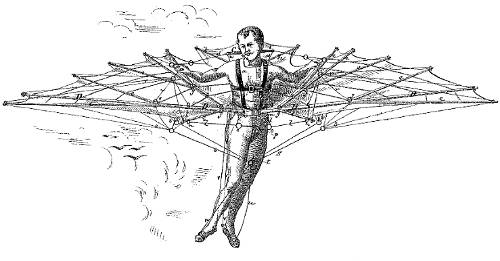
“What can you conceive more silly and extravagant than to suppose a man racking his brains, and studying night and day how to fly?” So wrote the English cleric William Law in 1728, and in the late 19th century inventors were still struggling to reach the heavens.
Watson Quinby’s 1872 “flying apparatus,” above, was designed to permit the user to grope through the air with actions “resembling those of swimming in water.”
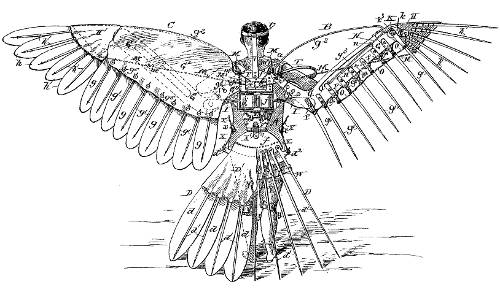
In 1889, Jasper Spalding was emulating a bird rather than a bat with a feathered flying machine designed to be suspended from a balloon (below).
Neither got off the ground … but within one lifetime we had reached the moon.
Unlikely names of actual court cases, compiled by Rodney Jones and Gerald Uelmen in Supreme Folly (1990):
In a certain kingdom, boys and girls are born in strictly equal proportions. Determined to increase the proportion of women in the land, the sultan issues a decree: Any woman who bears a son is forbidden to have any further children. He reasons that some families will thus contain multiple daughters but a single son.
A number of years pass, and the sultan is confused to find that the kingdom still contains an equal number of boys and girls. Why?
expergefaction
n. waking up
matutolypea
n. ill humor in the morning; “getting up on the wrong side of the bed”
“An Extraordinary Sleeper at Newcastle”
In the year 1752, during the summer, the following particulars happened at Newcastle, in Staffordshire, related by a lady of discernment and veracity, who went to see the sleeper several times. She was a girl about 19 years of age; she slept 14 weeks, without waking, although several methods were tried to wake her, as bleeding, blistering, &c.; in all which time she took no sustenance, except about nine o’clock every night, she opened her mouth, and then some person that attended her, dipped a feather in wine, and with that wetted the inside of her mouth. Her father often gave her an airing in a horse chair, and sometimes took her several miles, to have the advice of the physicians; but neither the motion of travelling, nor any thing the physicians could do, could awake her; she appeared to be healthy all the time, breathed freely, and her pulse beat very regularly, but rather too slow; she never moved herself all the time, except once, it is thought, she moved one leg. When she awaked, it was very gradually, being two or three days from the time she began to stir and open her eyes, before she was quite awake, and then seemed to be very well, but complained of faintness. I heard, last summer, that she had good health, and had no return of her sleepiness.
— Gentleman’s Magazine, 1753, quoted in Kirby’s Wonderful and Eccentric Museum, 1820
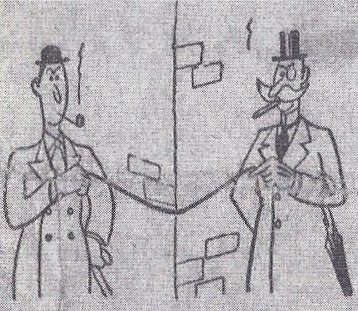
Posing as a surveyor, English prankster Horace de Vere Cole asked a passerby to hold one end of a length of string while he made a measurement. He chose the “pompous sort of good citizen of the bowler hat and rolled umbrella sort,” according to his friend David Scott-Moncrieff. Then he walked around a corner and give the other end to “another consequential ass.”
“Both victims held their ends for fully ten minutes, each invisible to the other, while the perpetrator of the joke quietly slipped away and joined me in a pub commanding a full view of the fun,” Scott-Moncrieff wrote later. “It succeeded far better than I had dared to hope, due to his brilliant selection of two absolutely perfect victims. Each blamed the other, and they nearly came to blows.”
On his honeymoon in 1919, Cole deposited neat piles of horse manure on Venice’s Piazza San Marco … which was devoid of horses. More of his pranks.