English history 1066-1154 as depicted by Mark Twain:
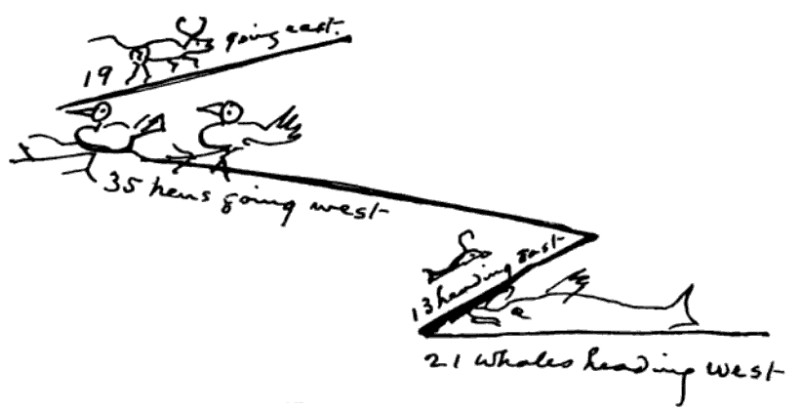
He had discovered that taking notes using pictures helped to fix details in his memory, and in an 1899 essay he recommended the practice to children. An explanation of the diagram, starting at the bottom:
21 whales heading west: These represent William I, whose reign lasted 21 years (1066-1087). “We choose the whale for several reasons: its name and William’s begin with the same letter; it is the biggest fish that swims, and William is the most conspicuous figure in English history in the way of a landmark; finally, a whale is about the easiest thing to draw.”
13 whales heading east: William II, 1087-1100. The change in direction marks a change in leaders. “Make him spout his water forward instead of backward; also make him small, and stick a harpoon in him and give him that sick look in the eye. Otherwise you might seem to be continuing the other William, and that would be confusing and a damage.”
35 hens going west: Henry I, 1100-1135. “That is a hen, and suggests Henry by furnishing the first syllable.”
19 steers going east: Stephen of Blois, 1135-1154. “That is a steer. The sound suggests the beginning of Stephen’s name. I choose it for that reason. I can make a better steer than that when I am not excited. But this one will do. It is a good-enough steer for history.”
The essay was published in Harper’s Monthly Magazine in December 1914, four years after Twain’s death.