
This image turned up in the subreddit Confusing Perspectives back in February.
One user wrote, “Someone needs to make this into real sheet music and see how it sounds. I’m curious now.”
Another version:
https://www.youtube.com/watch?v=MT5erpy3kOA

This image turned up in the subreddit Confusing Perspectives back in February.
One user wrote, “Someone needs to make this into real sheet music and see how it sounds. I’m curious now.”
Another version:
https://www.youtube.com/watch?v=MT5erpy3kOA

“I do not remember this day.” — Dorothy Wordsworth, diary, March 17, 1798
“Such a beautiful day, that one felt quite confused how to make the most of it, and accordingly frittered it away.” — Caroline Fox, diary, January 4, 1848
“I shall not remember what happened on this day. It is a blank. At the end of my life I may want it, may long to have it. There was a new moon: that I remember. But who came or what I did — all is lost. It’s just a day missed, a day crossing the line.” — Katherine Mansfield, diary, Jan. 28, 1920
“Wrote nothing.” — Franz Kafka, diary, June 1, 1912
“I awakened feeling dull. The weather is neither cheerful nor depressing. It makes me restless. The trees are tossed by gusty, fantastic wind. The sun is hidden. If I put on my dressing-gown I am too hot, if I take it off I am cold. Leaden day in which I shall accomplish nothing worth while. Tired and apathetic brain! I have been drinking tea in the hope that it would carry this mood to a climax and so put an end to it.” — George Sand, diary, June 1, 1837
Samuel Johnson resolved 14 times “to keep a journal.” The first resolution was in 1760, the last in 1782.

Suppose lightning strikes a dead tree in a swamp; I am standing nearby. My body is reduced to its elements, while entirely by coincidence (and out of different molecules) the tree is turned into my physical replica. My replica, The Swampman, moves exactly as I did; according to its nature it departs the swamp, encounters and seems to recognize my friends, and appears to return their greetings in English. It moves into my house and seems to write articles on radical interpretation. No one can tell the difference.
But there is a difference. My replica can’t recognize my friends; it can’t recognize anything, since it never cognized anything in the first place. It can’t know my friends’ names (though of course it seems to), it can’t remember my house. It can’t mean what I do by the word ‘house’, for example, since the sound ‘house’ it makes was not learned in a context that would give it the right meaning — or any meaning at all. Indeed, I don’t see how my replica can be said to mean anything by the sounds it makes, nor to have any thoughts.
(“I should emphasize that I am not suggesting that an object accidentally or artificially created could not think; The Swampman simply needs time in which to acquire a causal history that would make sense of the claim that he is speaking of, remembering, identifying, or thinking of items in the world.”)
(Donald Davidson, “Knowing One’s Own Mind,” Proceedings and Addresses of the American Philosophical Association 60:3 [January 1987], 441-458.)
A “kinde of Divination” “to tell your friend how many pence or single peeces, reckoning them one with another, he hath in his purse, or should thinke in his minde,” from Robert Recorde’s The Ground of Arts, 1618:
[F]irst bid him double the peeces hee hath in his purse, or the number hee thinketh. … Now after hee hath doubled his number, bid him adde thereunto 5 more, which done, bid him multiply that his number by 5 also: which done bid him tell you the just sum of his last multiplication, which sum the giver thinking it nothing availeable, because it is so great above his pretended imagination: yet thereby shall you presently with the helpe of Subtraction tell his proposed number.

Apparently the section on “divers Sportes and Pastimes, done by Number” was contributed by Southwark schoolmaster John Mellis in 1582. “[T]he fact that this chapter on mathematical games was included in every subsequent edition of The Ground of Artes, save one, indicates that the idea of mathematical games found a receptive audience among arithmetic students.”
(Jessica Marie Otis, “‘Sportes and Pastimes, done by Number’: Mathematical Games in Early Modern England,” in Allison Levy, ed., Playthings in Early Modernity: Party Games, Word Games, Mind Games, 2017.)
Glimmering, gone — springtime stream
Lapping … road winding down
The shimmering hill. Hometown
Napping … sweet, solemn dream!
Dream solemn, sweet … napping
Hometown … hill shimmering … the
Down-winding road … lapping
Stream … springtime … gone, glimmering.
Willard R. Espy quotes this in his 1999 book The Best of an Almanac of Words at Play without citing the source. It’s by David L. Stephens.
The fastest man on earth is named Bolt. Is that a coincidence, or did his name influence his choice of career?
In 2015, four curious researchers combed the British medical register looking for practitioners whose surnames seemed apt for their specialties (e.g., a neurologist named Brain). Then they compared the frequency of apt names listed under hospital specialties against their frequency in the register in general. They found that “[t]he frequency of names relevant to medicine and to subspecialties was much greater than that expected by chance.” Some examples:
General surgery: Gore, Butcher, Boyle, Blunt
Urology: Ball, Burns, Cox, Dick, Waterfall
Psychiatry: Downs, Lowe, Bhatti, Moodie, Nutt
Cardiology: Hart, Pump, Payne
Dermatology: Boyle, Hickey
Neurology: Counsell, Panicker
Paediatric medicine: Boys, Gal, Child, Kinder
Also: “Paediatric medicine was much more likely to be Wong than White (10:2), whereas anaesthetists were far more likely to be White than Wong (22:4).” And “One wonders if the comforting words of Dr Lie carry less impact because of the name, or whether consultations with Dr Dark in oncology are made any more ominous.”
(C. Limb et al., “Nominative Determinism in Hospital Medicine,” Bulletin of the Royal College of Surgeons of England 97:1 [2015], 24-26.) (See Doctor Doctor and the Doctor’s Names List.)
09/03/2022 UPDATE: In August 2022 the American Association for Anatomy named a new editor for the journal Anatomical Sciences Education. He is Jason Organ. (Thanks, Kevin.)

On May 14, 2008, 19-year-old Brandon Swanson of Marshall, Minnesota, called his parents around 2 a.m. to say he’d driven his car into a ditch and asked them to pick him up. He said he wasn’t hurt and gave them his best estimate of his location.
His parents drove to meet him, keeping in touch by phone, but couldn’t find him. Each party flashed its headlights, but neither could see the other.
Finally Brandon told them he was going to walk toward some lights that he took to be the town of Lynd, 7 miles from Marshall. He named a bar there and asked his father to meet him in the parking lot, and his father began to drive there, talking to Brandon as he did.
Shortly after 2:30 a.m., 47 minutes into the call, Brandon suddenly interrupted himself with the words “Oh, shit!”, and the connection was lost. He has not been seen or heard from since.
Cell phone records showed that he’d been near Porter, 25 miles from the location he’d estimated. His car was found nearby, but years of searching have not found a body. The case remains unsolved.
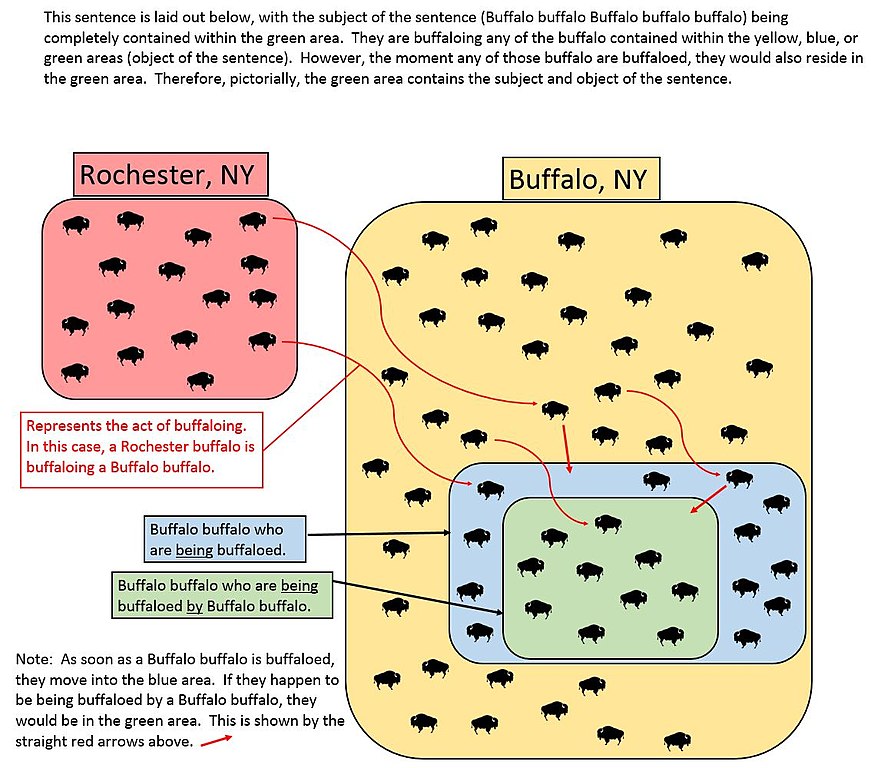
I had written about this back in 2006, but it’s worth mentioning again because someone has created this pellucid diagram: Buffalo buffalo Buffalo buffalo buffalo buffalo Buffalo buffalo is a grammatical English sentence. It means something like “Bison residing in Buffalo, New York, feeling themselves intimidated by their fellows, visit a similar fate upon yet others of their local ilk.”
I’d attributed it to linguist William J. Rapaport, but apparently it’s arisen independently at least three times, first (it is believed) by wordplay maven Dmitri Borgmann, in 1965.
An unpaired word has a prefix or suffix that suggests that an antonym exists when in fact it doesn’t: disheveled is a word, but sheveled isn’t. In many cases the seeming antonym is a real word that’s fallen out of popular usage: corrigible, domitable, effable, feckful, gainly, nocuous, scathed, stinting, trepid, and wieldy are words; they’re just not used as often as their opposites.
Somewhat similarly, a plurale tantum is a noun that appears only its plural form: We speak of scissors and trousers, but not normally of “a scissor” or “a trouser.” A singulare tantum is a noun that’s used only in the singular, such as information, dust, or wealth.
(See “A Very Descript Man.”) (Thanks, Matt.)
A little oddity: In Edith Wharton’s The Age of Innocence, a sentence of 16 words (“The change will do you good, and you must be sure to go and see Ellen,” spoken to Newland Archer by his wife May) has a meaning of 221 words:
It was the only word that passed between them on the subject; but in the code in which they had both been trained it meant: ‘Of course you understand that I know all that people have been saying about Ellen, and heartily sympathise with my family in their effort to get her to return to her husband. I also know that, for some reason you have not chosen to tell me, you have advised her against this course, which all the older men of the family, as well as our grandmother, agree in approving; and that it is owing to your encouragement that Ellen defies us all, and exposes herself to the kind of criticism of which Mr. Sillerton Jackson probably gave you, this evening, the hint that has made you so irritable…. Hints have indeed not been wanting; but since you appear unwilling to take them from others, I offer you this one myself, in the only form in which well-bred people of our kind can communicate unpleasant things to each other: by letting you understand that I know you mean to see Ellen when you are in Washington, and are perhaps going there expressly for that purpose; and that, since you are sure to see her, I wish you to do so with my full and explicit approval — and to take the opportunity of letting her know what the course of conduct you have encouraged her in is likely to lead to.’